Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-26T22:27:04.871Z Has data issue: false hasContentIssue false
  • English
  • Français

Buckling of a Longitudinally Stiffened Flat Panel

Published online by Cambridge University Press:  07 June 2016

H. L. Cox  and
J. R. Riddell
H. L. Cox
Affiliation:
Engineering Division, National Physical Laboratory
J. R. Riddell
Affiliation:
Engineering Division, National Physical Laboratory
Get access

Summary

  • (a)Purpose of Investigation. To determine the least size of stringers necessary to prevent overall buckling of a flat stiffened panel before buckling of the plates between stringers.

  • (b)Range of Investigation. The conditions for buckling of a longitudinally stiffened flat panel are established on the assumption that the rotational stiffness of the stringers is negligible. The results are applied to determine the limiting characteristics of the stringers to ensure that these members remain straight up to the stress at which the plates buckle between stringers. The analysis has been carried through in detail for panels with one, two, or three stringers and is capable of extension to four, five or more stringers. This extension appears unnecessary, because the three stringer case is moderately close to the limiting case of a wide panel with an indefinitely large number of stringers.

  • (c)Conclusions. The stiffening effect of the stringers, having each the area of section A, and modulus of section AsK2, when attached to a sheet of thickness t and length a, at spacing b depends upon the three ratios (k/t), (a/b) and (As/bt) In general, the size of stringer necessary to ensure that the stringers remain straight until the plates buckle between stringers may be represented by the conditions

    .

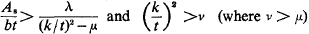    For a panel stiffened by one stringer only suitable values are λ = 4.5 and μ = 12.5, independent of the value of (a/b), while v=0.366 (a/b)2or 23 if 8>(a/b)>6.

    For two or more stringers the same general conditions hold, but it is impossible to assign values to λ and μ which would be fully satisfactory for all values of the ratio a/b. In these cases, therefore, it is more satisfactory to select the values of λ and μ appropriate to each value of the ratio a/b. These values may be obtained from Figs. 2 and 3 where curves of bt/As against (k/t)2for varying values of (a/b) are shown for two and three stringers respectively. For an indefinitely large number of stringers the corresponding condition is

    and the second condition (k/t)2 0.366 (a/b)2 is virtually included in the first.

  • (d)Further Developments. In the present analysis the resistance of the stringers. to rotation is neglected. Inclusion of this characteristic in the basic analysis is straightforward, but practical interpretation of the results becomes much more difficult. Moreover, the effect of this stiffness in modifying the condition for buckling between stringers is likely to affect the conclusions more than the direct effect of their torsional stiffness in modifying the condition for buckling of the stiffened panel. This consideration is particularly important in cases of light stringers and heavy sheet, when the effective rotational stiffness of the stringers at buckling of the plates between stringers may be greatly reduced as a result of the end loading and may even be negative. Representation of the effect of both longitudinal stiffeners (stringers) and lateral stiffeners (ribs), including their stiffnesses to rotation is also straightforward; but interpretation of the results in forms suitable for practical application would be extremely difficult.

Type
Research Article
Information
Aeronautical Quarterly , Volume 1 , Issue 3 , 01 November 1949 , pp. 225 - 244
Copyright
Copyright © Royal Aeronautical Society. 1949

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Timoshenko, S. (1936). Theory of Elastic Stability, McGraw Hill Book Co., p. 371, 1936.Google Scholar
2. Cox, H. L., and Smith, H. E. (1943). The Buckling of Grids of Stringers and Ribs, Proc. Land. Math. Soc, Ser. 2, Vol. 48, p. 1, 1943.Google Scholar
3. Cox, H. L., and Smith, H. E. (1943). Structures of Minimum Weight, R.&M. 1923, 1943.Google Scholar
4. Cox, H. L., and Smith, H. E. (1943). The Buckling of a Thin Sheet Transversely Stiffened, Proc. Lond. Math. Soc, Ser. 2, Vol. 48, p. 27, 1943.Google Scholar
5. Cox, H. L. (1945). The Buckling of a Flat Rectangular Plate under Axial Compression and its Behaviour after Buckling, R.& M. 2041, 1945.Google Scholar
6. Smith, C. B., Ringelstetter, L. A., and Norris, C. B. (1947). Buckling of Stiffened Flat Plywood Plates in Compression: a Single Stiffener Parallel to Stress. U.S. Dept. of Agriculture, Forest Products Lab. Rep. No. 1553-B, 1947Google Scholar