No CrossRef data available.
Published online by Cambridge University Press: 17 February 2009
The paper presents some additional solutions of the diffusion equation
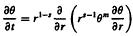
for the case s = 2, m = −1, a case that was left open in a previous discussion. These solutions behave in a manner that is physically acceptable as the time, t, increases and as the radial coordinate, r, tends to infinity.