Published online by Cambridge University Press: 17 February 2009
Our purpose in this paper is to display the stability analysis of Runge–Kutta methods applied to a Volterra integral equation of a simple form. As prerequisite we define, and then develop the structure of, the class of Runge–Kutta methods considered. The test equation is taken as the “;basic” equation 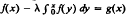 ; the simple form of this equation permits ready insight into features which are more obscure when considering (as elsewhere [1], [2], [6]) equations of a more complicated form. Due to the structure of the methods and the nature of the test equation, the stability analysis reduces to the study of recurrence relations of the form Фk + 1 = MФ k + γk (k = 0, 1, 2, …) which are common in stability discussions in numerical analysis.
; the simple form of this equation permits ready insight into features which are more obscure when considering (as elsewhere [1], [2], [6]) equations of a more complicated form. Due to the structure of the methods and the nature of the test equation, the stability analysis reduces to the study of recurrence relations of the form Фk + 1 = MФ k + γk (k = 0, 1, 2, …) which are common in stability discussions in numerical analysis.