The accurate measurement of body composition is crucial to understanding the impacts of different types of weight-loss interventions on body compartments. Although impressive advances have been made in the techniques available for measuring human body composition, due to cost and limited availability, use in clinical practice has been limited. The most common methods for estimating body composition changes that are available to clinicians include dual-energy X-ray absorptiometry (DXA), bioelectrical impedance analysis (BIA), anthropometry and, the most widely applied, BMI.
At the simplest level, techniques for measuring body composition can assume that the body is divided into two compartments, fat mass (FM) and fat-free mass (FFM). The fat component is of relatively homogeneous composition, but FFM consists of a heterogeneous mix of water, mineral, protein and additional minor constituentsReference Baumgartner, Heymsfield, Lichtman, Wang and Pierson1, Reference Heymsfield and Waki2. In order to quantify the amount of FM and FFM, using a two-compartment model, one must assume that these components exist in a known relationship to each other. For example, hydrodensitometry, or underwater weighing, assumes known densities of FM and FFM. By measuring the mass and volume of a subject, one could estimate the amount of FM and FFM, based on the assumed densities of these compartments. In clinical practice, however, there is considerable inter-individual variability in the density of FM and FFM that can affect the accuracy of this measureReference Baumgartner, Heymsfield, Lichtman, Wang and Pierson1, Reference Heymsfield and Waki2. There are even within-subject differences, particularly in the proportion of water and mineral in FFMReference Heymsfield and Waki2. This variability contributes to the absolute error of this method.
Because of the changes in FFM and total body composition that can occur during a weight-loss programme, multi-compartment models have been developed in which various components of the body are independently measured, thus reducing the error involved with body composition measurementReference Fogelholm and van Marken Lichtenbelt3. An early example of this approach was that of SiriReference Siri and Henschel4 who divided the body into a three-compartment model comprising of fat, water, and a ‘fat-free dry substance’ (protein plus mineral). Moreover, four-compartment models, that also take into account the measurement of body mineral, can further reduce the variability in the density of FFM (DFFM) measurement. One of the primary advantages of the four-compartment model is that it provides a more accurate measure of body composition than do other methods, particularly because it requires fewer assumptions than three- and two-compartment modelsReference Heymsfield, Lohman, Wang and Going5. Despite their apparent advantages, few studies have used four-compartment models to evaluate changes in body composition that result during weight lossReference Fogelholm, Sievanen, van Marken Lichtenbelt and Westerterp6.
Any changes in the assumed constants induce inaccurate FM assessment when two-compartment models are used. This may be the case when measuring body composition in obese patients, as changes in the DFFM were observed due to an increased hydration of FFMReference Leone, Gallagher, Wang and Heymsfield7. Within the scope of weight loss this methodological issue assumes further importance for the use of a reference four-compartment model, because it is plausible that FFM composition may differ from the assumed constants in the two-compartment model and may change from subject to subject.
In addition to the aforementioned applications in a research setting, clinicians might also benefit from the use of four-compartment models in clinical diagnosis and patient care when accurate estimates are desired. However, these estimates are costly and time consuming, highlighting a need to identify alternative methods that are convenient, reliable and accurate for measuring changes in body composition that occur with weight loss. Only three studies have assessed the validity of different body composition measures in overweight and obese populations by making comparisons with a reference four-compartment molecular modelReference Fogelholm, Sievanen, van Marken Lichtenbelt and Westerterp6, Reference Albu, Smolowitz, Lichtman, Heymsfield, Wang, Pierson and Pi-Sunyer8, Reference Evans, Saunders, Spano, Arngrimsson, Lewis and Cureton9. However, none of these studies used a long-term weight-loss programme in women with a wide range of fatness change. Therefore, the aim of the present study was to compare measures from DXA, BIA and anthropometry with a reference four-component molecular model to estimate FM and FFM changes in overweight and obese women after a weight-loss management programme.
Experimental methods
Subjects
Participants were recruited from a community sample for a 2-year weight-management programme through newspaper advertisements, the Internet and announcement flyers. Only females were eligible to participate in the study. The other inclusion criteria were age >24 years, premenopausal and not currently pregnant, BMI>24·9 kg/m2, healthy and not currently taking medications. After one orientation session, 152 women signed up for the programme. During the run-in phase, four women decided not to participate (reporting new time and scheduling conflicts), four did not comply with testing requirements and were excluded, three women found out they were pregnant or decided to attempt pregnancy and were also excluded, and one subject was found ineligible due to medical reasons (untreated hyperthyroidism), leaving a total of 140 women who started the intervention. An initial visit with the study physician ensured that subjects met all medical inclusion criteria. Participants agreed to refrain from participating in any other weight-loss programme and gave written informed consent before participation in the study. The Institutional Review Board of the Faculty of Human Movement, Technical University of Lisbon, approved the study, described elsewhereReference Teixeira, Going, Houtkooper, Cussler, Metcalfe, Blew, Sardinha and Lohman10.
At 1 year after the intervention, ninety-three women were evaluated but only forty-eight women performed all the body composition methods and lost weight.
Body composition measurements
Measurements of body composition using each technique were conducted according to standard procedures. Subjects came to the laboratory, after a 12 h fast, and 24 h without exercise, alcohol or stimulant beverages. All measurements were carried out in the same morning. In brief, the procedures were as follows.
Anthropometry
Subjects were weighed to the nearest 0·01 kg wearing a bathing suit and without shoes on an electronic scale connected to the plethysmograph computer (BOD POD©; Life Measurement, Inc., Concord, CA, USA). Height was measured to the nearest 0·1 cm with a stadiometer (Seca, Hamburg, Germany).
The Weltman et al. Reference Weltman, Levine, Seip and Tran11 formula was used to estimate percentage FM, as this model was developed for obese subjects. The equation is described as follows:
where ABCirc is the mean abdominal circumference ((AB1+AB2)/2; AB1 is the abdominal circumference measured between the appendix xifoid and the umbilical level and AB2 is measured at the umbilical level), Ht is height (cm) and Wt is body weight (kg).
A trained researcher performed the circumference measurements according to the standardised procedures described elsewhereReference Harrison, Buskirk, Lindsay Carter, Johnston, Lohman, Pollock, Roche, Wilmore, Lohman, Roche and Martorell12. Based on ten repetitions, the technical error of measurement (TEM) and intraclass coefficient of correlation were 0·37 cm and 1·00 for AB1 and 0·62 cm and 1·00 for AB2, respectively.
Bioelectrical impedance analysis
BIA from foot to foot was measured using the Tanita body composition analyser (model TBF-310; Tanita Corp., Tokyo, Japan), which provides a print-out of measured impedance and calculated body fat. Subjects were measured without shoes, and in bathing suits. BIA from hand to hand was measured using the Omron BF300 (Omron Corp., Kyoto, Japan), which provides absolute and percentage body fat.
Dual-energy X-ray absorptiometry
To estimate FM and FFM, DXA measurements were made with a total body scanner (QDR-1500, pencil-beam mode, software version 5.67 enhanced whole-body analyses; Hologic, Waltham, MA, USA) that measured the attenuation of X-rays pulsed between 70 and 140 kV synchronously with the line frequency for each pixel of the scanned image. Following the protocol for DXA described by the manufacturer, a step phantom with six fields of acrylic and aluminium of varying thickness and known absorptive properties was scanned alongside each subject to serve as an external standard for the analysis of different tissue components. The same laboratory technician positioned the subjects, performed the scans and executed the analysis according to the operator's manual using the standard analysis protocol. Based on ten subjects, the CV in our laboratory for FM and FFM were 2·9 and 1·7 %, respectively.
Four-compartment model
The four-compartment model developed by Heymsfield et al. Reference Heymsfield, Lohman, Wang and Going5 was used as the reference method. Accordingly, FM was assessed with the following equation:
where BV is body volume (litres), TBW is total body water (kg), Mo is bone mineral (kg) and BW is body weight (kg).
Calculation of density of fat-free mass
The DFFM was estimated from TBW, bone mineral (Mo) and residual (residual is equal to body mass minus FM from the four-compartment model, TBW and bone mineral) contents of FFM (estimated as body mass minus FM from the four-compartment model) and densities of TBW (DTBW), bone mineral (DMo) and residual (Dresidual); 0·99 371, 2·982 and 1·404 g/cm3, respectively:
Body volume
BV was assessed by air-displacement plethysmograph (BOD POD®; Life Measurement, Inc., Concord, CA, USA). The use of this method is described in detail elsewhereReference McCrory, Gomez, Bernauer and Mole13, Reference Dempster and Aitkens14. Briefly, after voiding the bladder, each subject was weighed to the nearest g while wearing a swimsuit. The BOD POD® was calibrated according to the manufacturer's instructions, and raw BV was determined. The effects of clothing and hair are accounted for by using minimal clothing, such as a bathing suit, and by compressing hair with a swimming cap. Finally, thoracic gas volume was measured in the BOD POD® by using a technique, common to standard pulmonary plethysmography, called the ‘panting manoeuvre’Reference Dubois, Botelho, Bedell, Marshall and Comroe15. While wearing a noseclip, the subjects breathed through a tube; after two to three normal breaths, the airway was occluded for 3 s at mid-exhalation. During this time, the subject was instructed to gently puff against the occlusion by alternately contracting and relaxing the diaphragm.
All measurements were conducted with the BOD POD® software version 1.68 (Life Measurement, Inc.).
Based on ten repetitions, TEM and the CV for BV were 0·2 litres and 0·5 %, respectively.
Total body water
An accurate method to estimate TBW is by bioelectrical impedance spectroscopy analysis (model 4000B; Xitron Technologies, San Diego, CA, USA). Before the test, subjects were instructed to lie in a supine position with their arms and legs abducted at a 45° angle for 10 min. This impedance spectra was modelled with the Cole–Cole cell suspension modelReference Cole and Cole16 to derive a theoretical impedance at zero and infinity frequency, based on a non-linear curve fitting from the measured resistance and reactance. Intracellular water and extracellular water were predicted from the Hanai mixture theoryReference Hanai and Sherman17, and TBW was estimated by the sum of intracellular water and extracellular water. Considering ten repeated measures, the TEM and CV for TBW were 0·47 litres and 1·1 % respectively.
Bone mineral
Total body bone mineral was measured using DXA (QDR-1500, pencil-beam mode, software version 5.67 enhanced whole-body analyses; Hologic, Waltham, MA, USA) as described earlier for FM and FFM. Considering that bone mineral content represents ashed bone, bone mineral content was converted to total body bone mineral by multiplying it by 1·0436 (Ballor 1996Reference Ballor and Lohman18). The TEM and CV for bone mineral content in our laboratory were 0·02 kg and 1·6 %, respectively.
Propagation measurement error
In the present study we selected air-displacement plethysmography to assess BV, DXA to estimate bone mineral, and BIA to estimate TBW. The propagation of measurement errors associated with the determination of BV, TBW and bone mineral (Mo) can be calculated by assuming that the squared technical errors of measurement (TEM2) are independent and additiveReference Ballor and Lohman18. Accordingly:

So, using the above equation:
From these calculations, the test–retest reliability in the present study was about 0·8 % FM units.
Statistical analysis
Data were analysed with SPSS for Windows version 13.0 (SPSS Inc., Chicago, IL, USA). Descriptive statistics including mean values and standard deviations were calculated for all outcome measurements (age, weight, height, BV, FM, FFM, TBW, bone mineral, protein, density).
Simple linear regressions were performed to calculate the relationship between FM, percentage FM, and FFM estimated by the reference four-compartment model and DXA, BIA using Tanita TBF-310 (BIA-Tanita), BIA using Omron BF300 (BIA-BF300) and anthropometrics. Bias between methods was assessed with paired t tests. Where differences were found between the test measures and the four-compartment model, linear regression models were developed to determine if the observed differences were explained by variation of DFFM and FFM hydration. Agreement between methods was assessedReference Bland and Altman19, including the 95 % limits of agreement. The correlation between the mean of the reference method and the assessed method with difference between both was used as an indication of trend, i.e. the difference between the methods varied across fatness levels. Linear regressions were also performed to assess the relationships between initial body weight and the differences between FM, percentage FM and FFM changes estimated by the reference four-compartment model and DXA, BIA-Tanita, BIA-BF300 and anthropometrics. For all tests, statistical significance was set at P < 0·05.
Results
Subjects' characteristics
Baseline and after intervention
Mean values and standard deviations for the descriptive characteristics are summarised in Table 1. It is worth mentioning that after the intervention, DFFM was identical to the reference man (1·1 g/cm3), even though all compartments of the FFM deviate from the reference man. There were no significant differences in bone mineral:FFM at baseline and after intervention (0·1 (sd 0·2) %; P = 0·096). All the other variables were significantly different (P < 0·05) after intervention.
Table 1 Subject (n 48) characteristics and body composition (multi-compartment model)
(Mean values and standard deviations)
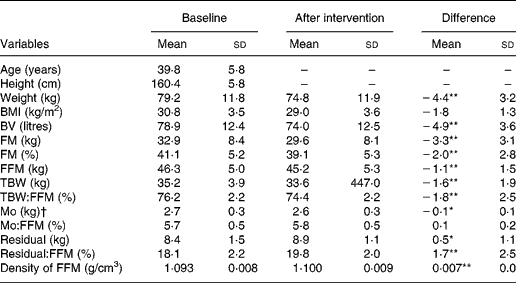
BV, body volume; FM, fat mass; FFM, fat-free mass; TBW, total body water; Mo, bone mineral (mineral osseous).
* Mean values at before weight loss and after weight loss were significantly different: *P ≤ 0·05, **P ≤ 0·001.
† Mo = BMC × 1·0436, where BMC is bone mineral content from dual-energy X-ray absorptiometry.
The mean values and standard deviations at baseline, after intervention, and respective differences in FM, percentage FM and FFM by the indicated methods are summarised in Table 2. When compared with the reference four-compartment model, FM, percentage FM, and FFM assessed by anthropometry (waist circumference-based model; Antrform), BIA-Tanita, BIA-BF300 and DXA were significantly different at baseline and after intervention (P ≤ 0·001), with the exception for FFM assessed by BIA-Tanita (baseline, P = 0·071 and after, P = 0·007).
Table 2 Body composition parameters in the sample (n 48) at baseline, after intervention and respective differences
(Mean values and standard deviations)

FM, fat mass; Antrform, anthropometry (waist circumference-based model); BIA-Tanita, bioelectrical impedance analysis using Tanita TBF-310; BIA-BF300, bioelectrical impedance analysis using Omron BF300; DXA, dual-energy X-ray absorptiometry; 4-C, four-compartment model; FFM, fat-free mass.
Mean values at baseline and after intervention were significantly different: *P ≤ 0·05, **P ≤ 0·001.
Mean change was significantly different from that observed using the reference method (4-C): †P < 0·05, ††P < 0·001.
Changes
All body composition methods presented a significant (P < 0·05) decrease in FM and percentage FM after intervention. The largest decrease was obtained by DXA, which overestimated FM change ( − 4·5 v. − 3·3 kg; P < 0·001 and − 3·7 v. − 2·0 %; P < 0·001) when compared with the four-compartment model. Conversely, the Antrform method underestimated FM change ( − 2·8 v. − 3·3 kg; P = 0·043 and − 1·1 v. − 2·0 %; P = 0·013). BIA-Tanita and BIA-BF300 did not differ (P < 0·05) from the reference multi-compartment model in any body composition variable.
Accuracy of body composition changes
The accuracy data of the Antroform, BIA-Tanita, BIA-BF300 and DXA methods to estimate FM, percentage FM and FFM changes are presented in Table 3.
Table 3 Performance criteria: slope, intercept, coefficient of correlation, standard error of estimation and the agreement (bias, limits and trend) between fat mass (FM), percentage FM and fat-free mass (FFM) changes using the four-compartment model as the reference (n 48)

Antrform, anthropometry (waist circumference-based model); BIA-Tanita, bioelectrical impedance analysis using Tanita TBF-310; BIA-BF300, bioelectrical impedance analysis using Omron BF300; DXA, dual-energy X-ray absorptiometry.
Trend was significant: *P ≤ 0·05, **P ≤ 0·001.
† Significantly different from 0.
‡ Significantly different from 1.
For FM and percentage FM all intercepts were not different from zero (P < 0·05). A similar result was found for FFM using the Antrform method. For FM, only slopes calculated from the BIA methods were not different from 1 (P>0·05), while for percentage FM only BIA-BF300 was not different from 1 (P < 0·05). All slopes for FFM were different from 1 (P < 0·05).
Agreement analysis
The agreement between the four-compartment model and the other methods is also indicated in Table 3. A significant bias was found between the four-compartment model and Antrform and DXA in all body composition variables. The 95 % limits of agreement ranged from − 3·4 to 4·5 for FM, from − 5·7 to 5·4 for percentage FM, and from − 3·8 to 4·4 for FFM. For FM and percentage FM, a significant trend was found for the Antrform method (P < 0·001), BIA-BF300 (P = 0·030) and DXA (P = 0·009), i.e. there was a correlation between the mean of each of these methods with the four-compartment model and the difference between both. Bland–Altman plots of absolute FM and FFM changes are shown in Fig. 1.

Fig. 1 Bland–Altman analysis for the change (difference between before and after weight loss) using the different methods. The middle solid line represents the mean difference between absolute fat mass (FM) (A, C, E, G) or fat-free mass (FFM) (B, D, F, H) from each method minus absolute FM or FFM from the four-component model (4-C); the upper and lower dashed lines represent ± 2 sd from the mean, i.e. 95 % limits of agreement ( ± 1·96 sd). (A, B) Anthropometric formula (Antrform; waist circumference-based model); (C, D) dual-energy X-ray absorptiometry (DXA); (E, F) bioelectrical impedance analysis using Tanita TBF-310 (Tanita); (G, H) bioelectrical impedance analysis using Omron BF300 (BF300).
Fat-free mass hydration and density
The effect of FFM hydration and density on body composition differences was investigated to determine if it could explain some of the disparities between body composition measures and the four-compartment model. The body composition changes were only significantly associated with the differences obtained between body composition variables from the four-compartment model and DXA (hydration, FM: β = − 19·3, P = 0·039; FFM: β = 17·0, P = 0·035; density, FM: β = 59·1, P = 0·021; FFM: β = − 54·6, P = 0·013). All the other differences using methods besides DXA were not associated with FFM hydration or density.
Relationship between body-weight changes and differences between methods
The relationship between the differences in FM using each method and the reference method and body-weight change is illustrated in Fig. 2. A positive correlation was found between body-weight changes and the difference between the reference four-compartment model and Antrform and DXA (r 0·418, P < 0·05 and r 0·386, P < 0·05, respectively). Thus, the more weight subjects lost, the greater the disparity in FM measures from Antrform and DXA and FM measures from the reference method. The difference between the methods explained 17·5 % FM and 14·9 % FM respectively, of total body-weight changes during the intervention. The magnitude of body-weight change did not explain the differences between FM measured from BIA-Tanita and BIA-BF300 and FM from the reference model (P>0·05).

Fig. 2 Relationship between body-weight changes and the differences between methods. (A) Relationship between the difference in fat mass (FM) using anthropometry (waist circumference-based model; Antrform) and the reference method (four-compartment model; 4-C) and body-weight change; (B) relationship between the difference in FM using Tanita TBF-310 (bioelectrical impedance analysis; Tanita) and the reference method and body-weight change; (C) relationship between the difference in FM using Omron BF300 (bioelectrical impedance analysis; BF300) and the reference method and body-weight change; (D) relationship between the difference in FM using dual-energy X-ray absorptiometry (DXA) and the reference method and body-weight change.
Discussion
The primary goal of the present study was to examine the validity of clinical body composition assessment methods for measuring changes in body composition across a 16-month weight-loss intervention using a molecular four-compartment model as reference. Four-compartment models provide more accurate estimates of body composition than the other methods used to estimate body compositionReference Lohman20, thus the present study is unique in its approach. To our knowledge, only three studies have validated measures of body composition across weight loss by comparing them with the reference four-compartment modelReference Fogelholm, Sievanen, van Marken Lichtenbelt and Westerterp6, Reference Albu, Smolowitz, Lichtman, Heymsfield, Wang, Pierson and Pi-Sunyer8, Reference Evans, Saunders, Spano, Arngrimsson, Lewis and Cureton9, but the present study extends these reports by including additional measures of comparison. The present findings reveal that the mean bias in estimating FM and percentage FM changes by DXA and Antrform were not similar when compared with the reference method. As in the cross-sectional data analysis, DXA overestimated FM and percentage FM change (1·3 kg, P < 0·05 and 1·7 %, P < 0·05) while Antrform underestimated FM and percentage FM changes ( − 0·5 kg, P < 0·05 and − 0·9 %, P < 0·05). The other two single-frequency BIA devices, BIA-Tanita and BIA-BF300, similarly estimated the FM and percentage FM changes when compared with the four-compartment model.
These results extend the findings of Evans et al. Reference Evans, Saunders, Spano, Arngrimsson, Lewis and Cureton9 that found no bias in percentage FM changes in the diet only and diet plus exercise weight-loss groups using BIA. Conversely, Fogelholm et al. Reference Fogelholm, Sievanen, van Marken Lichtenbelt and Westerterp6 found that BIA underestimated the change in FM when compared with the four-compartment model. However, in the present short-term 12-week weight-loss study, it is worth noting that, contrary to expected, FFM hydration increased from 72·9 % from baseline to 75·7 % after the intervention, which may explain why changes in FM and percentage FM were underestimated when compared with the reference model. Besides this methodological issue, the authors speculate that TBW may have not been detected accurately by BIA due to the expansion of the intracellular water associated with the refeeding weight-loss-stabilisation period, because the BIA method is essentially dependent on the extracellular pool.
In addition to differences in measuring the change in body composition, baseline measures of FM and percentage FM by Antrform, BIA-Tanita, BIA-BF300 and DXA were not similar to the reference model. Antrform and DXA overestimated FM and percentage FM while BIA-Tanita and BIA-BF300 underestimated FM and percentage FM. Similar results were found after the intervention. Before weight loss, FFM hydration in the present study was 76·2 %, demonstrating an overhydrated state as was expected in overweight and obese individualsReference Leone, Gallagher, Wang and Heymsfield7. After weight loss, FFM hydration changed to 74·4 %. It is important to note that before weight loss FFM hydration was significantly higher than the assumed hydration status (73·8 %) based on chemical cadaver analysisReference Brozek, Grande, Anderson and Keys21. These results indicate that women in the present study may have been overly hydrated, which would result in an overestimation of FFM before weight loss by using BIA-based methods. Indeed, the present results extend the results found by Frisard et al. Reference Frisard, Greenway and Delany22 and Carella et al. Reference Carella, Rodgers, Anderson and Gossain23 and are in agreement with the knowledge that there is large variation in the water content of FFMReference Hewitt, Going, Williams and Lohman24, and that these differences can lead to an underestimation of FFM with BIA in the dehydrated state and an overestimation in the overhydrated stateReference de Fijter, de Fijter, Oe, Donker and de Vries25. These findings may explain the significant increase in the DFFM after weight loss. No differences were found between DFFM observed and the assumed DFFM (1·1 g/cm3) after weight loss, while the baseline values were significantly lower.
As extensively documented, the pencil-beam DXA technology used in the present study (Hologic QDR-1500W with enhanced software version 5.71) tends to overestimate FMReference Evans, Saunders, Spano, Arngrimsson, Lewis and Cureton9, Reference Lohman, Harris, Teixeira and Weiss26. Williams et al. Reference Williams, Wells, Wilson, Haroun, Lucas and Fewtrell27, using a fan-beam technology, also suggested an average higher error in obese subjects. We also found this trend. As compared with the four-compartment model, at baseline when subjects were fatter, DXA overestimated FM by 3·9 kg, while after weight loss this difference decreased to 2·7 kg. It has been suggested that ‘beam hardening’ due to increased body tissue thickness may explain these differencesReference Blake, McKeeney, Chhaya, Ryan and Fogelman28. New fan-beam technology and algorithms have already improved DXA accuracy to estimate FMReference Tylavsky, Fuerst, Nevitt, Dockrell, Wan, Cauley and Harris29, Reference Visser, Fuerst, Lang, Salamone and Harris30. The highest correlation coefficient between body composition changes was found for DXA.
The slopes and intercepts for FM and percentage FM changes with the two BIA methods were not different from 1 and 0, respectively, when the four-compartment model was used as the reference. This would indicate a good accuracy for BIA-Tanita and BIA-BF300. However, considering the data from the agreement analysis, both methods had a wide range for the 95 % CI, indicating a reduced individual accuracy. Further, a significant trend, i.e. a significant correlation between the mean changes observed between BF300 and the four-compartment model and the difference between both methods was found. In other words, the fact that there were not differences between mean FM and percentage FM changes estimated by the four-compartment model and BIA-BF300 is most likely because BIA-BF300 overestimated in subjects that lost less FM and underestimated in subjects that lost more FM. This result underscores the notion that BIA-BF300 is not sensitive to track accurately individuals across large changes in body composition.
Results using the Antrform method are in line with the recognition that it is very difficult for anthropometric methods to accurately estimate body composition changesReference Bellisari, Roche, Heymsfield, Lohman, Wang and Going31. Besides the significant mean bias found for FM and percentage FM changes with DXA, this method also presented a significant trend in the opposite direction. DXA overestimated FM loss in subjects that lost more FM. This finding agrees with previous findings that DXA is less accurate in subjects with larger FMReference Williams, Wells, Wilson, Haroun, Lucas and Fewtrell27. As depicted in Fig. 1, this was further emphasised by the finding that body-weight changes explained 17·5 % of the difference between the methods. Comparable but opposite results were found with Antrform. Differences between FM changes with the BIA devices and the four-compartment model were not associated with the body-weight changes. Thus, the estimated changes are not dependent of the amount of body weight loss.
The effects of the FFM hydration and DFFM on body composition differences observed using the several methods and the four-compartment model were also investigated. The findings showed that there were no associations between changes in FFM hydration and the disparities between the test body composition measures and the four-compartment model, with the exception of DXA (hydration: β = − 19·26, P = 0·039; density: β = 59·05, P = 0·021). Our findings are consistent with those obtained by KohrtReference Kohrt32 that found no influence of FFM hydration changes on assessing small changes of body composition.
Early work showed that the high precision of DXA for estimation of body composition components provided the technology to detect very small changes in body compositionReference Heymsfield, Wang, Lichtman, Kamen, Kehayias and Pierson33. Studies in haemodialysis patients and healthy adults have shown that DXA accurately assessed acute changes in soft tissueReference Stenver, Gotfredsen, Hilsted and Nielsen34, Reference Formica, Atkinson, Nyulasi, McKay, Heale and Seeman35. DXA also appears to be a suitable method for assessing body composition changes in longitudinal studiesReference Frisard, Greenway and Delany22, Reference Svendson36. It is important to note that the conclusions regarding DXA's ability to correctly assess FM and percentage FM may not be applicable to the equipment used in the present investigation, as known significant differences exist between manufacturers and softwareReference Kohrt32, Reference Van Loan, Keim, Berg and Mayclin37.
Although the above findings are based on the assumption that differences between clinical estimates of FM and percentage FM from the reference four-compartment model resulted from error in the alternative method estimation, absolute FM from the reference model is not without error. It has been suggested that the increased error associated with the greater number of measurements contributing to FM estimation with the four-compartment model may negate its greater theoretical accuracyReference Fuller, Jebb, Laskey, Coward and Elia38. The present investigation and the results from Friedl et al. Reference Friedl, DeLuca, Marchitelli and Vogel39 found, however, that the within-subject sd for replicate measurements FM is low ( < 0·8 % of body weight) and similar to that derived with other indirect methods based on fewer measurements, indicating that propagation of measurement error is not a significant problem. For example, the se of estimation for predicting changes in percentage FM from the reference method from changes in percentage FM from DXA, BIA-Tanita, Antrform and BIA-BF300 were 1·7, 1·9, 2·1 and 2·2 % of body weight, respectively, for an average se of estimation of about 2·0 % of body weight for the clinical methods. With the use of the law of propagation of errors and assuming that the criterion measure is not error free (total error of estimation for percentage FM of the four-compartment model: 0·8 % of body weight), the proportion of the se of estimation attributable to the clinical method is reduced to 1·8 % of body weight. To detect a true change in percentage FM from a weight loss in 95 % of individuals, percentage FM would have to change by ≥ 3·2 %. Because the propagation of measurement error is nearly 0·8 % and the mean percentage FM change obtained by the reference method is 2·0 %, the present study agrees with other authors that highlight the difficulty in accurately assessing small changes in body composition with clinical methodsReference Evans, Saunders, Spano, Arngrimsson, Lewis and Cureton9, Reference Lohman40.
When interpreting the results from the present study the following limitations need consideration. First, our sample did not include severely obese women and the sample on average did not lose much weight. Second, the present study used bioelectrical impedance spectroscopy to estimate TBW. A correlation coefficient of 0·96 between bioelectrical impedance spectroscopy and a dilution method has been reportedReference Matthie, Zarowitz, De Lorenzo, Andreoli, Katzarski, Pan and Withers41. In results from our laboratory (AM Silva et al. unpublished results) we found that estimations of TBW using isotopic MS and 2H are highly correlated (r 0·92). Even though these high correlation coefficients were found between bioelectrical impedance spectroscopy and 2H dilution, one should consider that these two methods may yield limits of agreement that are close to 5 %. However, considering the four-compartment model used in the present study, this results in an error of ± 1·2 kg of FM based on a four-compartment model. Regardless, the use of a four-compartment model in the present study must be considered as a major strength. Other strengths include the validity assessment of a wide variety of alternative clinical and field methods to track body composition changes.
Conclusion
The findings of the present study highlight the fact that methods widely used in clinical settings should not be applied interchangeably to detect body composition changes after a weight-loss management programme. In conclusion, the results suggest that errors in body composition changes with weight loss assessed by DXA, BIA-Tanita, BF-300 and Antrform are similar when compared with a four-compartment model, though BIA-Tanita estimates of mean changes in body composition were unbiased when compared with the other clinical methods. Overall, a similar potential for individual accuracy was found in the detection of FM changes. However, DXA presented a better performance in predicting mean FFM changes from the reference model in comparison with the other methods. Furthermore, given the relatively larger average error of prediction with the techniques used in the present study, small physiological changes in body composition may not be accurately detected by these clinical methods after a weight-loss intervention.
Acknowledgements
The present study was supported by the Portuguese Foundation for Science and Technology.







