Published online by Cambridge University Press: 17 April 2009
Our main result is that if G(x1, …, xn) = 0 is a system of homogeneous equations such that for every partition of the positive integers into finitely many classes there are distinct y1,…, yn in one class such that G(y1, …, yn) = 0, then, for every partition of the positive integers into finitely many classes there are distinct Z1, …, Zn in one class such that
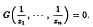
In particular, we show that if the positive integers are split into r classes, then for every n ≥ 2 there are distinct positive integers x1, x1, …, xn in one class such that
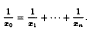
We also show that if [1, n6 − (n2 − n)2] is partitioned into two classes, then some class contains x0, x1, …, xn such that
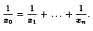
(Here, x0, x2, …, xn are not necessarily distinct.)