Published online by Cambridge University Press: 28 March 2019
Let 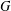 $G$ be a group,
$G$ be a group, 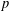 $p$ be a prime and
$p$ be a prime and 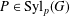 $P\in \text{Syl}_{p}(G)$. We say that a
$P\in \text{Syl}_{p}(G)$. We say that a 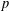 $p$-Brauer character
$p$-Brauer character 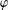 $\unicode[STIX]{x1D711}$ is monolithic if
$\unicode[STIX]{x1D711}$ is monolithic if 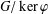 $G/\ker \unicode[STIX]{x1D711}$ is a monolith. We prove that
$G/\ker \unicode[STIX]{x1D711}$ is a monolith. We prove that  $P$ is normal in
$P$ is normal in 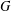 $G$ if and only if
$G$ if and only if 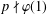 $p\nmid \unicode[STIX]{x1D711}(1)$ for each monolithic Brauer character
$p\nmid \unicode[STIX]{x1D711}(1)$ for each monolithic Brauer character 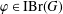 $\unicode[STIX]{x1D711}\in \text{IBr}(G)$. When
$\unicode[STIX]{x1D711}\in \text{IBr}(G)$. When 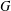 $G$ is
$G$ is 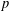 $p$-solvable, we also prove that
$p$-solvable, we also prove that 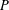 $P$ is normal in
$P$ is normal in 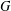 $G$ and
$G$ and 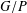 $G/P$ is nilpotent if and only if
$G/P$ is nilpotent if and only if 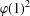 $\unicode[STIX]{x1D711}(1)^{2}$ divides
$\unicode[STIX]{x1D711}(1)^{2}$ divides 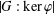 $|G:\ker \unicode[STIX]{x1D711}|$ for all monolithic irreducible
$|G:\ker \unicode[STIX]{x1D711}|$ for all monolithic irreducible 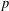 $p$-Brauer characters
$p$-Brauer characters 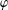 $\unicode[STIX]{x1D711}$ of
$\unicode[STIX]{x1D711}$ of 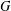 $G$.
$G$.
The first author is supported by the Fund for Young Key Teachers of Henan University of Technology, the Fund of Henan Administration of Foreign Experts Affairs, the Project of Henan Province (182102410049) and the NSFC (11571129 and 11771356).