Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Henson, C. Ward
1979.
Unbounded Loeb measures.
Proceedings of the American Mathematical Society,
Vol. 74,
Issue. 1,
p.
143.
Loeb, Peter A.
1979.
Weak limits of measures and the standard part map.
Proceedings of the American Mathematical Society,
Vol. 77,
Issue. 1,
p.
128.
Kessler, Christoph
1986.
Nonstandard conditions for the global Markov property for lattice fields.
Acta Applicandae Mathematicae,
Vol. 7,
Issue. 3,
p.
225.
1986.
Vol. 122,
Issue. ,
p.
225.
Stroyan, K. D.
1986.
Previsible sets for hyperfinite filtrations.
Probability Theory and Related Fields,
Vol. 73,
Issue. 2,
p.
183.
1986.
Foundations of Infinhesimal Stochastic Ankysis.
Vol. 119,
Issue. ,
p.
446.
Garling, D. J. H.
1986.
Another ‘short’ proof of the Riesz representation theorem.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 99,
Issue. 2,
p.
261.
Landers, D.
and
Rogge, L.
1987.
Universal Loeb-measurability of sets and of the standard part map with applications.
Transactions of the American Mathematical Society,
Vol. 304,
Issue. 1,
p.
229.
Keisler, H. Jerome
Kunen, Kenneth
Miller, Arnold
and
Leth, Steven
1989.
Descriptive set theory over hyperfinite sets.
The Journal of Symbolic Logic,
Vol. 54,
Issue. 4,
p.
1167.
Sun, Yeneng
1989.
A nonstandard proof of the Riesz representation theorem for weakly compact operators on C(Ω).
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 105,
Issue. 1,
p.
141.
Miller, Arnold W.
1990.
Set theoretic properties of Loeb measure.
Journal of Symbolic Logic,
Vol. 55,
Issue. 3,
p.
1022.
Živaljević, Boško
1990.
Some results about Borel sets in descriptive set theory of hyperfinite sets.
Journal of Symbolic Logic,
Vol. 55,
Issue. 2,
p.
604.
Ross, David
1990.
Lifting theorems in nonstandard measure theory.
Proceedings of the American Mathematical Society,
Vol. 109,
Issue. 3,
p.
809.
Keisler, H.Jerome
1991.
From discrete to continuous time.
Annals of Pure and Applied Logic,
Vol. 52,
Issue. 1-2,
p.
99.
Živaljević, Boško
1991.
The structure of graphs all of whose Y-sections are internal sets.
Journal of Symbolic Logic,
Vol. 56,
Issue. 1,
p.
50.
Živaljević, Boško
1991.
U-meager sets when the cofinality and the coinitiality of U are uncountable.
The Journal of Symbolic Logic,
Vol. 56,
Issue. 3,
p.
906.
Živaljević, Boško
1991.
Every Borel function is monotone Borel.
Annals of Pure and Applied Logic,
Vol. 54,
Issue. 1,
p.
87.
Keisler, H. Jerome
and
Leth, Steven C.
1991.
Meager sets on the hyperfinite time line.
Journal of Symbolic Logic,
Vol. 56,
Issue. 1,
p.
71.
Panetta, R. Lee
1992.
A finite intersection property and the Loeb measurability of ultrafilters on hyperfinite sets.
Annals of Mathematics and Artificial Intelligence,
Vol. 6,
Issue. 1-3,
p.
267.
Živaljević, Boško
1992.
Uniqueness of unbounded Loeb measure using Choquet’s theorem.
Proceedings of the American Mathematical Society,
Vol. 116,
Issue. 2,
p.
529.
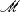 be a set theoretical structure and *
be a set theoretical structure and * 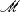 a nonstandard extension of
a nonstandard extension of 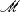 , as in the usual framework for nonstandard analysis (see [10]). Let X be a Hausdorff space in
, as in the usual framework for nonstandard analysis (see [10]). Let X be a Hausdorff space in 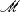 and stx the standard part map for X, defined on the set of nearstandard points in *X. Suppose, for example, µ is an internal, finitely additive probability measure defined on the internal subsets of *X.
and stx the standard part map for X, defined on the set of nearstandard points in *X. Suppose, for example, µ is an internal, finitely additive probability measure defined on the internal subsets of *X.