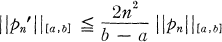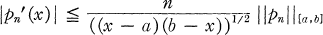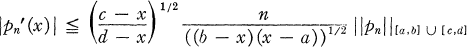Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Lukashov, A. L.
1999.
A bernstein type inequality for derivatives of rational functions on two intervals.
Mathematical Notes,
Vol. 66,
Issue. 4,
p.
415.
Лукашов, Алексей Леонидович
and
Lukashov, Aleksei Leonidovich
1999.
Неравенство типа Бернштейна для производных рациональных функций на двух отрезках.
Математические заметки,
Vol. 66,
Issue. 4,
p.
508.
Totik, Vilmos
2001.
Polynomial inverse images and polynomial inequalities.
Acta Mathematica,
Vol. 187,
Issue. 1,
p.
139.
Лукашов, Алексей Леонидович
and
Lukashov, Aleksei Leonidovich
2004.
Неравенства для производных рациональных функций на нескольких отрезках.
Известия Российской академии наук. Серия математическая,
Vol. 68,
Issue. 3,
p.
115.
Totik, Vilmos
2012.
Approximation Theory XIII: San Antonio 2010.
Vol. 13,
Issue. ,
p.
345.
Akturk, M.A.
and
Lukashov, A.
2016.
Sharp Markov-type inequalities for rational functions on several intervals.
Journal of Mathematical Analysis and Applications,
Vol. 436,
Issue. 2,
p.
1017.
Beberok, Tomasz
2020.
Markov’s Inequality and $$C^{\infty }$$ Functions on Certain Algebraic Hypersurfaces.
Bulletin of the Malaysian Mathematical Sciences Society,
Vol. 43,
Issue. 6,
p.
4303.
Sroka, Grzegorz
2022.
Constants in Markov’s and Bernstein inequality on a finite interval in $${\mathbb {R}}$$.
Analysis and Mathematical Physics,
Vol. 12,
Issue. 5,