No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
We shall consider quasi-fields which satisfy the multiplicative Identity
1.1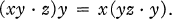
(1.1) will be called the right Bol law and a quasi-field satisfying it will be called a right Bol quasi-held. Moufang quasi-fields, i.e., those satisfying the Moufang identity
1.2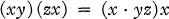
were studied in (5). Quasi-fields satisfying the left Bol identity
1.3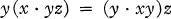
were studied by Burn (3) and the author (6). Such quasi-fields are called Bol quasi-fields.
Our investigation will parallel the investigations in (5; 6). In § 2 we derive necessary and sufficient conditions for a right Bol quasi-field to be an alternative division ring and also criteria for it to be a near-field. With this information we derive in §§ 3 and 4 new characterizations of Moufang planes similar to those in (5; 6).
Loops satisfying (1.1) have been studied by Robinson (10). He calls such loops Bol loops.