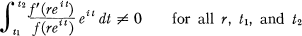Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Başgöze, T.
and
Keogh, F. R.
1970.
The Hardy class of a spiral-like function and its derivative.
Proceedings of the American Mathematical Society,
Vol. 26,
Issue. 2,
p.
266.
Ziegler, M. R.
1972.
A class of regular functions containing spirallike and close-to-convex functions.
Transactions of the American Mathematical Society,
Vol. 166,
Issue. 0,
p.
59.
Eenigenburg, P. J.
1972.
Boundary behavior of starlike functions.
Proceedings of the American Mathematical Society,
Vol. 33,
Issue. 2,
p.
428.
Libera, Richard J.
and
Ziegler, Michael R.
1972.
Regular functions 𝑓(𝑧) for which 𝑧𝑓’(𝑧) is 𝛼-spiral.
Transactions of the American Mathematical Society,
Vol. 166,
Issue. 0,
p.
361.
Kulshrestha, P.K
1973.
Generalized convexity in conformal mappings.
Journal of Mathematical Analysis and Applications,
Vol. 43,
Issue. 2,
p.
441.
Silvia, E. M.
1974.
On a subclass of spiral-like functions.
Proceedings of the American Mathematical Society,
Vol. 44,
Issue. 2,
p.
411.
Sizhuk, P. I.
and
Chernikov, V. V.
1975.
On some properties of schlicht functions.
Mathematical Notes of the Academy of Sciences of the USSR,
Vol. 17,
Issue. 4,
p.
332.
Nasr, M A
and
Aouf, M K
1983.
Bounded starlike functions of complex order.
Proceedings of the Indian Academy of Sciences - Section A,
Vol. 92,
Issue. 2,
p.
97.
Bhatia, B.L.
and
Rajasekaran, S.
1983.
Coefficient estimates for alpha-spiral functions.
Bulletin of the Australian Mathematical Society,
Vol. 28,
Issue. 3,
p.
319.
Juneja, O. P.
and
Rajasekaran, S.
1986.
Coefficient estimates for inverses of α-spiral functions.
Complex Variables, Theory and Application: An International Journal,
Vol. 6,
Issue. 2-4,
p.
99.
Ahuja, Om P.
and
Jain, Pawan K.
1986.
On the spiral-likeness of rational functions.
Rendiconti del Circolo Matematico di Palermo,
Vol. 35,
Issue. 3,
p.
376.
Ahuja, O.P.
1993.
On the generalised Ruscheweyh class of analytic functions of complex order.
Bulletin of the Australian Mathematical Society,
Vol. 47,
Issue. 2,
p.
247.
Rao, S. Nanjunda
and
Latha, S.
1994.
Convex combinations of n analytic functions in generalized Ruscheweyh class.
International Journal of Mathematical Education in Science and Technology,
Vol. 25,
Issue. 6,
p.
791.
Altintaş, Osman
Özkan, Öznur
and
Srivastava, H.M.
2001.
Majorization by starlike functions of complex order.
Complex Variables, Theory and Application: An International Journal,
Vol. 46,
Issue. 3,
p.
207.
Saltik, Gülşah
Deni̇z, Erhan
and
Kadioğlu, Ekrem
2010.
Two new general p-valent integral operators.
Mathematical and Computer Modelling,
Vol. 52,
Issue. 9-10,
p.
1605.
Abubaker, Afaf A. Ali
Darus, Maslina
and
Breaz, Daniel
2011.
Majorization for A Subclass of β-Spiral Functions of Order α Involving a Generalized Linear Operator.
Advances in Decision Sciences,
Vol. 2011,
Issue. ,
p.
1.
Murugusundaramoorthy, G.
2012.
Subordination results for spiral-like functions associated with the Srivastava–Attiya operator.
Integral Transforms and Special Functions,
Vol. 23,
Issue. 2,
p.
97.
Xu, Qing-Hua
Lv, Chun-Bo
and
Srivastava, H.M.
2013.
Coefficient estimates for the inverses of a certain general class of spirallike functions.
Applied Mathematics and Computation,
Vol. 219,
Issue. 12,
p.
7000.
Frasin, B. A.
2013.
Sufficient Conditions for -Spirallike and -Robertson Functions of Complex Order.
Journal of Mathematics,
Vol. 2013,
Issue. ,
p.
1.
Tang, Huo
Li, Shu-hai
and
Deng, Guan-tie
2014.
Majorization properties for a new subclass of θ-spiral functions of order γ
.
Mathematica Slovaca,
Vol. 64,
Issue. 1,
p.
39.