Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-11T00:22:26.829Z Has data issue: false hasContentIssue false
  • English
  • Français

Block Decomposition and Weighted Hausdorff Content

Part of: Harmonic analysis in several variables

Published online by Cambridge University Press:  16 December 2019

Hiroki Saito ,
Hitoshi Tanaka  and
Toshikazu Watanabe
Hiroki Saito
Affiliation:
College of Science and Technology, Nihon University, Narashinodai 7-24-1, Funabashi City, Chiba, 274-8501, Japan Email: saitou.hiroki@nihon-u.ac.jp
Hitoshi Tanaka
Affiliation:
Research and Support Center on Higher Education for the hearing and Visually Impaired, National University Corporation Tsukuba University of Technology, Kasuga 4-12-7, Tsukuba City, Ibaraki, 305-8521, Japan Email: htanaka@k.tsukuba-tech.ac.jp
Toshikazu Watanabe
Affiliation:
College of Science and Technology, Nihon University, 1-8-14 Kanda-Surugadai, Chiyoda-ku, Tokyo, 101-8308, Japan Email: twatana@edu.tuis.ac.jp
Get access Rights & Permissions [Opens in a new window]

Abstract

Block decomposition of 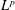$L^{p}$ spaces with weighted Hausdorff content is established for 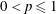$0<p\leqslant 1$ and the Fefferman–Stein type inequalities are shown for fractional integral operators and some variants of maximal operators.

Keywords

block decompositionChoquet integralFefferman–Stein inequalityfractional integral operatorfractional maximal operatorweighted Hausdorff content

MSC classification

Primary: 42B25: Maximal functions, Littlewood-Paley theory
Secondary: 42B35: Function spaces arising in harmonic analysis
Type
Article
Information
Canadian Mathematical Bulletin , Volume 63 , Issue 1 , March 2020 , pp. 141 - 156
Copyright
© Canadian Mathematical Society 2019

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Author H. S. is supported by Grant-in-Aid for Young Scientists (19K14577), the Japan Society for the Promotion of Science. Author H. T. is supported by Grant-in-Aid for Scientific Research (C) (15K04918 and 19K03510), the Japan Society for the Promotion of Science.

References

Adams, D. R., A note on the Choquet integrals with respect to Hausdorff capacity. In: Function spaces and applications. Proc. Lund 1986, Lecture Notes in Math., 1302, Springer, Berlin, 1988, pp. 115–124.CrossRefGoogle Scholar
Adams, D. R., Choquet integrals in potential theory. Publ. Mat. 42(1998), 3–66.CrossRefGoogle Scholar
Adams, D. and Xiao, J., Nonlinear potential analysis on Morrey spaces and their capacities. Indiana Univ. Math. J. 53(2004), 1629–1663.CrossRefGoogle Scholar
Bernardis, A. L., Pradolini, G., Lorente, M., and Riveros, and M. S., Composition of fractional Orlicz maximal operators and A 1-weights on spaces of homogeneous type. Acta Math. Sinica 26(2010), 1509–1518.CrossRefGoogle Scholar
Carozza, M. and Passarelli Di Napoli, A., Composition of maximal operators. Publ. Mat. 40(1996), 397–409.CrossRefGoogle Scholar
Cruz-Uribe, D., SFO, Two weight norm inequalities for fractional integral operators and commutators. arxiv:1412.4157v1 [math.CA] 12 Dec 2014.Google Scholar
Muckenhoupt, B. and Wheeden, R. L., Weighted norm inequalities for fractional integrals. Trans. Amer. Math. Soc. 192(1974), 261–274.CrossRefGoogle Scholar
Orobitg, J. and Verdera, J., Choquet integrals, Hausdorff content and the Hardy–Littlewood maximal operator. Bull. London Math. Soc. 30(1998), 145–150.CrossRefGoogle Scholar
Pérez, C., Sharp estimates for commutators of singular integrals via iterations of the Hardy–Littlewood maximal function. J. Fourier Anal. Appl. 3(1997), 743–756.CrossRefGoogle Scholar
Saito, H., Boundedness of the strong maximal operator with the Hausdorff content. Bull. Korean Math. Soc. 56(2019), no. 2, 399–406.Google Scholar
Saito, H., Tanaka, H., and Watanabe, T., Abstract dyadic cubes and the dyadic maximal operator with the Hausdorff content. Bull. Sci. Math. 140(2016), 757–773.CrossRefGoogle Scholar
Saito, H., Tanaka, H., and Watanabe, T., Fractional maximal operators with weighted Hausdorff content. Positivity 23(2019), 125–138.CrossRefGoogle Scholar
Sawano, Y. and Tanaka, H., The Fatou property of block spaces. J. Math. Sci. Univ. Tokyo 22(2015), 663–683.Google Scholar
Sawyer, E., Weighted norm inequalities for fractional maximal operators. Proc. Canad. Math. Spc. 1(1981), 283–309.Google Scholar
Sawyer, E. and Wheeden, R., Weighted inequalities for fractional integrals on Euclidean and homogeneous spaces. Amer. J. Math. 114(1992), 813–874.CrossRefGoogle Scholar
Tang, L., Choquet integrals, weighted Hausdorff content and maximal operators. Georgian Math. J. 18(2011), 587–596.Google Scholar
Turesson, B. O., Nonlinear potential theory and weighted Sobolev spaces. Lecture Notes in Math., 1736, Springer-Verlag, Berlin, 2000.CrossRefGoogle Scholar
Zorko, C. T., Morrey space. Proc. Amer. Math. Soc. 98(1986), 586–592.CrossRefGoogle Scholar