On the binomial transforms of Apéry-like sequences

Abstract

In his proof of the irrationality of 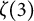$\zeta (3)$ and 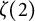$\zeta (2)$, Apéry defined two integer sequences through $3$-term recurrences, which are known as the famous Apéry numbers. Zagier, Almkvist–Zudilin, and Cooper successively introduced the other $13$ sporadic sequences through variants of Apéry’s 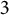$3$-term recurrences. All of the 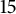$15$ sporadic sequences are called Apéry-like sequences. Motivated by Gessel’s congruences mod $24$ for the Apéry numbers, we investigate congruences of the form 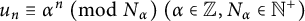$u_n\equiv \alpha ^n \ \pmod {N_{\alpha }}~(\alpha \in \mathbb {Z},N_{\alpha }\in \mathbb {N}^{+})$ for all of the $15$ Apéry-like sequences $\{u_n\}_{n\ge 0}$. Let 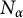$N_{\alpha }$ be the largest positive integer such that 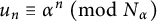$u_n\equiv \alpha ^n\ \pmod {N_{\alpha }}$ for all non-negative integers n. We determine the values of 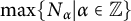$\max \{N_{\alpha }|\alpha \in \mathbb {Z}\}$ for all of the 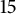$15$ Apéry-like sequences 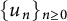$\{u_n\}_{n\ge 0}$. The binomial transforms of Apéry-like sequences provide us a unified approach to this type of congruences for Apéry-like sequences.