Convergence Rates of Cascade Algorithms with Infinitely Supported Masks

Abstract

We investigate the solutions of refinement equations of the form

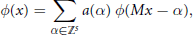$$\phi (x)\,=\,\sum\limits_{\alpha \in {{\mathbb{Z}}^{S}}}{a(\alpha )\,}\phi (Mx\,-\,\alpha ),$$

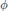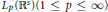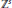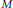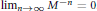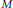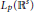where the function $\phi $ is in ${{L}_{p}}({{\mathbb{R}}^{s}})(1\,\le \,p\,\le \,\infty )$, $a$ is an infinitely supported sequence on ${{\mathbb{Z}}^{s}}$ called a refinement mask, and $M$ is an $s\,\times \,s$ integer matrix such that ${{\lim }_{n\to \infty }}\,{{M}^{-n}}\,=\,0$. Associated with the mask $a$ and $M$ is a linear operator ${{\text{Q}}_{a,M}}$ defined on ${{L}_{p}}({{\mathbb{R}}^{s}})$ by 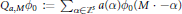${{\text{Q}}_{a,M}}{{\phi }_{0}}\,:=\,{{\sum }_{\alpha \in {{\mathbb{Z}}^{s}}}}\,a(\alpha ){{\phi }_{0}}(M\,\cdot \,-\alpha )$. Main results of this paper are related to the convergence rates of ${{(\text{Q}_{a,M}^{n}{{\phi }_{o}})}_{n=1,2,\ldots }}$ in 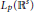${{L}_{p}}({{\mathbb{R}}^{s}})$ with mask $a$ being infinitely supported. It is proved that under some appropriate conditions on the initial function 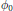${{\phi }_{0}}$, 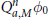$\text{Q}_{a,M}^{n}{{\phi }_{0}}$ converges in 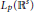${{L}_{p}}({{\mathbb{R}}^{s}})$ with an exponential rate.