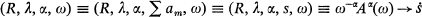Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Ziza, O. A.
1979.
Summation of orthogonal series by the methods (?, ?).
Mathematical Notes of the Academy of Sciences of the USSR,
Vol. 25,
Issue. 2,
p.
91.
2017.
An Introductory Course in Summability Theory.
p.
85.


 be an arbitrary series with partial sums
be an arbitrary series with partial sums