No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
The celebrated Fredholm theory of linear integral equations holds if the kernel K(x, y) or one of its iterates K(n) is bounded. Hilbert utilizing his theory of quadratic form was able to extend the theory to the kernels K(x, y) satisfying
a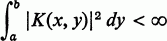
b
where k is independent of u(x).
These theories were extended considerably by T. Carleman who deleted condition (b) above.
Equations involving this Carleman kernel have been found useful in connection with Hermitian forms, continued fractions, Schroedinger wave equations (see [1], [2]) and more recently in scattering theory in quantum physics, etc. [3]. See also [5] for a variety of applications and extensions.