Article contents
On the Largest Dynamic Monopolies of Graphs with a Given Average Threshold
Published online by Cambridge University Press: 20 November 2018
Abstract
Let 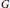 $G$ be a graph and let
$G$ be a graph and let  $\tau $ be an assignment of nonnegative integer thresholds to the vertices of
$\tau $ be an assignment of nonnegative integer thresholds to the vertices of 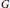 $G$. A subset of vertices,
$G$. A subset of vertices, 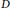 $D$, is said to be a
$D$, is said to be a  $\tau $-dynamicmonopoly if
$\tau $-dynamicmonopoly if  $V\left( G \right)$ can be partitioned into subsets
$V\left( G \right)$ can be partitioned into subsets 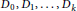 ${{D}_{0}},\,{{D}_{1}},\,\ldots \,,\,{{D}_{k}}$ such that
${{D}_{0}},\,{{D}_{1}},\,\ldots \,,\,{{D}_{k}}$ such that 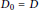 ${{D}_{0}}\,=\,D$ and for any
${{D}_{0}}\,=\,D$ and for any 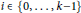 $i\,\in \,\left\{ 0,\,\ldots \,,\,k-1 \right\}$, each vertex
$i\,\in \,\left\{ 0,\,\ldots \,,\,k-1 \right\}$, each vertex  $v$ in
$v$ in 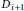 ${{D}_{i+1}}$ has at least
${{D}_{i+1}}$ has at least 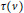 $\tau \left( v \right)$ neighbors in
$\tau \left( v \right)$ neighbors in 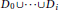 ${{D}_{0}}\cup \cdots \cup {{D}_{i}}$. Denote the size of smallest
${{D}_{0}}\cup \cdots \cup {{D}_{i}}$. Denote the size of smallest  $\tau $-dynamic monopoly by
$\tau $-dynamic monopoly by 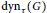 $\text{dy}{{\text{n}}_{\tau }}\left( G \right)$ and the average of thresholds in
$\text{dy}{{\text{n}}_{\tau }}\left( G \right)$ and the average of thresholds in  $\tau $ by
$\tau $ by  $\bar{\tau }$. We show that the values of
$\bar{\tau }$. We show that the values of 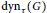 $\text{dy}{{\text{n}}_{\tau }}\left( G \right)$ over all assignments
$\text{dy}{{\text{n}}_{\tau }}\left( G \right)$ over all assignments  $\tau $ with the same average threshold is a continuous set of integers. For any positive number
$\tau $ with the same average threshold is a continuous set of integers. For any positive number 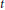 $t$, denote the maximum
$t$, denote the maximum 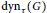 $\text{dy}{{\text{n}}_{\tau }}\left( G \right)$ taken over all threshold assignments
$\text{dy}{{\text{n}}_{\tau }}\left( G \right)$ taken over all threshold assignments  $\tau $ with
$\tau $ with  $\bar{\tau }\,\le \,t$, by
$\bar{\tau }\,\le \,t$, by  $\text{Ldy}{{\text{n}}_{t}}\left( G \right)$. In fact,
$\text{Ldy}{{\text{n}}_{t}}\left( G \right)$. In fact,  $\text{Ldy}{{\text{n}}_{t}}\left( G \right)$ shows the worst-case value of a dynamicmonopoly when the average threshold is a given number
$\text{Ldy}{{\text{n}}_{t}}\left( G \right)$ shows the worst-case value of a dynamicmonopoly when the average threshold is a given number 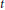 $t$. We investigate under what conditions on
$t$. We investigate under what conditions on 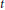 $t$, there exists an upper bound for
$t$, there exists an upper bound for  $\text{Ldy}{{\text{n}}_{t}}\left( G \right)$ of the form
$\text{Ldy}{{\text{n}}_{t}}\left( G \right)$ of the form 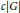 $c\left| G \right|$, where
$c\left| G \right|$, where 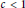 $c\,<\,1$. Next, we show that
$c\,<\,1$. Next, we show that  $\text{Ldy}{{\text{n}}_{t}}\left( G \right)$ is
$\text{Ldy}{{\text{n}}_{t}}\left( G \right)$ is  $\text{coNP}$-hard for planar graphs but has polynomial-time solution for forests.
$\text{coNP}$-hard for planar graphs but has polynomial-time solution for forests.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2015
References
- 2
- Cited by


