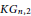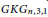Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Ganesamurthy, S.
and
Paulraja, P.
2018.
Existence of a P2k+1-decomposition in the Kneser graph KGt,2
.
Discrete Mathematics,
Vol. 341,
Issue. 7,
p.
2113.
C., Cecily Sahai
S., Sampath Kumar
and
T., Arputha Jose
2025.
Four cycle decomposition of λK(n,2).
Discrete Applied Mathematics,
Vol. 372,
Issue. ,
p.
65.
Sahai, C. Cecily
Kumar, S. Sampath
and
Jose, T. Arputha
2025.
A note on Pk-decomposition of the Kneser graph.
Discrete Mathematics, Algorithms and Applications,
Vol. 17,
Issue. 04,