Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-27T23:04:48.623Z Has data issue: false hasContentIssue false
  • English
  • Français

A Short Note on the Higher Level Version of the Krull–Baer Theorem

Published online by Cambridge University Press:  20 November 2018

Dejan Velušček
Dejan Velušček*
Affiliation:
University of Ljubljana, Faculty of Mathematics and Physics, Department of Mathematics, Ljubljana, Sloveniae-mail: dejan.veluscek@fmf.uni-lj.si
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

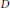Klep and Velušček generalized the Krull–Baer theorem for higher level preorderings to the non-commutative setting. A $n$-real valuation $v$ on a skew field $D$ induces a group homomorphism 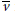$\overline{v}$. A section of 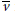$\overline{v}$ is a crucial ingredient of the construction of a complete preordering on the base field 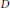$D$ such that its projection on the residue skew field 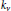${{k}_{v}}$ equals the given level 1 ordering on 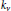${{k}_{v}}$. In the article we give a proof of the existence of the section of 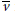$\overline{v}$, which was left as an open problem by Klep and Velušček, and thus complete the generalization of the Krull–Baer theorem for preorderings.

Keywords

14P9906Fxxorderings of higher leveldivision ringsvaluations
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 54 , Issue 2 , 01 June 2011 , pp. 381 - 384
Copyright
Copyright © Canadian Mathematical Society 2011

References

[1] Becker, E., Summen n-ter Potenzen in Körpern. J. Reine Angew. Math. 307/308(1979), 8–30.Google Scholar
[2] Klep, I., Velušček, D., n-real valuations and the higher level version of the Krull–Baer theorem. J. Algebra 279(2004), no. 1, 345–361. doi:10.1016/j.jalgebra.2004.05.012Google Scholar
[3] Fuchs, L., Infinite abelian groups. Vol. I. Pure and Applied Mathematics, 36, Academic Press, New York-London, 1970.Google Scholar
[4] Schilling, O. F. G., The theory of valuations, Mathematical Surveys, 4, American Mathematical Society, New York, 1950.Google Scholar
[5] Powers, V., Holomorphy rings and higher level orders on skew fields. J. Algebra 136(1991), no. 1, 51–59. doi:10.1016/0021-8693(91)90063-EGoogle Scholar