Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-26T06:05:27.107Z Has data issue: false hasContentIssue false
  • English
  • Français

A Singular Critical Potential for the Schrödinger Operator

Published online by Cambridge University Press:  20 November 2018

Thomas Duyckaerts
Thomas Duyckaerts*
Affiliation:
Département de mathématiques, Université de Cergy-Pontoise, 2 avenue Adolphe Chauvin, 95302 Cergy-Pontoise Cedex, France e-mail: Thomas.Duyckaerts@u-cergy.fr
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Consider a real potential 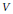$V$ on 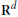${{\text{R}}^{d}}$, 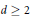$d\ge 2$, and the Schrödinger equation:

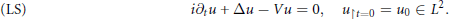$$\left( \text{LS} \right)\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,i{{\partial }_{t}}u+\Delta u-Vu=0,{{u}_{\upharpoonright }}_{t=0}={{u}_{0}}\in {{L}^{2}}$$

In this paper, we investigate the minimal local regularity of 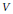$V$ needed to get local in time dispersive estimates (such as local in time Strichartz estimates or local smoothing effect with gain of 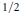$1/2$ derivative) on solutions of 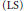$\left( \text{LS} \right)$. Prior works show some dispersive properties when 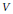$V$ (small at infinity) is in ${{L}^{d/2}}$ or in spaces just a little larger but with a smallness condition on 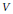$V$ (or at least on its negative part).

In this work, we prove the critical character of these results by constructing a positive potential 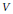$V$ which has compact support, bounded outside 0 and of the order 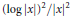${{\left( \log \left| x \right| \right)}^{2}}/{{\left| x \right|}^{2}}$ near 0. The lack of dispersiveness comes from the existence of a sequence of quasimodes for the operator 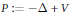$P:=-\Delta +V$.

The elementary construction of 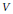$V$ consists in sticking together concentrated, truncated potential wells near 0. This yields a potential oscillating with infinite speed and amplitude at 0, such that the operator $P$ admits a sequence of quasi-modes of polynomial order whose support concentrates on the pole.

Keywords

35B6535L0535Q4035Q55
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 50 , Issue 1 , 01 March 2007 , pp. 35 - 47
Copyright
Copyright © Canadian Mathematical Society 2007

References

[1] Banica, V., Dispersion and Strichartz inequalities for Schrödinger equations with singular coefficients. SIAM J. Math. Anal. 35(2003), no. 4, 868–883.Google Scholar
[2] Burq, N., Semi-classical estimates for the resolvent in nontrapping geometries. Internat. Math. Res. Notices (2002), no. 5, 221–241.Google Scholar
[3] Burq, N., Smoothing effect for Schrödinger boundary value problems. Duke Math. J. 123(2004), no. 2, 403–427.Google Scholar
[4] Burq, N., Gérard, P., and Tzvetkov, N., On nonlinear Schrödinger equations in exterior domains. Ann. Inst. H. Poincaré Anal. Non Linéaire 21(2004), no. 3, 295–318.Google Scholar
[5] Burq, N., Planchon, F., Stalker, J. G., and Tahvildar-Zadeh, A. Shadi, Strichartz estimates for the wave and Schrödinger equations with the inverse-square potential. J. Funct. Anal. 203(2003), no. 2, 519–549.Google Scholar
[6] Castro, C. and Zuazua, E., Concentration and lack of observability of waves in highly heterogeneous media. Arch. Rational Mech. Anal. 164(2002), no. 1, 39–72.Google Scholar
[7] Constantin, P. and Saut, J. C., Local smoothing properties of Schrödinger equations. Indiana Univ. Math. J. 38(1989), no. 3, 791–810.Google Scholar
[8] D’Ancona, P. and Pierfelice, V., On the wave equation with a large rough potential. J. Funct. Anal. 227(2005), no. 1, 30–77.Google Scholar
[9] Duyckaerts, T., Inégalités de résolvante pour l’opérateur de Schrödinger avec potentiel multipolaire critique. Bull. Soc. Math. France 134(2006), no. 2, 201–239.Google Scholar
[10] Goldberg, M., Dispersive bounds for the three-dimensional Schrödinger equation with almost critical potentials. Preprint http://www.math.jhu.edu/_mikeg/3dim.pdf.Google Scholar
[11] Reed, M. and Simon, B., Methods of Modern Mathematical Physics. II. Fourier analysis, self-adjointness. Academic Press, San Diego, 1975.Google Scholar
[12] Rodnianski, I. and Schlag, W.. Time decay for solutions of Schrödinger equations with rough and time-dependent potentials. Invent. Math. 155(2004), no. 3, 451–513.Google Scholar
[13] Ruiz, A. and Vega, L., Local regularity of solutions to wave with time-dependent potentials. Duke Math. J. 76(1994), no. 3, 913–940.Google Scholar
[14] Sjölin, P., Regularity of solutions to the Schrödinger equation. Duke Math. J. 55(1987), no. 3, 699–715.Google Scholar
[15] Smith, H. F. and Sogge, C. D., Global Strichartz estimates for non-trapping perturbations of the Laplacian. Comm. Partial Differential Equations 25(2000), no. 11–12, 2171–2183.Google Scholar
[16] Vega, L., Schrödinger equations: pointwise convergence to the initial data. Proc. Amer.Math. Soc. 102(1988), no. 4, 874–878.Google Scholar