Published online by Cambridge University Press: 20 November 2018
We carry out the computation of the Iwasawa invariants  $\rho _{S}^{T},\,\mu _{S}^{T},\,\lambda _{S}^{T}$ associated to abelian
$\rho _{S}^{T},\,\mu _{S}^{T},\,\lambda _{S}^{T}$ associated to abelian 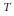 $T$-ramified
$T$-ramified 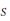 $S$-decomposed
$S$-decomposed 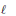 $\ell$-extensions over the finite steps
$\ell$-extensions over the finite steps 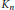 ${{K}_{n}}$ of the cyclotomic
${{K}_{n}}$ of the cyclotomic
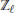 ${{\mathbb{Z}}_{\ell }}$
-extension
${{\mathbb{Z}}_{\ell }}$
-extension  ${{K}_{\infty }}/K$ of a number field of CM-type.
${{K}_{\infty }}/K$ of a number field of CM-type.
Nous déterminons explicitement les paramétres d'Iwasawa  $\rho _{S}^{T},\,\mu _{S}^{T},\,\lambda _{S}^{T}$ des
$\rho _{S}^{T},\,\mu _{S}^{T},\,\lambda _{S}^{T}$ des 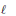 $\ell$-groupes de
$\ell$-groupes de 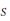 $S$-classes
$S$-classes 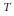 $T$-infinitésimales
$T$-infinitésimales
 $\text{Cl}_{S}^{T}\left( {{K}_{n}} \right)$
attachés aux étages finis de la
$\text{Cl}_{S}^{T}\left( {{K}_{n}} \right)$
attachés aux étages finis de la 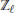 ${{\mathbb{Z}}_{\ell }}$-extension cyclotomique
${{\mathbb{Z}}_{\ell }}$-extension cyclotomique  ${{K}_{\infty }}/K$ d'un corps de nombres à conjugaison complexe.
${{K}_{\infty }}/K$ d'un corps de nombres à conjugaison complexe.