On the asymptotic properties of a simple estimate of the Mode

Abstract

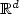We consider an estimate of the mode θ of a multivariate probability density f with support in $\mathbb R^d$ using a kernel estimate f _n drawn from a sample X₁,...,X_n . The estimate θ_n is defined as any x in {X₁,...,X_n } such that $f_n(x)=\max_{i=1, \hdots,n} f_n(X_i)$ 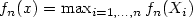. It is shown that θ_n behaves asymptotically as any maximizer ${\hat{\theta}}_n$ 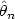of f _n . More precisely, we prove that for any sequence $(r_n)_{n\geq 1}$ 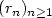of positive real numbers such that $r_n\to\infty$ and $r_n^d\log n/n\to 0$ , one has $r_n\,\|\theta_n-{\hat{\theta}}_n\| \to 0$ 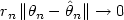in probability. The asymptotic normality of θ_n follows without further work.