Article contents
SYMMETRIC AND ASYMMETRIC RAMSEY PROPERTIES IN RANDOM HYPERGRAPHS
Published online by Cambridge University Press: 23 November 2017
Abstract
A celebrated result of Rödl and Ruciński states that for every graph  $F$ , which is not a forest of stars and paths of length 3, and fixed number of colours
$F$ , which is not a forest of stars and paths of length 3, and fixed number of colours 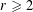 $r\geqslant 2$ there exist positive constants
$r\geqslant 2$ there exist positive constants 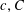 $c,C$ such that for
$c,C$ such that for 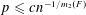 $p\leqslant cn^{-1/m_{2}(F)}$ the probability that every colouring of the edges of the random graph
$p\leqslant cn^{-1/m_{2}(F)}$ the probability that every colouring of the edges of the random graph  $G(n,p)$ contains a monochromatic copy of
$G(n,p)$ contains a monochromatic copy of 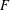 $F$ is
$F$ is  $o(1)$ (the ‘0-statement’), while for
$o(1)$ (the ‘0-statement’), while for 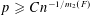 $p\geqslant Cn^{-1/m_{2}(F)}$ it is
$p\geqslant Cn^{-1/m_{2}(F)}$ it is 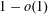 $1-o(1)$ (the ‘1-statement’). Here
$1-o(1)$ (the ‘1-statement’). Here 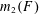 $m_{2}(F)$ denotes the 2-density of
$m_{2}(F)$ denotes the 2-density of 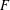 $F$ . On the other hand, the case where
$F$ . On the other hand, the case where 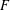 $F$ is a forest of stars has a coarse threshold which is determined by the appearance of a certain small subgraph in
$F$ is a forest of stars has a coarse threshold which is determined by the appearance of a certain small subgraph in 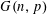 $G(n,p)$ . Recently, the natural extension of the 1-statement of this theorem to
$G(n,p)$ . Recently, the natural extension of the 1-statement of this theorem to 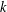 $k$ -uniform hypergraphs was proved by Conlon and Gowers and, independently, by Friedgut, Rödl and Schacht. In particular, they showed an upper bound of order
$k$ -uniform hypergraphs was proved by Conlon and Gowers and, independently, by Friedgut, Rödl and Schacht. In particular, they showed an upper bound of order  $n^{-1/m_{k}(F)}$ for the 1-statement, where
$n^{-1/m_{k}(F)}$ for the 1-statement, where  $m_{k}(F)$ denotes the
$m_{k}(F)$ denotes the 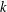 $k$ -density of
$k$ -density of 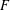 $F$ . Similarly as in the graph case, it is known that the threshold for star-like hypergraphs is given by the appearance of small subgraphs. In this paper we show that another type of threshold exists if
$F$ . Similarly as in the graph case, it is known that the threshold for star-like hypergraphs is given by the appearance of small subgraphs. In this paper we show that another type of threshold exists if  $k\geqslant 4$ : there are
$k\geqslant 4$ : there are  $k$ -uniform hypergraphs for which the threshold is determined by the asymmetric Ramsey problem in which a different hypergraph has to be avoided in each colour class. Along the way we obtain a general bound on the 1-statement for asymmetric Ramsey properties in random hypergraphs. This extends the work of Kohayakawa and Kreuter, and of Kohayakawa, Schacht and Spöhel who showed a similar result in the graph case. We prove the corresponding 0-statement for hypergraphs satisfying certain balancedness conditions.
$k$ -uniform hypergraphs for which the threshold is determined by the asymmetric Ramsey problem in which a different hypergraph has to be avoided in each colour class. Along the way we obtain a general bound on the 1-statement for asymmetric Ramsey properties in random hypergraphs. This extends the work of Kohayakawa and Kreuter, and of Kohayakawa, Schacht and Spöhel who showed a similar result in the graph case. We prove the corresponding 0-statement for hypergraphs satisfying certain balancedness conditions.
MSC classification
Information
- Type
- Research Article
- Information
- Creative Commons
- This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
- Copyright
- © The Author(s) 2017
References
- 12
- Cited by


