1 Introduction
The introduction of the chirped pulse amplification (CPA) technique more than thirty years ago[Reference Strickland and Mourou1] has enabled the development of high-intensity, ultra-short laser systems that opened up new horizons for a wide range of experiments involving sub-picosecond light–matter interactions. New research areas, such as astrophysics in the laboratory[Reference Remington, Arnett, Drake and Takabe2], high-energy-density experiments[Reference Roth, Cowan, Key, Hatchett, Brown, Fountain, Johnson, Pennington, Snavely, Wilks, Yasuike, Ruhl, Pegoraro, Bulanov, Campbell, Perry and Powell3] and novel schemes for particle acceleration[Reference Bartal, Foord, Bellei, Key, Flippo, Gaillard, Offermann, Patel, Jarrott, Higginson, Roth, Otten, Kraus, Stephens, McLean, Giraldez, Wei, Gautier and Beg4, Reference Ledingham and Galster5] are explored in large-scale facilities as well as more modest table-top laboratories. Prominent among other studies is laser-based ion acceleration, mainly due to its possible significant impact on hadron therapy of cancer. A noteworthy scheme is target normal sheath acceleration (TNSA), where the acceleration originates from thin foils irradiated by high-intensity (
![]() ${>}10^{18}~\text{W}/\text{cm}^{2}$
), short-pulse lasers.
${>}10^{18}~\text{W}/\text{cm}^{2}$
), short-pulse lasers.
During this process, multi-MeV range beams[Reference Clark, Krushelnick, Zepf, Beg, Tatarakis, Machacek, Santala, Watts, Norreys and Dangor6–Reference Mackinnon, Sentoku, Patel, Price, Hatchett, Key, Andersen, Snavely and Freeman8], tightly confined in time (picosecond scale) and space (few
![]() $\unicode[STIX]{x03BC}\text{m}$
radius), are produced. The conventional understanding of the underlying process is laser heating of the target’s electrons, thus producing a ‘hot’ electron cloud at the vicinity of its back surface. Some of them are energetic enough to escape and a positive unbalanced charge is left on the target. This process is responsible for building up an electrostatic potential, which in turn governs the ion acceleration[Reference Krygier, Schumacher and Freeman9, Reference Macchi, Borghesi and Passoni10], occurring typically in a sub-picosecond timescale. During the potential setup, the electronic cloud locked near the target, within a distance comparable to the Debye length[Reference Badziak, Głowacz, Jabłoński, Parys, Wołowski, Hora, Krása, Láska and Rohlena11], is thermalizing and some energetic electrons are still escaping from the target. Finally, this process ends when electrons cannot overcome the electrostatic potential induced near the target surface, and a second slower expansion–relaxation process takes over[Reference Dubois, Lubrano-Lavaderci, Raffestin, Ribolzi, Gazave, Compant La Fontaine, d’Humières, Hulin, Nicolaï, Poyé and Tikhonchuk12]. For sub-picosecond laser pulse irradiation, target charge neutralization by the electrons coming from outer-target, darkened sections can be neglected: the amount of escaped energetic electrons corresponds to the net positive charge left on the target surface[Reference Dubois, Lubrano-Lavaderci, Raffestin, Ribolzi, Gazave, Compant La Fontaine, d’Humières, Hulin, Nicolaï, Poyé and Tikhonchuk12]. The subsequent cooling process, including multiple collisions with the surrounding ions, sets the upper limit of the time range of target charging (that is, the effective lifetime of the potential barrier). Moreover, the locked hot electrons, bouncing back and forth, are continuously ionizing the target rear face, creating plasma and huge electromagnetic pulses (EMPs)[Reference Hamster, Sullivan, Gordon, White and Falcone13–Reference Poyé, Dubois, Lubrano-Lavaderci, d’Humières, Bardon, Hulin, Bailly-Grandvaux, Ribolzi, Raffestin, Santos, Nicolaï and Tikhonchuk15], up to some TV/m, depending on the laser pulse intensity. The generated EMP spectrum is composed of two main elements: one consisting in ultra-short THz pulses[Reference Gopal, Herzer, Schmidt, Singh, Reinhard, Ziegler, Brömmel, Karmakar, Gibbon, Dillner, May, Meyer and Paulus16, Reference Jin, Zhuo, Nakazawa, Shin, Wakamatsu, Yugami, Hosokai, Zou, Yu, Sheng and Kodama17], related to the fast electrons’ induced current, whose propagation, measured through coil-like structures[Reference Kar, Ahmed, Prasad, Cerchez, Brauckmann, Aurand, Cantono, Hadjisolomou, Lewis, Macchi, Nersisyan, Robinson, Schroer, Swantusch, Zepf, Willi and Borghesi18] or wires[Reference Quinn, Wilson, Cecchetti, Ramakrishna, Romagnani, Sarri, Lancia, Fuchs, Pipahl, Toncian, Willi, Clarke, Neely, Notley, Gallegos, Carroll, Quinn, Yuan, McKenna, Liseykina, Macchi and Borghesi19, Reference Tokita, Sakabe, Nagashima, Hashida and Inoue20], occurs on a tens of picoseconds timescale; the other, in the GHz range, with nanosecond duration, is due to neutralization currents flowing through the target to balance its induced positive charge[Reference Brown, Throop, Eder and Kimbrough21–Reference Consoli, De Angelis, Duvillaret, Andreoli, Cipriani, Cristofari, Di Giorgio, Ingenito and Verona23]. A complete picture of the several processes happening in laser–target interactions is experimentally complicated to realize, since different diagnostic techniques are usually needed. The detection of the ejected fast electrons followed by the emission of ultra-short EMPs, in particular, has not been experimentally provided until now. The major obstacle relies on obtaining data that accurately determine the electric fields and the modifications in the transient interaction process evolving from the sub-picosecond scale up to several picoseconds[Reference Dubois, Lubrano-Lavaderci, Raffestin, Ribolzi, Gazave, Compant La Fontaine, d’Humières, Hulin, Nicolaï, Poyé and Tikhonchuk12]. So far, experimental evidence has been obtained with high-resolution measurements of the plasma density near the target surface[Reference Jäckel, Polz, Pfotenhauer, Schlenvoigt, Schwoerer and Kaluza24], probing the quasi-static magnetic fields in plasmas[Reference Sandhu, Dharmadhikari, Rajeev, Kumar, Sengupta, Das and Kaw25, Reference Chatterjee, Singh, Adak, Lad and Kumar26] or the emitted electric pulses[Reference Jin, Zhuo, Nakazawa, Shin, Wakamatsu, Yugami, Hosokai, Zou, Yu, Sheng and Kodama17, Reference Zhuo, Zhang, Li, Zhou, Li, Zou, Yu, Wu, Sheng and Zhou27]. In the following, we provide a review of the different diagnostics employed in laser–target interaction experiments related to electron, proton and ion acceleration. Finally, we present our recently developed non-destructive, single-shot, temporally resolved diagnostic[Reference Pompili, Anania, Bisesto, Botton, Castellano, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler28–Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31] based on electro-optical sampling (EOS)[Reference Wilke, MacLeod, Gillespie, Berden, Knippels and Van Der Meer32], able to record with femtosecond resolution ultra-short EMPs and fast electrons emitted from solid targets during the interaction, previously adopted just in accelerator physics. Our diagnostic is able to provide a more detailed picture of this phenomenon at the sub-picosecond timescale, highlighting its ultra-fast dynamics. The analysis is expected to be significant in further understanding and developing future high-energy particle accelerators and radiation sources.
$\unicode[STIX]{x03BC}\text{m}$
radius), are produced. The conventional understanding of the underlying process is laser heating of the target’s electrons, thus producing a ‘hot’ electron cloud at the vicinity of its back surface. Some of them are energetic enough to escape and a positive unbalanced charge is left on the target. This process is responsible for building up an electrostatic potential, which in turn governs the ion acceleration[Reference Krygier, Schumacher and Freeman9, Reference Macchi, Borghesi and Passoni10], occurring typically in a sub-picosecond timescale. During the potential setup, the electronic cloud locked near the target, within a distance comparable to the Debye length[Reference Badziak, Głowacz, Jabłoński, Parys, Wołowski, Hora, Krása, Láska and Rohlena11], is thermalizing and some energetic electrons are still escaping from the target. Finally, this process ends when electrons cannot overcome the electrostatic potential induced near the target surface, and a second slower expansion–relaxation process takes over[Reference Dubois, Lubrano-Lavaderci, Raffestin, Ribolzi, Gazave, Compant La Fontaine, d’Humières, Hulin, Nicolaï, Poyé and Tikhonchuk12]. For sub-picosecond laser pulse irradiation, target charge neutralization by the electrons coming from outer-target, darkened sections can be neglected: the amount of escaped energetic electrons corresponds to the net positive charge left on the target surface[Reference Dubois, Lubrano-Lavaderci, Raffestin, Ribolzi, Gazave, Compant La Fontaine, d’Humières, Hulin, Nicolaï, Poyé and Tikhonchuk12]. The subsequent cooling process, including multiple collisions with the surrounding ions, sets the upper limit of the time range of target charging (that is, the effective lifetime of the potential barrier). Moreover, the locked hot electrons, bouncing back and forth, are continuously ionizing the target rear face, creating plasma and huge electromagnetic pulses (EMPs)[Reference Hamster, Sullivan, Gordon, White and Falcone13–Reference Poyé, Dubois, Lubrano-Lavaderci, d’Humières, Bardon, Hulin, Bailly-Grandvaux, Ribolzi, Raffestin, Santos, Nicolaï and Tikhonchuk15], up to some TV/m, depending on the laser pulse intensity. The generated EMP spectrum is composed of two main elements: one consisting in ultra-short THz pulses[Reference Gopal, Herzer, Schmidt, Singh, Reinhard, Ziegler, Brömmel, Karmakar, Gibbon, Dillner, May, Meyer and Paulus16, Reference Jin, Zhuo, Nakazawa, Shin, Wakamatsu, Yugami, Hosokai, Zou, Yu, Sheng and Kodama17], related to the fast electrons’ induced current, whose propagation, measured through coil-like structures[Reference Kar, Ahmed, Prasad, Cerchez, Brauckmann, Aurand, Cantono, Hadjisolomou, Lewis, Macchi, Nersisyan, Robinson, Schroer, Swantusch, Zepf, Willi and Borghesi18] or wires[Reference Quinn, Wilson, Cecchetti, Ramakrishna, Romagnani, Sarri, Lancia, Fuchs, Pipahl, Toncian, Willi, Clarke, Neely, Notley, Gallegos, Carroll, Quinn, Yuan, McKenna, Liseykina, Macchi and Borghesi19, Reference Tokita, Sakabe, Nagashima, Hashida and Inoue20], occurs on a tens of picoseconds timescale; the other, in the GHz range, with nanosecond duration, is due to neutralization currents flowing through the target to balance its induced positive charge[Reference Brown, Throop, Eder and Kimbrough21–Reference Consoli, De Angelis, Duvillaret, Andreoli, Cipriani, Cristofari, Di Giorgio, Ingenito and Verona23]. A complete picture of the several processes happening in laser–target interactions is experimentally complicated to realize, since different diagnostic techniques are usually needed. The detection of the ejected fast electrons followed by the emission of ultra-short EMPs, in particular, has not been experimentally provided until now. The major obstacle relies on obtaining data that accurately determine the electric fields and the modifications in the transient interaction process evolving from the sub-picosecond scale up to several picoseconds[Reference Dubois, Lubrano-Lavaderci, Raffestin, Ribolzi, Gazave, Compant La Fontaine, d’Humières, Hulin, Nicolaï, Poyé and Tikhonchuk12]. So far, experimental evidence has been obtained with high-resolution measurements of the plasma density near the target surface[Reference Jäckel, Polz, Pfotenhauer, Schlenvoigt, Schwoerer and Kaluza24], probing the quasi-static magnetic fields in plasmas[Reference Sandhu, Dharmadhikari, Rajeev, Kumar, Sengupta, Das and Kaw25, Reference Chatterjee, Singh, Adak, Lad and Kumar26] or the emitted electric pulses[Reference Jin, Zhuo, Nakazawa, Shin, Wakamatsu, Yugami, Hosokai, Zou, Yu, Sheng and Kodama17, Reference Zhuo, Zhang, Li, Zhou, Li, Zou, Yu, Wu, Sheng and Zhou27]. In the following, we provide a review of the different diagnostics employed in laser–target interaction experiments related to electron, proton and ion acceleration. Finally, we present our recently developed non-destructive, single-shot, temporally resolved diagnostic[Reference Pompili, Anania, Bisesto, Botton, Castellano, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler28–Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31] based on electro-optical sampling (EOS)[Reference Wilke, MacLeod, Gillespie, Berden, Knippels and Van Der Meer32], able to record with femtosecond resolution ultra-short EMPs and fast electrons emitted from solid targets during the interaction, previously adopted just in accelerator physics. Our diagnostic is able to provide a more detailed picture of this phenomenon at the sub-picosecond timescale, highlighting its ultra-fast dynamics. The analysis is expected to be significant in further understanding and developing future high-energy particle accelerators and radiation sources.
2 Detection techniques implemented in TNSA experiments
As introduced before, a complete picture of the TNSA is experimentally complicated to realize. Nevertheless, several pieces of experimental evidence were obtained. In this section, we will review the most important diagnostics employed to detect electrons, EMPs and ions/protons produced during such kinds of interaction.
2.1 Plasma density measurements
The first type of measurement was presented in Refs. [Reference Jäckel, Polz, Pfotenhauer, Schlenvoigt, Schwoerer and Kaluza24]: an all-optical diagnostic method, based on interferometry, was employed to directly measure the evolution of the hot-electron distribution driving the ion beam acceleration in the TNSA regime, adopting thin foils as targets, as shown in Figure 1. The available parameters retrieved, determining the subsequent ion acceleration, are the hot-electron density near the surface, with the temperature distribution and the conversion efficiency from laser pulse energy into hot electrons.

Figure 1. Electron density interferometric measurement. (a) Schematic overview of the experimental diagnostic setup. (b) Temporal evolution of the electron density at the rear surface of the target. All pictures are shifted +200 fs due to the logarithmic timescale. Figure from Ref. [Reference Jäckel, Polz, Pfotenhauer, Schlenvoigt, Schwoerer and Kaluza24].
2.2 Probing the quasi-static magnetic fields in plasma
The second type of measurement was presented in Refs. [Reference Sandhu, Dharmadhikari, Rajeev, Kumar, Sengupta, Das and Kaw25, Reference Chatterjee, Singh, Adak, Lad and Kumar26]: they detected ultra-short (picosecond regime), multi-megagauss magnetic pulses generated in a TNSA process. This diagnostic, shown in Figures 2(a) and 2(b), consisted in a pump-and-probe technique providing a complete (that is, temporal and spatial) map of these fields. The probe pulse, normally incident on target, undergoes a polarization state change induced by the magnetic field in the plasma; large and easily measurable ellipticity changes were noticed. The field evolution was obtained with high resolution both temporally (order of the pulse length) and spatially (few
![]() $\unicode[STIX]{x03BC}\text{m}$
). Instead of probing the “dc” magnetic fields, similar measurements can be performed for the emitted electric field, as shown by Ref. [Reference Jin, Zhuo, Nakazawa, Shin, Wakamatsu, Yugami, Hosokai, Zou, Yu, Sheng and Kodama17]. Figure 3 shows how intense electric radiation in the THz regime is recorded behind a thin target interacting with an ultra-short (high-contrast) laser pulse. The efficient THz emission can be attributed to the hot-electron beam acceleration by the target sheath E-field. In Ref. [Reference Jin, Zhuo, Nakazawa, Shin, Wakamatsu, Yugami, Hosokai, Zou, Yu, Sheng and Kodama17] a single-shot THz time-domain spectroscopy system (TDS) with direct spatial encoding pump–probe EOS[Reference Kim, Yellampalle, Taylor, Rodriguez and Glownia33] (providing a
$\unicode[STIX]{x03BC}\text{m}$
). Instead of probing the “dc” magnetic fields, similar measurements can be performed for the emitted electric field, as shown by Ref. [Reference Jin, Zhuo, Nakazawa, Shin, Wakamatsu, Yugami, Hosokai, Zou, Yu, Sheng and Kodama17]. Figure 3 shows how intense electric radiation in the THz regime is recorded behind a thin target interacting with an ultra-short (high-contrast) laser pulse. The efficient THz emission can be attributed to the hot-electron beam acceleration by the target sheath E-field. In Ref. [Reference Jin, Zhuo, Nakazawa, Shin, Wakamatsu, Yugami, Hosokai, Zou, Yu, Sheng and Kodama17] a single-shot THz time-domain spectroscopy system (TDS) with direct spatial encoding pump–probe EOS[Reference Kim, Yellampalle, Taylor, Rodriguez and Glownia33] (providing a
![]() ${\sim}10~\text{ps}$
time window with around 25 fs temporal resolution) was studied. Its simplicity and robustness made the technique ideal for real-time relativistic electron beam characterization. In addition, they used a Golay cell, coupled with a polyethylene polarizer, in measuring its energy and polarization, to calibrate the peak THz field on the EO crystal.
${\sim}10~\text{ps}$
time window with around 25 fs temporal resolution) was studied. Its simplicity and robustness made the technique ideal for real-time relativistic electron beam characterization. In addition, they used a Golay cell, coupled with a polyethylene polarizer, in measuring its energy and polarization, to calibrate the peak THz field on the EO crystal.

Figure 2. Magnetic pulse measurement. Schematic of the pump–probe experimental diagnostic setup for probing the megagauss magnetic fields at the (a) front and (b) rear target surfaces. (c) Magnetic field pulse profile for p- and s-polarized pump lasers with an intensity of
![]() $1.1\times 10^{16}~\text{W}/\text{cm}^{2}$
. Solid line shows the fit for the p-polarized case using a phenomenological model. The inset shows the reflectivity and induced ellipticity of the probe as a function of delay time. Figure from Ref. [Reference Sandhu, Dharmadhikari, Rajeev, Kumar, Sengupta, Das and Kaw25].
$1.1\times 10^{16}~\text{W}/\text{cm}^{2}$
. Solid line shows the fit for the p-polarized case using a phenomenological model. The inset shows the reflectivity and induced ellipticity of the probe as a function of delay time. Figure from Ref. [Reference Sandhu, Dharmadhikari, Rajeev, Kumar, Sengupta, Das and Kaw25].

Figure 3. THz pulse detection. (a) Experimental setup. The THz radiation emitted from the target rear surface is collected and sent to a calibrated THz energy meter or to a single-shot TDS system with a dual reflective echelon pair. The beamlets produced by the echelon pair arrive at the ZnTe with different time delays. Via the electro-optic effect induced by the THz electric field, the temporal evolution of the THz field is retrieved. (b) Experimentally detected THz time-domain signals and (c) corresponding spectra from Cu targets with different thicknesses. The laser energy is 600 mJ. The blue dashed line gives the calculated sheath lifetime. Figure from Ref. [Reference Jin, Zhuo, Nakazawa, Shin, Wakamatsu, Yugami, Hosokai, Zou, Yu, Sheng and Kodama17].
2.3 Electromagnetic pulse detection
A target irradiated with a high-power laser pulse blows off a large amount of charge and, in turn, the target itself emits EMPs, owing to the high return current flowing to the ground through the target holder[Reference De Marco, Krása, Cikhardt, Pfeifer, Krouskỳ, Margarone, Ahmed, Borghesi, Kar, Giuffrida, Vrana, Velyhan, Limpouch, Korn, Weber, Velardi, Delle Side, Nassisi and Ullschmied34]. Generally, the interaction chamber can be seen as a resonant cavity in which different modes of EMP oscillate for hundreds of nanoseconds, until the EMP is transmitted outside – for example, through the chamber glass windows – and EM waves are attenuated. However, due to the effects of small-scale structures in the target chamber, as well as of equipment inside the chamber, such as the motorized stages, cameras and other detectors, there is a shift in the ideal chamber resonant frequency[Reference De Marco, Pfeifer, Krousky, Krasa, Cikhardt, Klir and Nassisi35]. The basic EMP diagnostic tool is represented by the Moebius loop antenna, typically made from solid coaxial copper cable, coupled with an electronic oscilloscope, able to measure a wide range of frequencies, up to the GHz level. As shown in Figure 4, a twist in the loop is present, which doubles the effective loop area and makes it insensitive to charged particles and ionizing radiation[Reference Duncan36]. The two outputs are added using a matching unit to give a signal proportional to the rate of change of magnetic field. Apart from this kind of conductive antenna, recently also a dielectric electro-optic-based probe has been employed for EMP detection in an experiment with a nanosecond-long laser[Reference Consoli, De Angelis, Duvillaret, Andreoli, Cipriani, Cristofari, Di Giorgio, Ingenito and Verona23]. In particular, measurements of external electric fields have been performed by detecting the change of polarization state, induced by the electro-optic effect, of a continuous-wave laser probe beam with
![]() $\unicode[STIX]{x1D706}_{p}=1550~\text{nm}$
and circular polarization, propagating in a
$\unicode[STIX]{x1D706}_{p}=1550~\text{nm}$
and circular polarization, propagating in a
![]() ${<}111>$
-cut
${<}111>$
-cut
![]() $\text{Bi}_{12}\text{SiO}_{20}$
(BSO) 5 mm thick crystal.
$\text{Bi}_{12}\text{SiO}_{20}$
(BSO) 5 mm thick crystal.

Figure 4. Moebius loop antenna layout. Figure from Ref. [Reference Mead, Neely, Gauoin, Heathcote and Patel14].
2.4 Escaping particle tracking
Other types of measurement presented here regard tracking of the escaping particles: electrons, protons, ions and photons.
2.4.1 Magnetic spectrometer
The first measurements of electrons produced by the interaction between an ultra-short, high-intensity laser and a solid target were presented in 1996 by Ref. [Reference Malka and Miquel37]. The implemented diagnostic, shown in Figure 5(a), was a simple charged particle energy spectrometer, working in the range 0.4–3 MeV, composed of a pair of permanent magnets producing a uniform magnetic field
![]() $(B=1700~\text{G})$
, installed along two different directions,
$(B=1700~\text{G})$
, installed along two different directions,
![]() $0^{\circ }$
and
$0^{\circ }$
and
![]() $22^{\circ }$
, respectively. In this way, the electron energy spectrum was detected for different laser intensities, as reported in Figure 5(b).
$22^{\circ }$
, respectively. In this way, the electron energy spectrum was detected for different laser intensities, as reported in Figure 5(b).
The use of a dipole spectrometer allows easy detection of charged particles and can resolve their energy spectrum. Nevertheless, there are some drawbacks: in absence of a collimator, the divergence and transverse beam size can affect this measurement; at the same time, the X-rays and
![]() $\unicode[STIX]{x03B3}$
-rays produce a background on the scintillator used to track the bent particles, which can be a source of systematic error.
$\unicode[STIX]{x03B3}$
-rays produce a background on the scintillator used to track the bent particles, which can be a source of systematic error.

Figure 5. Electron energy spectrum measurement. (a) Experimental setup. The magnetic spectrometer
![]() $(B=1700~\text{G})$
is used to measure the energy of electrons ejected from a
$(B=1700~\text{G})$
is used to measure the energy of electrons ejected from a
![]() $30~\unicode[STIX]{x03BC}\text{m}$
thick CH target at different observation angles (0 and 22 deg). (b) Electron distributions from 0.4 to 3 MeV, for
$30~\unicode[STIX]{x03BC}\text{m}$
thick CH target at different observation angles (0 and 22 deg). (b) Electron distributions from 0.4 to 3 MeV, for
![]() $9\times 10^{18}~\text{W}/\text{cm}^{2}$
,
$9\times 10^{18}~\text{W}/\text{cm}^{2}$
,
![]() $3\times 10^{18}~\text{W}/\text{cm}^{2}$
, and
$3\times 10^{18}~\text{W}/\text{cm}^{2}$
, and
![]() $2\times 10^{18}~\text{W}/\text{cm}^{2}$
laser intensities, at normal incidence, measured along the laser propagation axis. Hot temperatures are, respectively, 891, 420, and 374 keV, assuming a Boltzmann distribution (solid lines). The vertical axis is the number of electrons per units of keV and steradians. The horizontal error bar takes into account the spatial extension of the diodes. Figure from Ref. [Reference Malka and Miquel37].
$2\times 10^{18}~\text{W}/\text{cm}^{2}$
laser intensities, at normal incidence, measured along the laser propagation axis. Hot temperatures are, respectively, 891, 420, and 374 keV, assuming a Boltzmann distribution (solid lines). The vertical axis is the number of electrons per units of keV and steradians. The horizontal error bar takes into account the spatial extension of the diodes. Figure from Ref. [Reference Malka and Miquel37].
2.4.2 Faraday cup
In a laser–target environment, the Faraday cup can be adopted to detect high-energy charged particles as a real-time detector based on the time-of-flight (TOF) technique. The TOF spectrum results from a time-resolved voltage amplitude measurement, induced by charged particles impinging the Faraday cup.
Faraday cup arrays are designed to cover different observation angles, with the normal direction to the target as reference, as shown in Figure 6(a). The diagnostic allows measurement of the two-dimensional spatial ion current density distribution and the ability to show its time evolution through TOF measurements, which is not achievable with a standard diagnostic such as laser optical interferometry. This is a unique method for two-dimensional measurements of ion currents from laser-generated plasmas, as shown in Figure 6(b).
As mentioned, the detector is used as an ion collector in an array covering the entire emission cone and is capable of detecting high currents when it is placed in a range of few tens of centimeters from the interaction point. In common with other diagnostics, the main disadvantage is that, because of the detector position close to the interaction point, large EMPs exist and can disturb the measurements if not accurately identified and de-embedded.
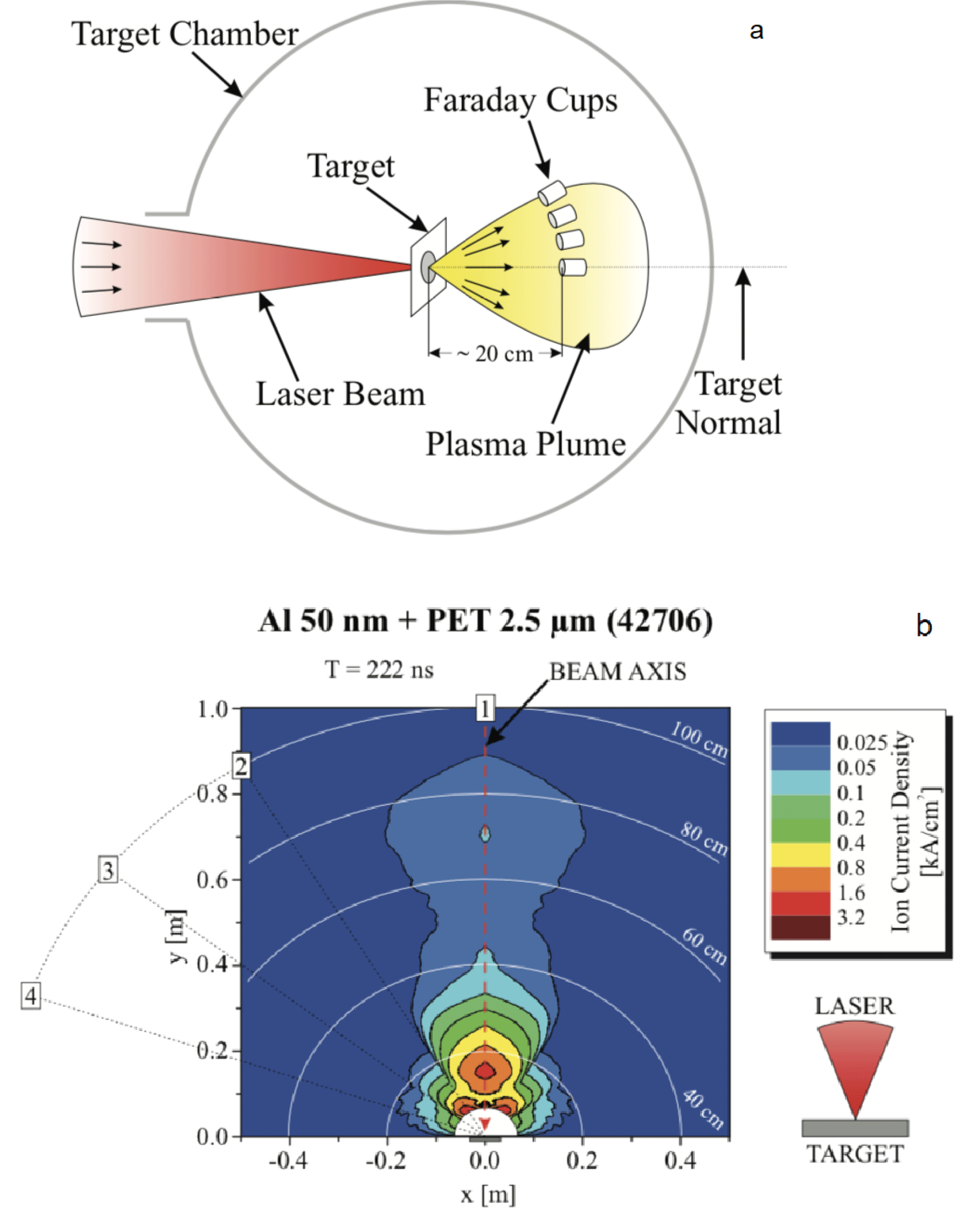
Figure 6. (a) Sketch of the experimental setup at the PHELIX sub-picosecond laser facility. (b) Spatial distribution of ion current density using a double-layer target with Al on the front side and PET on the rear side irradiated with 516 J of laser energy.
2.4.3 Radiochromic film
Measurements of ion species parameters, such as divergence, emittance, spatial and energy resolved distribution, are of primary interest. During the interaction between powerful laser systems and targets, significant EMPs can be generated. Because of these, sensitive electronics close to the interaction point is not reliable; in contrast, film detection has been demonstrated not to be sensitive to EMPs, but electrons and X-rays can cause a signal background (easily identifiable).
The simplest setup[Reference Mackinnon, Borghesi, Hatchett, Key, Patel, Campbell, Schiavi, Snavely, Wilks and Willi38] consists in employing radiochromic film (RCF) layers acting as an energy filter. In general, particles penetrating one or more layers of the film detector deposit energy, thus the two-dimensional particle distribution can be obtained. Moreover, each film records the deposited energy, so an energy-resolved measurement (referred to as radiochromic film imaging spectroscopy) is possible. Low-energy protons are stopped in the initial film layers, whereas the most energetic penetrate and are stopped successively in the stack, as shown in Figure 7. The energy and spatially resolved proton distribution obtained from the film stack complete the beam reconstruction. Together with RCF, the use of activated materials for proton imaging has been attempted to observe global beam structures, showing positive results[Reference McKenna, Ledingham and Robson39]. Moreover, Ref. [Reference Clarke, Simpson, Kar, Green, Bellei, Carroll, Dromey, Kneip, Markey, McKenna, Murphy, Nagel, Willingale and Zepf40] used silver and copper foils for energy filtering within an RCF stack. Silver foils were used to reduce the observed activity in order to minimize the exposure to the radioactive material. On the other hand, copper foils enabled absolute proton flux measurements, exploiting the
![]() $\text{}^{63}\text{Cu}~(\text{p},\text{n})~\text{}^{63}\text{Zn}$
reaction, decaying through
$\text{}^{63}\text{Cu}~(\text{p},\text{n})~\text{}^{63}\text{Zn}$
reaction, decaying through
![]() $511~\text{keV}~\unicode[STIX]{x03B2}+$
emission, and measured using NaI co-incidence detectors. The energy filtering foils were placed onto Fuji image plates and left to be exposed to the radiation emitted from the decaying isotopes. It should be noted that, although the foil and RCF within the stack are positioned together, they do not sample the same energy slice. This is due to the threshold energy for generating a (p, n) reaction, typically around 4–5 MeV. In contrast, the RCF responds directly to the incoming radiation dose.
$511~\text{keV}~\unicode[STIX]{x03B2}+$
emission, and measured using NaI co-incidence detectors. The energy filtering foils were placed onto Fuji image plates and left to be exposed to the radiation emitted from the decaying isotopes. It should be noted that, although the foil and RCF within the stack are positioned together, they do not sample the same energy slice. This is due to the threshold energy for generating a (p, n) reaction, typically around 4–5 MeV. In contrast, the RCF responds directly to the incoming radiation dose.

Figure 7. Electron bunches with different energies stopped in different films within the detector. Figure from Ref. [Reference Bolton, Borghesi, Brenner, Carroll, De Martinis, Fiorini, Flacco, Floquet, Fuchs, Gallegos, Giove, Green, Green, Jones, Kirby, McKenna, Neely, Nuesslin, Prasad, Reinhardt, Roth, Schramm, Scott, Ter-Avetisyan, Tolley, Turchetti and Wilkens41].
2.4.4 Imaging plates
Other diagnostic techniques widely adopted to determine the beam energy and profile are image plates (IPs) and scintillators, respectively. The IP is made of phosphors with phosphorescent properties which can release the stored energy in a de-excitation process. When photons or charged particles are incident on the IP, the electrons are promoted to a meta-stable state. Therefore, the energy stored can be retrieved by stimulating the excited meta-stable state by photons, and then the released energy (as light) is called photo-stimulated luminescence (PSL)[Reference Schwoerer, Pfotenhauer, Jäckel, Amthor, Liesfeld, Ziegler, Sauerbrey, Ledingham and Esirkepov42]. On the other hand, a scintillator-based diagnostic can spatially resolve the transverse beam profile over a number of specified energy ranges. Multiple sheets of organic scintillators emitting light over different wavelengths can be placed in the beam path: the lowest-energy ions are stopped in the first sheet, while higher energies are stopped in subsequent sheets. As shown in Figure 8, the collected optical signal is imaged with a gated, intensified CCD camera recording the transverse beam profiles for two or more beam energy ranges[Reference Schwoerer, Pfotenhauer, Jäckel, Amthor, Liesfeld, Ziegler, Sauerbrey, Ledingham and Esirkepov42]. IPs are sensitive to laser light and, therefore, should be properly protected to avoid signal losses. A simple strategy is to cover them with a thin aluminum foil stopping the laser.
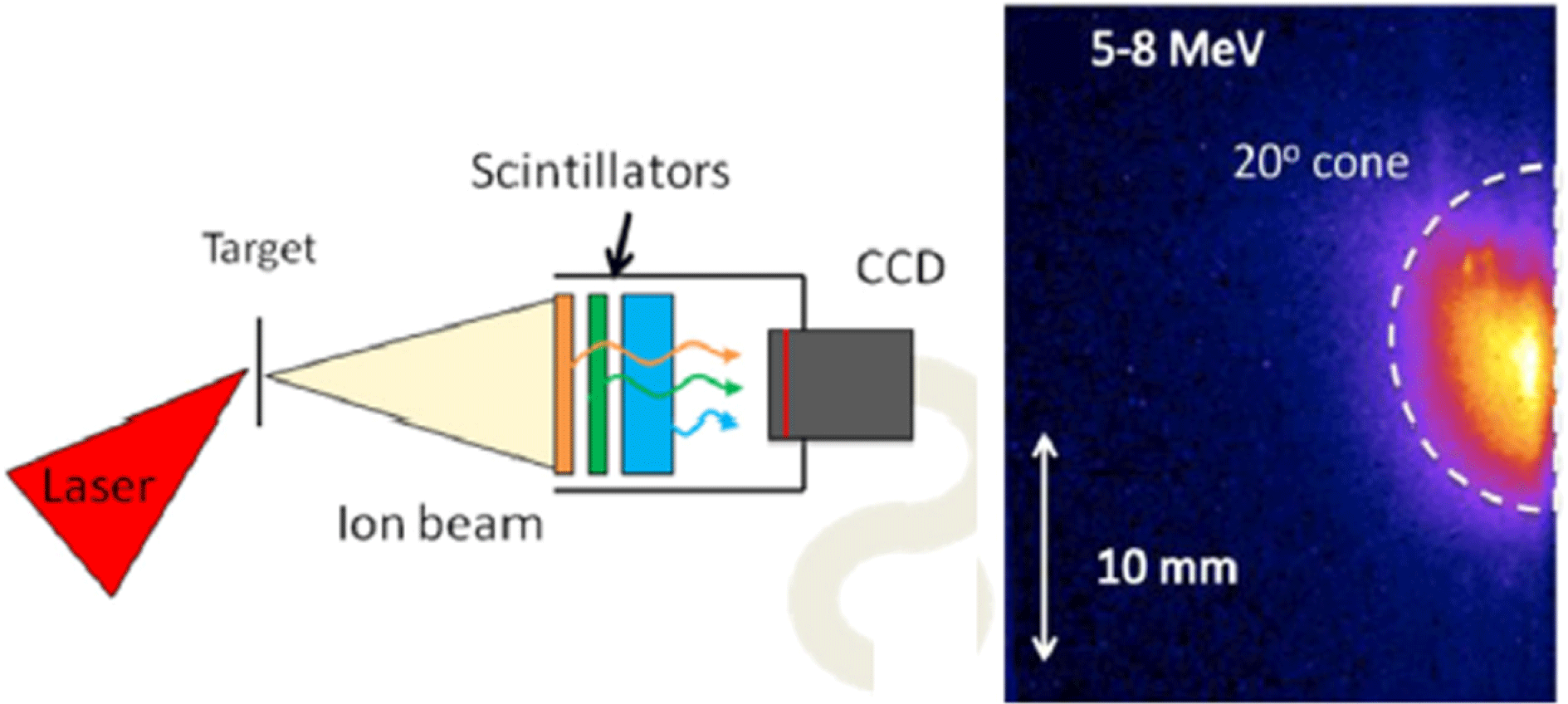
Figure 8. (Left) Schematic for a three-color proton beam spatial profiler. Higher-energy protons are stopped in the shorter-wavelength scintillators located further downstream in the stack. The combined optical signal is collected and relayed to a CCD camera via a fiber optic bundle. (Right) Proton (half) beam profile for a 100 nm Al target irradiated at
![]() $5\times 10^{20}~\text{W}/\text{cm}^{2}$
with high contrast (
$5\times 10^{20}~\text{W}/\text{cm}^{2}$
with high contrast (
![]() ${>}10^{9}$
).
${>}10^{9}$
).
2.4.5 Thompson parabola
Another diagnostic, based on collection of the escaping particles, employing both magnetic and electric fields to induce a deflection on their propagation, is called the Thompson parabola[Reference Harres, Schollmeier, Brambrink, Audebert, Blažević, Flippo, Gautier, Geißel, Hegelich, Nürnberg, Schreiber, Wahl and Roth43–Reference Alejo, Gwynne, Doria, Ahmed, Carroll, Clarke, Neely, Scott, Borghesi and Kar47]. As schematically shown in Figure 9, this diagnostic consists of an entrance pinhole, parallel electric
![]() $(E)$
and magnetic
$(E)$
and magnetic
![]() $(B)$
fields and a detector. The working principle is the following: ions with the same charge-to-mass ratio
$(B)$
fields and a detector. The working principle is the following: ions with the same charge-to-mass ratio
![]() $(Z/M)$
will trace the same parabola in the detection plane, while the position of the particles along the trace depends on their energy; higher-energy ones result closer to the so-called zero point. This is an origin point where the line joining the source and the spectrometer’s entrance intercepts the detector plane and where neutral particles and photons coming from the source would hit the detector. Although Thompson parabolas allow one to clearly identify the ion species composing the beam population, they are sensitive to EMPs. To reduce this effect, it is possible to put it outside the vacuum chamber; otherwise specific de-embedding procedures should be carried out as well as having to take electronic precautions to eliminate EMP disturbances.
$(Z/M)$
will trace the same parabola in the detection plane, while the position of the particles along the trace depends on their energy; higher-energy ones result closer to the so-called zero point. This is an origin point where the line joining the source and the spectrometer’s entrance intercepts the detector plane and where neutral particles and photons coming from the source would hit the detector. Although Thompson parabolas allow one to clearly identify the ion species composing the beam population, they are sensitive to EMPs. To reduce this effect, it is possible to put it outside the vacuum chamber; otherwise specific de-embedding procedures should be carried out as well as having to take electronic precautions to eliminate EMP disturbances.
2.4.6 Time-of-flight detector
TOF measurements are also widely employed in laser–solid matter experiments[Reference Consoli, De Angelis, Duvillaret, Andreoli, Cipriani, Cristofari, Di Giorgio, Ingenito and Verona23, Reference Margarone, Krása, Giuffrida, Picciotto, Torrisi, Nowak, Musumeci, Velyhan, Prokpek, Láska, Mocek, Ullschmied and Rus48–Reference Bisesto, Galletti, Anania, Massimo, Pompili, Botton, Zigler, Consoli, Salvadori, Andreoli and Verona52], allowing fundamental time-resolved measurements for a detailed study of ion beam parameters, such as kinetic energy and total charge, as well as shot-to-shot reproducibility[Reference Margarone, Krása, Giuffrida, Picciotto, Torrisi, Nowak, Musumeci, Velyhan, Prokpek, Láska, Mocek, Ullschmied and Rus48]. TOF spectra are usually composed by a broad ion peak signal (tens of nanoseconds at about one meter detection distance) following a narrow low-energy electrons peak and an X-ray flash. The latter, in turn, being the first to arrive on the detector, acts as a trigger for the measurement. In this scenario, semiconductor detectors are the ideal candidates. Indeed, the current response of such devices is proportional to the incident radiation energy released into the detector active layer; thus the photo-peak (usually consisting of the response to UV/soft-X-rays) is strongly lowered in comparison to the fast (high-energy) ion peak[Reference Margarone, Krása, Giuffrida, Picciotto, Torrisi, Nowak, Musumeci, Velyhan, Prokpek, Láska, Mocek, Ullschmied and Rus48].
Diamond semiconductors can be used as detectors sensitive to photons and to particles with energies above their band gap threshold, which makes them also insensitive to the visible background, which is quite noticeable in laser–plasma experiments. Nevertheless, specific designs have to be produced to eliminate EMPs coupling with the signal, as in Ref. [Reference De Angelis, Consoli, Verona, Di Giorgio, Andreoli, Cristofari, Cipriani, Ingenito, Marinelli and Verona-Rinati49]. On the other hand, the maximum detectable energy depends on the diamond thickness: the thicker the target, the higher the maximum energy is.

Figure 9. Thompson parabola. (Left) Schematic for a three-color proton beam spatial profiler. Higher-energy protons are stopped in the shorter-wavelength scintillators located further downstream in the stack. The combined optical signal is collected and relayed to a CCD camera via a fiber optic bundle. (Right) Proton (half) beam profile for a 100 nm Al target irradiated at
![]() $5\times 10^{20}~\text{W}/\text{cm}^{2}$
with high contrast (
$5\times 10^{20}~\text{W}/\text{cm}^{2}$
with high contrast (
![]() ${>}10^{9}$
).
${>}10^{9}$
).
2.4.7
 $\unicode[STIX]{x03B3}$
-ray detection
$\unicode[STIX]{x03B3}$
-ray detection
Electrons are initially accelerated during the laser–plasma interaction; subsequently, they emit hard X-rays via the Bremsstrahlung process as they are stopped in solid material. Moreover, this phenomenon regulates the electron–positron pair production
![]() $(\unicode[STIX]{x03B3}\unicode[STIX]{x03B3}\rightarrow \text{e}^{-}\text{e}^{+})$
through photon pair annihilation, enhanced by using a high-
$(\unicode[STIX]{x03B3}\unicode[STIX]{x03B3}\rightarrow \text{e}^{-}\text{e}^{+})$
through photon pair annihilation, enhanced by using a high-
![]() $Z$
material as the target (for example, gold). Such high-energy photons will produce photo-nuclear reactions in almost all materials associated with the target assembly and vacuum chamber. Reference [Reference Perry, Sefcik, Cowan, Hatchett, Hunt, Moran, Pennington, Snavely and Wilks53] designed a target in order to retrieve the Bremsstrahlung spectrum by measuring the TOF neutron energy spectrum associated with the photo-disintegration of deuterium:
$Z$
material as the target (for example, gold). Such high-energy photons will produce photo-nuclear reactions in almost all materials associated with the target assembly and vacuum chamber. Reference [Reference Perry, Sefcik, Cowan, Hatchett, Hunt, Moran, Pennington, Snavely and Wilks53] designed a target in order to retrieve the Bremsstrahlung spectrum by measuring the TOF neutron energy spectrum associated with the photo-disintegration of deuterium:
![]() $\text{D}(\unicode[STIX]{x03B3},\text{n})\text{H}(Q=2.405~\text{MeV})$
. Substantial radioactivity was measurable in the target assembly due to photo-nuclear reactions in both the gold target and the surrounding copper target holder, producing transmutation to platinum and nickel daughter isotopes. These processes are usually detected by means of thermo-luminescent dosimeters (TLDs). They can measure ionizing radiation from the intensity of visible light emitted by a crystal inside the detector when the crystal is heated. The intensity of light emitted is dependent upon the radiation exposure. The two most common types of TLDs are calcium fluoride (CaF) and lithium fluoride (LiF). The former is used to record
$\text{D}(\unicode[STIX]{x03B3},\text{n})\text{H}(Q=2.405~\text{MeV})$
. Substantial radioactivity was measurable in the target assembly due to photo-nuclear reactions in both the gold target and the surrounding copper target holder, producing transmutation to platinum and nickel daughter isotopes. These processes are usually detected by means of thermo-luminescent dosimeters (TLDs). They can measure ionizing radiation from the intensity of visible light emitted by a crystal inside the detector when the crystal is heated. The intensity of light emitted is dependent upon the radiation exposure. The two most common types of TLDs are calcium fluoride (CaF) and lithium fluoride (LiF). The former is used to record
![]() $\unicode[STIX]{x03B3}$
-ray exposure, and the latter for gamma and neutron exposure (indirectly, using the Li-6 (n,alpha) nuclear reaction).
$\unicode[STIX]{x03B3}$
-ray exposure, and the latter for gamma and neutron exposure (indirectly, using the Li-6 (n,alpha) nuclear reaction).
2.5 Other techniques
Finally, it is worth mentioning an innovative technique concerning the temporal measurement of proton bursts produced from laser–solid target interactions[Reference Dromey, Coughlan, Senje, Taylor, Kuschel, Villagomez-Bernabe, Stefanuik, Nersisyan, Stella, Kohanoff, Borghesi, Currell, Riley, Jung, Wahlström, Lewis and Zepf54], called the optical streaking technique[Reference Polli, Brida, Mukamel, Lanzani and Cerullo55], shown in Figure 10. Here, protons are accelerated via the TNSA mechanism using a
![]() $10~\unicode[STIX]{x03BC}\text{m}$
thick Au foil and collimated by means of a
$10~\unicode[STIX]{x03BC}\text{m}$
thick Au foil and collimated by means of a
![]() $100~\unicode[STIX]{x03BC}\text{m}$
Al slit (Figure 10(a)). Then, a transversely moving chirped laser pulse is used to encode the temporal profile of the proton burst: different frequency components traverse the irradiated region at different times (Figure 10(b)). Finally, the optical streak is obtained by using an imaging spectrometer (Figure 10(c)).
$100~\unicode[STIX]{x03BC}\text{m}$
Al slit (Figure 10(a)). Then, a transversely moving chirped laser pulse is used to encode the temporal profile of the proton burst: different frequency components traverse the irradiated region at different times (Figure 10(b)). Finally, the optical streak is obtained by using an imaging spectrometer (Figure 10(c)).

Figure 10. Optical streaking technique for proton beams. TNSA accelerated protons are blocked in a high-purity
![]() $\text{SiO}_{2}$
sample. The corresponding transient opacity (gray) is recorded using a synchronized chirped probe pulse. This allows one to observe the interaction over a large temporal window. In (a) and (b), the incident proton bunch is collimated using an aluminum slit (CS). Different frequency components of the chirped pulse, coming from the left, traverse the irradiated region at different times. (c) The optical streak is obtained using an imaging spectrometer. The region of interest (ROI in (d)) for the ion beam interaction is a
$\text{SiO}_{2}$
sample. The corresponding transient opacity (gray) is recorded using a synchronized chirped probe pulse. This allows one to observe the interaction over a large temporal window. In (a) and (b), the incident proton bunch is collimated using an aluminum slit (CS). Different frequency components of the chirped pulse, coming from the left, traverse the irradiated region at different times. (c) The optical streak is obtained using an imaging spectrometer. The region of interest (ROI in (d)) for the ion beam interaction is a
![]() ${\sim}10~\text{mm}$
scale slice along the central axis of laser. This is imaged onto the entrance slit of the spectrometer. Figure from Ref. [Reference Dromey, Coughlan, Senje, Taylor, Kuschel, Villagomez-Bernabe, Stefanuik, Nersisyan, Stella, Kohanoff, Borghesi, Currell, Riley, Jung, Wahlström, Lewis and Zepf54].
${\sim}10~\text{mm}$
scale slice along the central axis of laser. This is imaged onto the entrance slit of the spectrometer. Figure from Ref. [Reference Dromey, Coughlan, Senje, Taylor, Kuschel, Villagomez-Bernabe, Stefanuik, Nersisyan, Stella, Kohanoff, Borghesi, Currell, Riley, Jung, Wahlström, Lewis and Zepf54].
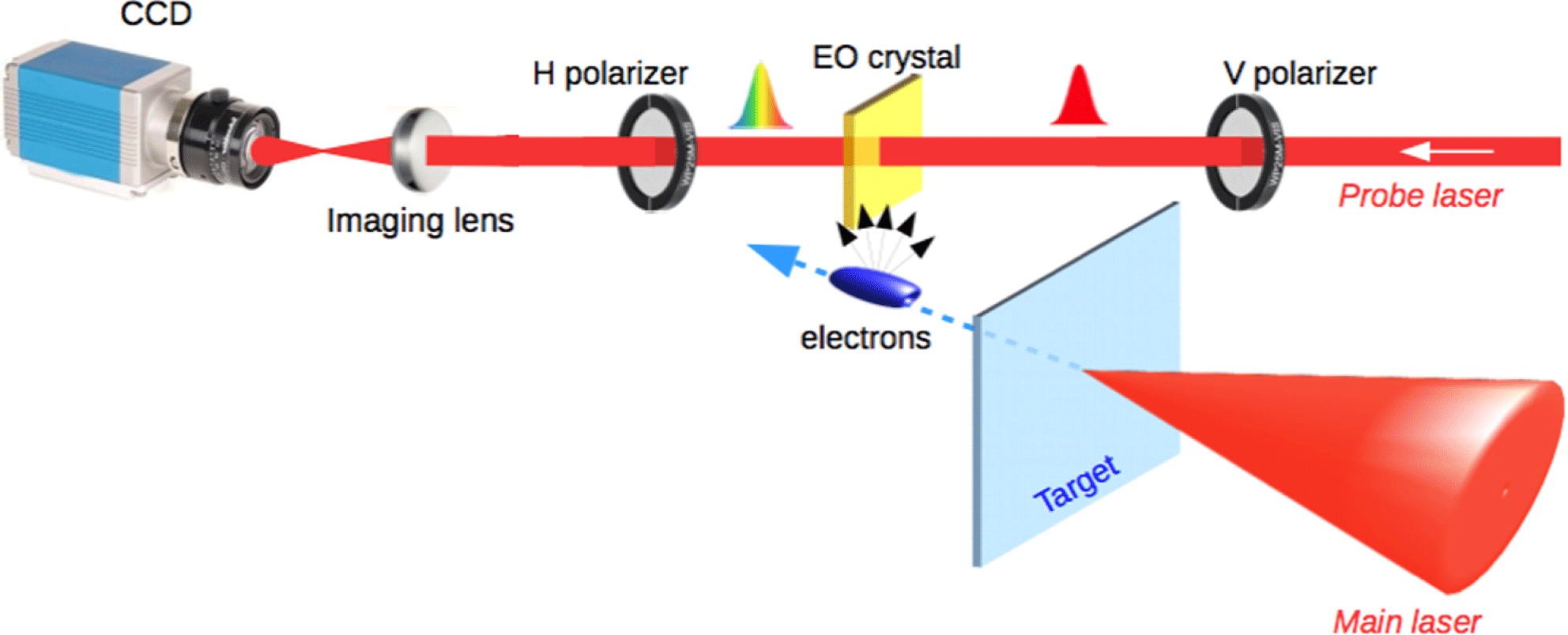
Figure 11. Sketch of the experimental setup. The FLAME laser is focused on a stainless steel target ejecting a relativistic electron beam able to escape from it as well as EMPs. These two interaction products induce local birefringence on a ZnTe electro-optic crystal, such that a linearly polarized laser, crossing the crystal, can probe them[Reference Pompili, Anania, Bisesto, Botton, Castellano, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler28].
3 EOS diagnostic for fast electron and electromagnetic pulse detection at SPARC_LAB
In laser–plasma interaction experiments, time-integrated diagnostics are usually employed, as shown in the previous section. On the other hand, single-shot, time-resolved techniques are needed to properly investigate evolution of the phenomena. At the SPARC_LAB test facility[Reference Ferrario, Alesini, Anania, Bacci, Bellaveglia, Bogdanov, Boni, Castellano, Chiadroni, Cianchi, Dabagov, De Martinis, Di Giovenale, Di Pirro, Dosselli, Drago, Esposito, Faccini, Gallo, Gambaccini, Gatti, Gatti, Ghigo, Giulietti, Ligidov, Londrillo, Lupi, Mostacci, Pace, Palumbo, Petrillo, Pompili, Rossi, Serafini, Spataro, Tomassini, Turchetti, Vaccarezza, Villa, Dattoli, Di Palma, Giannessi, Petralia, Ronsivalle, Spassovsky, Surrenti, Gizzi, Labate, Levato and Rau56], an EOS diagnostic (see Appendix A) has been installed in the FLAME laser[Reference Bisesto, Anania, Bellaveglia, Chiadroni, Cianchi, Costa, Curcio, Di Giovenale, Di Pirro, Ferrario, Filippi, Gallo, Marocchino, Pompili, Zigler and Vaccarezza57] target area to study the electron emission from solid matter targets.
Thus far, only indirect evidence of the escaping electrons has been detected. In particular, by measuring the X-ray emission by
![]() $\text{K}\unicode[STIX]{x03B1}$
spectroscopy, Bremsstrahlung radiation, interferometric measurement, and laser energy absorption[Reference Jäckel, Polz, Pfotenhauer, Schlenvoigt, Schwoerer and Kaluza24, Reference Key, Cable, Cowan, Estabrook, Hammel, Hatchett, Henry, Hinkel, Kilkenny, Koch, Kruer, Langdon, Lasinski, Lee, MacGowan, MacKinnon, Moody, Moran, Offenberger, Pennington, Perry, Phillips, Sangster, Singh, Stoyer, Tabak, Tietbohl, Tsukamoto, Wharton and Wilks58–Reference Chen, Shepherd, Chung, Kemp, Hansen, Wilks, Ping, Widmann, Fournier, Dyer, Faenov, Pikuz and Beiersdorfer62], some time-integrated hot-electron properties – for example, charge, angular distribution and energy – have been directly measured. On the other hand, our diagnostic allowed us to perform direct and temporally resolved measurements.
$\text{K}\unicode[STIX]{x03B1}$
spectroscopy, Bremsstrahlung radiation, interferometric measurement, and laser energy absorption[Reference Jäckel, Polz, Pfotenhauer, Schlenvoigt, Schwoerer and Kaluza24, Reference Key, Cable, Cowan, Estabrook, Hammel, Hatchett, Henry, Hinkel, Kilkenny, Koch, Kruer, Langdon, Lasinski, Lee, MacGowan, MacKinnon, Moody, Moran, Offenberger, Pennington, Perry, Phillips, Sangster, Singh, Stoyer, Tabak, Tietbohl, Tsukamoto, Wharton and Wilks58–Reference Chen, Shepherd, Chung, Kemp, Hansen, Wilks, Ping, Widmann, Fournier, Dyer, Faenov, Pikuz and Beiersdorfer62], some time-integrated hot-electron properties – for example, charge, angular distribution and energy – have been directly measured. On the other hand, our diagnostic allowed us to perform direct and temporally resolved measurements.
Some experimental campaigns[Reference Pompili, Anania, Bisesto, Botton, Castellano, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler28, Reference Pompili, Anania, Bisesto, Botton, Castellano, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler29, Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31] have been performed with the FLAME laser using the setup in Figure 11. The EOS diagnostic installed for this experiment relies on a
![]() $500~\unicode[STIX]{x03BC}\text{m}$
thick ZnTe crystal and, exploiting the spatial encoding technique[Reference Cavalieri, Fritz, Lee, Bucksbaum, Reis, Rudati, Mills, Fuoss, Stephenson, Kao, Siddons, Lowney, MacPhee, Weinstein, Falcone, Pahl, Als-Nielsen, Blome, Dusterer, Ischebeck, Schlarb, Schulte-Schrepping, Tschentscher, Schneider, Hignette, Sette, Sokolowski-Tinten, Chapman, Lee, Hansen, Synnergren, Larsson, Techert, Sheppard, Wark, Bergh, Caleman, Huldt, van der Spoel, Timneanu, Hajdu, Akre, Bong, Emma, Krejcik, Arthur, Brennan, Gaffney, Lindenberg, Luening and Hastings63], with the probe laser entering the crystal at an incidence angle
$500~\unicode[STIX]{x03BC}\text{m}$
thick ZnTe crystal and, exploiting the spatial encoding technique[Reference Cavalieri, Fritz, Lee, Bucksbaum, Reis, Rudati, Mills, Fuoss, Stephenson, Kao, Siddons, Lowney, MacPhee, Weinstein, Falcone, Pahl, Als-Nielsen, Blome, Dusterer, Ischebeck, Schlarb, Schulte-Schrepping, Tschentscher, Schneider, Hignette, Sette, Sokolowski-Tinten, Chapman, Lee, Hansen, Synnergren, Larsson, Techert, Sheppard, Wark, Bergh, Caleman, Huldt, van der Spoel, Timneanu, Hajdu, Akre, Bong, Emma, Krejcik, Arthur, Brennan, Gaffney, Lindenberg, Luening and Hastings63], with the probe laser entering the crystal at an incidence angle
![]() $\unicode[STIX]{x1D703}_{i}\approx 28^{\circ }$
, the temporal charge profile of the emitted electrons is encoded along the transverse profile of the probe laser. Since the probe diameter on the crystal is
$\unicode[STIX]{x1D703}_{i}\approx 28^{\circ }$
, the temporal charge profile of the emitted electrons is encoded along the transverse profile of the probe laser. Since the probe diameter on the crystal is
![]() $d_{L}\approx 6~\text{mm}$
, the time window provided by our diagnostic is
$d_{L}\approx 6~\text{mm}$
, the time window provided by our diagnostic is
![]() $\unicode[STIX]{x0394}t=(d_{L}/c)\sin \unicode[STIX]{x1D703}_{i}\approx 10~\text{ps}$
, where
$\unicode[STIX]{x0394}t=(d_{L}/c)\sin \unicode[STIX]{x1D703}_{i}\approx 10~\text{ps}$
, where
![]() $c$
is the vacuum speed of light. Moreover, in our experimental conditions, the temporal resolution is about 100 fs. Downstream of the ZnTe crystal, as shown in Figure 11, a polarizer is set to retrieve the electric field actually encoded into the ‘EOS-probe’ signal. The polarizer is rotated
$c$
is the vacuum speed of light. Moreover, in our experimental conditions, the temporal resolution is about 100 fs. Downstream of the ZnTe crystal, as shown in Figure 11, a polarizer is set to retrieve the electric field actually encoded into the ‘EOS-probe’ signal. The polarizer is rotated
![]() $90^{\circ }$
with respect to the input laser polarization – that is, there is no light transmitted in the absence of an EO-induced phase retardation. It is able to convert the probe polarization modulation into intensity modulation. The diagnostic signal can be detected simply using a CCD camera.
$90^{\circ }$
with respect to the input laser polarization – that is, there is no light transmitted in the absence of an EO-induced phase retardation. It is able to convert the probe polarization modulation into intensity modulation. The diagnostic signal can be detected simply using a CCD camera.
Moreover, our EOS diagnostic is also suitable to work as a TOF monitor[Reference Cavalieri, Fritz, Lee, Bucksbaum, Reis, Rudati, Mills, Fuoss, Stephenson, Kao, Siddons, Lowney, MacPhee, Weinstein, Falcone, Pahl, Als-Nielsen, Blome, Dusterer, Ischebeck, Schlarb, Schulte-Schrepping, Tschentscher, Schneider, Hignette, Sette, Sokolowski-Tinten, Chapman, Lee, Hansen, Synnergren, Larsson, Techert, Sheppard, Wark, Bergh, Caleman, Huldt, van der Spoel, Timneanu, Hajdu, Akre, Bong, Emma, Krejcik, Arthur, Brennan, Gaffney, Lindenberg, Luening and Hastings63, Reference Pompili, Anania, Bellaveglia, Biagioni, Castorina, Chiadroni, Cianchi, Croia, Di Giovenale, Ferrario, Filippi, Gallo, Gatti, Giorgianni, Giribono, Li, Lupi, Mostacci, Petrarca, Piersanti, Di Pirro, Romeo, Scifo, Shpakov, Vaccarezza and Villa64]. Indeed, by measuring the relative delay with respect to the reference time, the particle TOF being
![]() $\unicode[STIX]{x0394}t_{\text{TOF}}$
, we can calculate the bunch velocity as
$\unicode[STIX]{x0394}t_{\text{TOF}}$
, we can calculate the bunch velocity as
![]() $v=d/\unicode[STIX]{x0394}t_{\text{TOF}}$
and its energy as
$v=d/\unicode[STIX]{x0394}t_{\text{TOF}}$
and its energy as
![]() $E=\unicode[STIX]{x1D6FE}m_{e}$
, where
$E=\unicode[STIX]{x1D6FE}m_{e}$
, where
![]() $\unicode[STIX]{x1D6FE}=1/\sqrt{1-(v/c)^{2}}$
is the relativistic Lorentz factor,
$\unicode[STIX]{x1D6FE}=1/\sqrt{1-(v/c)^{2}}$
is the relativistic Lorentz factor,
![]() $c$
is the speed of light and
$c$
is the speed of light and
![]() $m_{e}$
is the electron rest mass in eV units. Unlike conventional time-integrated techniques (for example, magnetic spectrometer), this method is able to provide energy measurements resolved in time (see Figure 12). In addition, the electric field generated by the bunch, inducing the electro-optic effect, is proportional to its charge. Therefore, the latter can be estimated from the signal intensity, making our EOS diagnostic act as a temporally resolved charge measurement with femtosecond resolution.
$m_{e}$
is the electron rest mass in eV units. Unlike conventional time-integrated techniques (for example, magnetic spectrometer), this method is able to provide energy measurements resolved in time (see Figure 12). In addition, the electric field generated by the bunch, inducing the electro-optic effect, is proportional to its charge. Therefore, the latter can be estimated from the signal intensity, making our EOS diagnostic act as a temporally resolved charge measurement with femtosecond resolution.
To summarize, our EOS-based diagnostic allows relativistic fast electron charge and mean energy time-resolved measurements, as well as providing the temporal length of the electron bunch. To be more precise, our diagnostic can detect any external electric field whose strength is high enough to induce a birefringence in the EO crystal.
In the following, we will treat two examples of experiments performed at SPARC_LAB: one devoted to fast electron characterization, the other for EMP detection.

Figure 12. Calibration of the diagnostics relative to TOF measurements. Each point corresponds to one delay line step (3 fs).
3.1 Experimental detection of fast electrons
By means of the setup depicted in Figure 11, we were able to detect the fast electrons emitted during the interaction between the FLAME laser and a solid matter target. Indeed, since the ZnTe crystal is installed 1 mm downstream of the target, in order to avoid particles hitting the crystal, it means that, from the probe laser point of view, there is no difference whether the electron bunch has a small or large transverse radius, since the distance of the observer is larger. This distance being much larger than the Debye length
![]() ${\sim}1~\unicode[STIX]{x03BC}\text{m}$
in our experimental conditions, only the highly energetic ejected electrons escaping the potential barrier are able to reach our diagnostic. The high-resolution EOS diagnostic technique allows us to operate on the same timescale as the process, determined by the temporal duration of the main laser[Reference Poyé, Hulin, Bailly-Grandvaux, Dubois, Ribolzi, Raffestin, Bardon, Lubrano-Lavaderci, d’Humières, Santos, Nicolaï and Tikhonchuk22, Reference Fuchs, Antici, d’Humières, Lefebvre, Borghesi, Brambrink, Cecchetti, Kaluza, Malka, Manclossi, Meyroneinc, Mora, Schreiber, Toncian, Pépin and Audebert65]. Figure 13 explains the electric field encoding process: the signal detected by a CCD camera comes from the temporal and spatial overlap between the probe laser and the local induced birefringence. The EOS response is mainly affected by the bunch current (charge per unit time) and mean energy, while it is not affected by the energy spectrum, and therefore its distribution. It means that the signal shape, duration and strength are actually determined by the average energy of the whole electron distribution, because the low/high-energy tails in the spectrum represent the minority of the entire charge; that is, the electrons in that range of energies are too few to contribute to the development of the EOS signal. Previous reports regarding ion acceleration by lasers have demonstrated a significant energy enhancement of the accelerated ions when structured targets[Reference Zigler, Eisenman, Botton, Nahum, Schleifer, Baspaly, Pomerantz, Abicht, Branzel, Priebe, Steinke, Andreev, Schnuerer, Sandner, Gordon, Sprangle and Ledingham66–Reference Margarone, Klimo, Kim, Prokupek, Limpouch, Jeong, Mocek, Psikal, Kim, Proska, Nam, Stolcova, Choi, Lee, Sung, Yu and Korn68] were employed instead of conventional targets in the TNSA scheme such as thin aluminum foils. During the laser-sharp target interaction, a higher quantity of electrons escape the target, building up a stronger potential well, which subsequently accelerates ions to higher energies. Proving this conjecture needs a direct time-resolved measurement of the escaping electrons. With this aim, we have tested different target shapes: a
${\sim}1~\unicode[STIX]{x03BC}\text{m}$
in our experimental conditions, only the highly energetic ejected electrons escaping the potential barrier are able to reach our diagnostic. The high-resolution EOS diagnostic technique allows us to operate on the same timescale as the process, determined by the temporal duration of the main laser[Reference Poyé, Hulin, Bailly-Grandvaux, Dubois, Ribolzi, Raffestin, Bardon, Lubrano-Lavaderci, d’Humières, Santos, Nicolaï and Tikhonchuk22, Reference Fuchs, Antici, d’Humières, Lefebvre, Borghesi, Brambrink, Cecchetti, Kaluza, Malka, Manclossi, Meyroneinc, Mora, Schreiber, Toncian, Pépin and Audebert65]. Figure 13 explains the electric field encoding process: the signal detected by a CCD camera comes from the temporal and spatial overlap between the probe laser and the local induced birefringence. The EOS response is mainly affected by the bunch current (charge per unit time) and mean energy, while it is not affected by the energy spectrum, and therefore its distribution. It means that the signal shape, duration and strength are actually determined by the average energy of the whole electron distribution, because the low/high-energy tails in the spectrum represent the minority of the entire charge; that is, the electrons in that range of energies are too few to contribute to the development of the EOS signal. Previous reports regarding ion acceleration by lasers have demonstrated a significant energy enhancement of the accelerated ions when structured targets[Reference Zigler, Eisenman, Botton, Nahum, Schleifer, Baspaly, Pomerantz, Abicht, Branzel, Priebe, Steinke, Andreev, Schnuerer, Sandner, Gordon, Sprangle and Ledingham66–Reference Margarone, Klimo, Kim, Prokupek, Limpouch, Jeong, Mocek, Psikal, Kim, Proska, Nam, Stolcova, Choi, Lee, Sung, Yu and Korn68] were employed instead of conventional targets in the TNSA scheme such as thin aluminum foils. During the laser-sharp target interaction, a higher quantity of electrons escape the target, building up a stronger potential well, which subsequently accelerates ions to higher energies. Proving this conjecture needs a direct time-resolved measurement of the escaping electrons. With this aim, we have tested different target shapes: a
![]() $10~\unicode[STIX]{x03BC}\text{m}$
thick aluminum foil, a wedged shape of stainless steel razor blade and a tip shape of a needle. Measuring the amount of charge and energy of the escaping electrons by means of the EOS detector, as explained before, provides the required evidence for the field-enhancement conjecture, summarized in Figure 14.
$10~\unicode[STIX]{x03BC}\text{m}$
thick aluminum foil, a wedged shape of stainless steel razor blade and a tip shape of a needle. Measuring the amount of charge and energy of the escaping electrons by means of the EOS detector, as explained before, provides the required evidence for the field-enhancement conjecture, summarized in Figure 14.

Figure 13. Spatial encoding process: (a) the coulomb field, relative to the electron bunch, makes the crystal birefringent; (b) while the E-field propagates into the crystal, the local birefringence shifts downwards; (c) the probe laser crosses the crystal and its polarization is rotated: the resulting signal comes from the region where the local birefringence and the probe laser are temporally overlapped[Reference Pompili, Anania, Bisesto, Botton, Castellano, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler29].
For the planar foil target, as in Figure 14(a), a first emitted bunch with 1.2 nC charge and 7 MeV energy is followed (delayed by about 1.5 ps) by a second broadened structure carrying more particles (about 3 nC) but that is less energetic (about 1 MeV).
The signal coming from the wedged target, as in Figure 14(b), shows a similar structure: the first bunch carries a larger amount of electrons (2 nC) with the same energy (7 MeV), while the second one is strongly reduced to 0.3 nC and delayed by about 2 ps. Figure 14(c) shows data captured from the tip target: the interaction with the laser produced a much larger number of fast electrons (about 7 nC), carrying also higher energies (about 12 MeV). Direct evidence of charge and energy boosts when using sharp tips can be extrapolated. Moreover, the presence of a second smaller bunch (B2) carrying about 3 nC charge can possibly be attributed to this target shape[Reference Pompili, Anania, Bisesto, Botton, Castellano, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler28].

Figure 14. Fast electron bunches for different target shapes. Experimental measurements of the longitudinal fast electrons’ charge profile from (a) planar, (b) wedged and (c) tip targets. The emitted charges are, respectively, (a) 1.2 nC (B1) and 3 nC (B2); (b) 2 nC (B1) and 0.3 nC (B2); (c) 7 nC (B1) and 3 nC (B2). The Gaussian envelopes represent the extrapolated charge profiles of each bunch. A
![]() $10^{2}$
neutral density filter has been used in (b) and (c) to avoid saturation of the CCD camera[Reference Pompili, Anania, Bisesto, Botton, Castellano, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler28].
$10^{2}$
neutral density filter has been used in (b) and (c) to avoid saturation of the CCD camera[Reference Pompili, Anania, Bisesto, Botton, Castellano, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler28].

Figure 15. Electro-optical encoding. (Left) EO crystal (yellow square) acquires a local birefringence when the electric field (dashed arrows) reaches it. (Right) The probe laser, starting with a well-defined linear polarization, crosses the crystal while the charge propagates on target, increasing the source size[Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31].
3.2 EMP detection
The interaction between an ultra-intense laser and a solid target also produces a huge number of EMPs. Besides the possibility to detect fast electrons, our EOS diagnostic has allowed us to reveal such EMPs. Figure 15 shows the resulting encoding process as detected by the CCD camera placed at the end of probe laser path. By means of the delay line installed along the probe laser path, it is possible to reproduce the temporal evolution of the pulses emitted by the target.

Figure 16. Evolution of the EM radiation pulse emitted by the target. By focusing the main laser onto a wedged target, the probe laser is sent onto the ZnTe crystal with different delays. The horizontal axis represents the relative probe arrival time (
![]() $t$
) associated with each pixel[Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31].
$t$
) associated with each pixel[Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31].
![]() $\unicode[STIX]{x0394}t_{0}$
is the delay between the main and probe laser pulses. From Ref. [Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31].
$\unicode[STIX]{x0394}t_{0}$
is the delay between the main and probe laser pulses. From Ref. [Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31].
Figure 16 shows the temporal evolution of the pulses emitted by the target, moving the delay line installed along the probe laser path by steps of 2.5 ps. Indeed, while the probe laser is delayed with respect to the interaction laser, the resulting signal moves towards the left and its amplitude decreases[Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31]. From this timeline, the dynamics of the target charging process can be deduced[Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31]. From the horizontal size of the detected signals, the charge spreading velocity or, similarly, the velocity at which the antenna emitting area grows with time, can be retrieved. We calculate a charge spreading velocity of
![]() $(0.94\,\pm \,0.03)c$
, in agreement with some previous works[Reference Kar, Ahmed, Prasad, Cerchez, Brauckmann, Aurand, Cantono, Hadjisolomou, Lewis, Macchi, Nersisyan, Robinson, Schroer, Swantusch, Zepf, Willi and Borghesi18, Reference Quinn, Wilson, Cecchetti, Ramakrishna, Romagnani, Sarri, Lancia, Fuchs, Pipahl, Toncian, Willi, Clarke, Neely, Notley, Gallegos, Carroll, Quinn, Yuan, McKenna, Liseykina, Macchi and Borghesi19, Reference Romagnani, Fuchs, Borghesi, Antici, Audebert, Ceccherini, Cowan, Grismayer, Kar, Macchi, Mora, Pretzler, Schiavi, Toncian and Willi69], where
$(0.94\,\pm \,0.03)c$
, in agreement with some previous works[Reference Kar, Ahmed, Prasad, Cerchez, Brauckmann, Aurand, Cantono, Hadjisolomou, Lewis, Macchi, Nersisyan, Robinson, Schroer, Swantusch, Zepf, Willi and Borghesi18, Reference Quinn, Wilson, Cecchetti, Ramakrishna, Romagnani, Sarri, Lancia, Fuchs, Pipahl, Toncian, Willi, Clarke, Neely, Notley, Gallegos, Carroll, Quinn, Yuan, McKenna, Liseykina, Macchi and Borghesi19, Reference Romagnani, Fuchs, Borghesi, Antici, Audebert, Ceccherini, Cowan, Grismayer, Kar, Macchi, Mora, Pretzler, Schiavi, Toncian and Willi69], where
![]() $c$
is the light velocity in vacuum. Indeed, since a larger emitting area produces wider EM radiation, its radial extent is directly proportional to the horizontal size of the detected signal; meanwhile, the signal thickness is directly proportional to the average duration of the detected radiation, which, for the signals reported in Figure 16, is
$c$
is the light velocity in vacuum. Indeed, since a larger emitting area produces wider EM radiation, its radial extent is directly proportional to the horizontal size of the detected signal; meanwhile, the signal thickness is directly proportional to the average duration of the detected radiation, which, for the signals reported in Figure 16, is
![]() $6\pm 1~\text{ps}$
. Furthermore, its peak value of the electric field has been found equal to 0.8 MV/m on our EOS crystal, corresponding to approximately 0.6 TV/m on target, as confirmed by particle-in-cell (PIC) simulations[Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31]. In addition, this field value allows only electrons with energies higher than 3 MeV to escape, in agreement with our measurements[Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31].
$6\pm 1~\text{ps}$
. Furthermore, its peak value of the electric field has been found equal to 0.8 MV/m on our EOS crystal, corresponding to approximately 0.6 TV/m on target, as confirmed by particle-in-cell (PIC) simulations[Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31]. In addition, this field value allows only electrons with energies higher than 3 MeV to escape, in agreement with our measurements[Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31].
4 Conclusions
In conclusion, this paper provides a review regarding the different diagnostics employed in laser–solid target interaction experiments related to electron, proton and ion acceleration. Finally, we present our experimental study regarding the development of single-shot temporal diagnostics based on EOS employed for fast electron and EMP detection at the femtosecond scale. In contrast to the reviewed diagnostics, which are mostly time-integrated, our diagnostic provides single-shot, time-resolved measurements of relativistic fast electrons in terms of charge, mean energy and temporal length. In particular, nanocoulomb charged beams have been detected with multi-MeV mean energy and temporal duration
![]() ${\sim}1~\text{ps}$
FWHM. Moreover, it allowed us to observe the temporal evolution of the EMPs emitted during this kind of interaction. The fields present a temporal duration of a few picoseconds and a peak electric field amplitude
${\sim}1~\text{ps}$
FWHM. Moreover, it allowed us to observe the temporal evolution of the EMPs emitted during this kind of interaction. The fields present a temporal duration of a few picoseconds and a peak electric field amplitude
![]() ${\sim}0.6~\text{TV}/\text{m}$
on target. The values obtained are in good agreement with PIC simulations[Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31]. This innovative diagnostic enables discrimination between the signal carried by the fast electrons and the emitted wave generated by currents in the target. Our studies open the way to perform new time-resolved experiments aimed at reaching a deeper understanding of the phenomena involved in laser–matter interaction experiments.
${\sim}0.6~\text{TV}/\text{m}$
on target. The values obtained are in good agreement with PIC simulations[Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31]. This innovative diagnostic enables discrimination between the signal carried by the fast electrons and the emitted wave generated by currents in the target. Our studies open the way to perform new time-resolved experiments aimed at reaching a deeper understanding of the phenomena involved in laser–matter interaction experiments.
Appendix A. Electro-optic effect in a zinc telluride crystal

Figure 17. ZnTe refractive index ellipsoid. The (110) plane in the ZnTe crystal and its coordinate system
![]() $(x,y)$
. The crystal is isotropic, with a constant refractive index
$(x,y)$
. The crystal is isotropic, with a constant refractive index
![]() $n_{0}$
, when no external electric field is applied. An external E-field E (blue arrow), with an angle
$n_{0}$
, when no external electric field is applied. An external E-field E (blue arrow), with an angle
![]() $\unicode[STIX]{x1D6FC}$
respect to the crystallographic
$\unicode[STIX]{x1D6FC}$
respect to the crystallographic
![]() $x$
axis, induces the electro-optic effect. The refractive index ellipsoid, projected onto the (110)-plane, is sketched as an ellipse rotated by
$x$
axis, induces the electro-optic effect. The refractive index ellipsoid, projected onto the (110)-plane, is sketched as an ellipse rotated by
![]() $\unicode[STIX]{x1D6F9}(\unicode[STIX]{x1D6FC})$
, whose main axes, parallel to
$\unicode[STIX]{x1D6F9}(\unicode[STIX]{x1D6FC})$
, whose main axes, parallel to
![]() $(\mathbf{U}_{1},\mathbf{U}_{2})$
, represent the new refractive indices
$(\mathbf{U}_{1},\mathbf{U}_{2})$
, represent the new refractive indices
![]() $n_{1}$
and
$n_{1}$
and
![]() $n_{2}$
of the crystal[Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31].
$n_{2}$
of the crystal[Reference Pompili, Anania, Bisesto, Botton, Chiadroni, Cianchi, Curcio, Ferrario, Galletti, Henis, Petrarca, Schleifer and Zigler31].
The zinc telluride (ZnTe) crystal is optically isotropic, with a uniform refractive index
![]() $n_{0}$
(
$n_{0}$
(
![]() ${\approx}2.85$
for wavelength
${\approx}2.85$
for wavelength
![]() $\unicode[STIX]{x1D706}_{L}=800~\text{nm}$
) when no external E-field
$\unicode[STIX]{x1D706}_{L}=800~\text{nm}$
) when no external E-field
![]() $\mathbf{E}(t)$
is applied. Conversely, the crystal optical isotropy vanishes and, as a consequence, its permeability tensor is altered as
$\mathbf{E}(t)$
is applied. Conversely, the crystal optical isotropy vanishes and, as a consequence, its permeability tensor is altered as
![]() $\unicode[STIX]{x1D702}_{ij}=\unicode[STIX]{x1D702}_{ij}^{0}+\sum _{k}r_{ijk}E(t)_{k}$
, where
$\unicode[STIX]{x1D702}_{ij}=\unicode[STIX]{x1D702}_{ij}^{0}+\sum _{k}r_{ijk}E(t)_{k}$
, where
![]() $\unicode[STIX]{x1D702}_{ij}^{0}$
is the unperturbed term describing the optical isotropy, while
$\unicode[STIX]{x1D702}_{ij}^{0}$
is the unperturbed term describing the optical isotropy, while
![]() $r_{ijk}$
is the tensor describing the linear electro-optic effect and
$r_{ijk}$
is the tensor describing the linear electro-optic effect and
![]() $E_{k}$
the
$E_{k}$
the
![]() $k$
-components constituting the electric field
$k$
-components constituting the electric field
![]() $\mathbf{E}(t)$
.
$\mathbf{E}(t)$
.
The tensor
![]() $\unicode[STIX]{x1D702}$
is symmetric, hence
$\unicode[STIX]{x1D702}$
is symmetric, hence
![]() $r_{ijk}=r_{jik}$
[Reference Casalbuoni, Schlarb, Schmidt, Schmüser, Steffen and Winter70]. Furthermore, a ZnTe crystal cut along the (110)-plane has an higher degree of symmetry; hence it is characterized by just one independent electro-optic coefficient:
$r_{ijk}=r_{jik}$
[Reference Casalbuoni, Schlarb, Schmidt, Schmüser, Steffen and Winter70]. Furthermore, a ZnTe crystal cut along the (110)-plane has an higher degree of symmetry; hence it is characterized by just one independent electro-optic coefficient:
![]() $r_{41}=r_{52}=r_{63}\approx 4.2~\text{pm}/\text{V}$
. Figure 17 shows the refraction indices,
$r_{41}=r_{52}=r_{63}\approx 4.2~\text{pm}/\text{V}$
. Figure 17 shows the refraction indices,
![]() $n_{1}$
and
$n_{1}$
and
![]() $n_{2}$
, related to the two induced, mutually perpendicular, principal axes
$n_{2}$
, related to the two induced, mutually perpendicular, principal axes
![]() $\mathbf{U}_{1,2}$
.
$\mathbf{U}_{1,2}$
.
![]() $\unicode[STIX]{x1D6FC}$
is the angle between the crystallographic
$\unicode[STIX]{x1D6FC}$
is the angle between the crystallographic
![]() $x$
axis and the externally applied electric field; the resulting ellipse, with semi-axes
$x$
axis and the externally applied electric field; the resulting ellipse, with semi-axes
![]() $\mathbf{U}_{1,2}$
defining the induced indices of refraction, is rotated by
$\mathbf{U}_{1,2}$
defining the induced indices of refraction, is rotated by
![]() $\unicode[STIX]{x1D6F9}(\unicode[STIX]{x1D6FC})$
. Consequently, the crystal, characterized by two different refractive indices, becomes birefringent.
$\unicode[STIX]{x1D6F9}(\unicode[STIX]{x1D6FC})$
. Consequently, the crystal, characterized by two different refractive indices, becomes birefringent.
Assuming the relation
![]() $r_{41}E\ll 1/n_{0}^{2}$
(with
$r_{41}E\ll 1/n_{0}^{2}$
(with
![]() $E=|\mathbf{E}|$
), the function describing the two refractive indices can be approximated as
$E=|\mathbf{E}|$
), the function describing the two refractive indices can be approximated as
A linearly polarized laser, with
![]() $\unicode[STIX]{x1D706}_{L}$
central wavelength, crossing an electro-optically (EO) modulated ZnTe crystal of thickness
$\unicode[STIX]{x1D706}_{L}$
central wavelength, crossing an electro-optically (EO) modulated ZnTe crystal of thickness
![]() $d$
, experiences a polarization modulation. Its electric field components, projected along the induced principal axes
$d$
, experiences a polarization modulation. Its electric field components, projected along the induced principal axes
![]() $\mathbf{U}_{1,2}$
, gain a relative phase shift. The latter, presented in Equation (A2), is linearly dependent on the applied field amplitude:
$\mathbf{U}_{1,2}$
, gain a relative phase shift. The latter, presented in Equation (A2), is linearly dependent on the applied field amplitude:
According to Equation (A2), it can be seen that if the external electric field is absent the pulse is not altered by the EO crystal and it maintains its linear polarization.


















