Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-30T23:01:55.959Z Has data issue: false hasContentIssue false
  • English
  • Français

Inequalities for branching processes

Published online by Cambridge University Press:  14 July 2016

C. R. Heathcote  and
E. Seneta
E. Seneta
Affiliation:
Australian National University
Get access Rights & Permissions [Opens in a new window]

Summary

If F(s) is the probability generating function of a non-negative random variable, the nth functional iterate Fn(s) = Fn–1 (F(s)) generates the distribution of the size of the nth generation of a simple branching process. In general it is not possible to obtain explicit formulae for many quantities involving Fn(s), and this paper considers certain bounds and approximations. 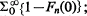Bounds are found for the Koenigs-type iterates limn→∞m−n {1−Fn(s)}, 0 ≦ s ≦ 1 where m = F′(1) < 1 and F′′(1) < ∞; for the expected time to extinction and for the limiting conditional-distribution generating function limn→∞{Fn(s) − Fn(0)} [1 – Fn(0)]–1. Particular attention is paid to the case F(s) = exp {m(s − 1)}.

Type
Short Communications
Information
Journal of Applied Probability , Volume 3 , Issue 1 , June 1966 , pp. 261 - 267
Copyright
Copyright © Sheffield: Applied Probability Trust 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Harris, T. E. (1963) The Theory of Branching Processes, Springer, Berlin.CrossRefGoogle Scholar
Heathcote, C. R., Seneta, E. and Vere-Jones, D. (1965), A refinement of two theorems in the theory of branching processes. Submitted for publication.Google Scholar
Kneser, H. (1950) Reele analytische Lösungen der Gleichung ?[?(x)] = ex und verwandter Funktionalgleichungen. J. Reine Angew. Math. 187, 56–67.CrossRefGoogle Scholar
Kolmogorov, A. N. (1938) Zur Lösung einer biologischen Aufgabe. Comm. Math. Mech. Chebychev Univ., Tomsk. 2, 1–6.Google Scholar
Montel, P. (1957) Leçons sur les Récurrences et leurs Applications. Gauthier-Villars, Paris.Google Scholar
Yaglom, A. M. (1947) Certain limit theorems of the theory of branching stochastic processes (in Russian). Dokl. Akad. Nauk SSSR, 56, 795–798.Google Scholar