1. Introduction
One of the largest sources of risk faced by corporations and their pension funds is longevity risk. Longevity risk is the risk that members of a population might live longer on average than expected. For example, suppose the population is a pool of annuitants. In that case, longevity risk is the risk that annuitants live longer on average than predicted in the mortality tables, which are used to price the annuities. Longevity risk is an important problem because of the uncertainty associated with longevity projections and the large number of liabilities exposed to longevity risk. The uncertainty of longevity projections is illustrated by the fact that life expectancy for men aged 60 was more than five years longer in 2005 than it was predicted to be in mortality projections made in the 1980s.Footnote 1 The amounts at risk are illustrated by the U.S. defined benefit plans that have been estimated to be valued at approximately 2.2 trillion dollars in 2007Footnote 2 and to cover 42 million participants. Swiss Re estimates that the total global exposure to longevity risk is approximately $21 trillion.Footnote 3
Exposure to longevity risk is a serious issue, yet traditionally, corporations and pension funds have had few means of managing it. Until recently, longevity risks were not securitized, and there were no longevity derivatives that these institutions could use to hedge their longevity risk exposures. This state of affairs has changed, and the new life market for longevity-linked financial instruments is continuing to develop.Footnote 4 However, the new life markets have been slow to grow due to agency costs associated with the securitization of longevity risk, e.g., see Zelenko (Reference Zelenko2014) and MacMinn and Brockett (Reference MacMinn and Brockett2017). In the interim, insurers and reinsurers have provided a means of transferring longevity risks through instruments such as buy-ins and buy-outs. These instruments transfer longevity and other risks from the corporate pension fund to the insurer. The buy-in or buy-out transfers the pension liability from the corporation to the insurer for a fee, and the insurer assumes the longevity and other risks associated with the pension fund. If the corporation must issue debt to finance the buy-in or buy-out, that corporation replaces the random liability with a known fixed payment on the bond. There are other instruments designed to hedge the longevity risk, including longevity swaps and q-forward contracts.Footnote 5 The swap pays the difference between the indemnity payoff and the index payoff at each date, while the q-forward exchanges the risky payoff then with a certain payoff, much like the buy-in or buy-out.
Interest in the notion of longevity risk and survivor or longevity bonds was initiated by Blake and Burrows (Reference Blake and Burrows2001). The literature on mortality and longevity risks and capital market instruments designed to hedge those risks has grown significantly since then. In 2003 Swiss Re successfully introduced a mortality-based security designed to hedge mortality changes in its life book of business. The concern was mortality risk, i.e., the risk of premature death. Since then, mortality bonds have become standard instruments for transferring mortality risk to the capital markets. The development of financial instruments to hedge longevity risk has generated academic and institutional interest. Longevity bonds have been designed but never issued due to a lack of demand. Forward contracts designed to hedge longevity risk have also lacked sufficient demand to make a market. On the other hand, buy-ins and buy-outs have been used by corporations to transfer longevity risk and so have generated an active market for longevity risk transfer.
Several reasons have been proposed for the failure of most longevity risk transfer instruments, including longevity bonds and q-forwards, etc. Blake et al. (Reference Blake, Cairns and Dowd2006) provides several possible reasons for the failure of longevity bonds. The reasons include (i) too short a time horizon on the bond to create an effective hedge, (ii) an excessive capital cost imposed on the hedgers, (iii) longevity risk that was transferred to a reinsurer rather than the capital markets, (iv) credit risk that would rest with the hedgers, and (v) too much basis risk relative to the price. These are primarily comments about the design of the longevity bond. While also noting basis risk, Lin and Cox (Reference Lin and Cox2008) provide a different rationale for these failures; they price the 2003 Mortality bond provided by Swiss Re through the special purpose vehicle Vita Capital I and then use the same model to price the European Investment Bank's longevity bond issue. They find that the risk premium in the mortality bond is less than that in the longevity bond. More importantly, the latter's high-risk premium was higher than the premium required by reinsurers, and so they claim that the high premium effectively caused the market failure. Chen and Cummins (Reference Chen and Cummins2010) also build a pricing model but claim that previous failures were due to design flaws; their model provides the same type of tail protection for hedgers as found in the mortality bond. Finally, Zelenko (Reference Zelenko2014), who was involved in the design and construction of the two attempted longevity bond issues in Chile, concluded in his piece that the reason for the market failures of those two attempted issues was the moral hazard problem. In referring to longevity risk, his explanation of the problem was “… it is remote and of low probability, and while it could jeopardize the firm's solvency, the government would have no choice but to intervene and bail out insurers, since all firms would be hit at the same time. Therefore, the optimal rational choice is to keep going or ‘keep dancing.’” The “keep dancing” is the moral hazard problem. The analysis here provides a simple model that captures longevity risk and the moral hazard problem; the moral hazard problem generates what is referred to as an agency problem in finance, e.g., see Jensen and Meckling (Reference Jensen and Meckling1976) for a discussion of agency costs. The agency problem exists even in the absence of any perceived government bailout. The optimal corporate choice, ceteris paribus, is to not hedge longevity risk. The analysis shows that the insolvency risk caused by the longevity risk yields an agency problem that is also called the under-investment problem. Finally, the analysis contributes to the literature by showing that the buy-in, buy-out or longevity bond can hedge the risk, solve the under-investment problem and create value for the pension fund and the current shareholders.
The paper is structured as follows: the financial market model for a corporation and its pension fund is constructed in the next section. The model is a complete financial market model like that first constructed by Arrow (Reference Arrow1963), Debreu (Reference Debreu1959), and subsequently by MacMinn (Reference MacMinn1987) and others. Some of the financial instruments for hedging longevity risk, i.e., buy-in and buy-out contracts, are considered in subsequent sections. The buy-ins and buy-outs are considered because the market for these instruments have been successful while those for other longevity risk transfer instruments such as longevity bonds have failed. The choice of a buy-in or buy-out contract is made because of the simplicity of the contracts and the active markets for them. The longevity bond is also modeled here even though there has not been a successful issue. The analysis of the longevity bond shows that it may also be used to successfully hedge the longevity risk and solve the under-investment problem even if there is basis risk. For each hedging instrument, we show that the stock value of the hedged firm is less than that of the unhedged firm, ceteris paribus, if the firm faces insolvency risk. It is the insolvency risk in conjunction with limited liability that introduces an agency cost for corporate management. Although hedging with the buy-ins, buy-outs, or longevity bond increases pension fund value, that same hedging, ceteris paribus, reduces the value of the current shareholders' stake in the firm. Since corporate management acts in the interests of current shareholders,Footnote 6 that management chooses not to hedge the longevity risk. Next, we show that if the firm has risky investment opportunities that yield sufficiently positive risk-adjusted net present values, hedging the longevity risk with these instruments may solve what would otherwise have been an under-investment problem. The solution to the under-investment yields an increase in value for the pension fund and for the current shareholders. The last section provides concluding remarks.
2. The financial market model
Consider a corporation in a competitive economy operating between the dates t = 0 and 1. The dates t = 0 and 1 are subsequently referred to as now and then, respectively. Decisions are made now, and payoffs on those decisions are received then. The economy is composed of corporations and risk-averse investors. Investors make portfolio decisions to maximize their expected utility subject to a budget constraint.Footnote 7 The corporation of interest here is a firm operating in a market for goods and services, making an investment decision and managing its corporate pension plan.
The firm is assumed to operate in competitive markets and makes investment decisions to advance its operations. The firm also has a pension plan for its employees that consists of an asset portfolio to cover the pension liabilities. Let Π (I, ω) denote the random payoff then on the investment of I dollars now; suppose that the investment frontier Π is increasing and concave in the dollar investment. Let A and L denote the asset and liability values then for the pension fund. Suppose the asset and liability values increase in state.Footnote 8
The firm also faces common capital market risks such as interest rate and insolvency risks in addition to the longevity risk on its pension plan. The returns on its pension asset portfolio payoff then, and the liability portfolio is composed of the pension payouts made then. The following partially summarizes the notation used in the development of the model:

The corporation is managed by an individual who makes decisions now. This individual will be referred to as the chief executive officer (CEO). The CEO makes the same decisions that other investors make and, in addition, makes decisions on behalf of the corporation. To distinguish between the two sets of decisions, the investor decisions on a portfolio are called decisions on personal account while the CEO decisions on investment, financing, and risk management are called decisions on corporate account. The CEO, like the investors, has an increasing concave utility function that expresses preferences for consumption now and then. The CEO makes decisions to maximize expected utility subject to a budget constraint and the corporate financing constraint. All decisions on personal and corporate account are made now, and the payoffs on those decisions occur then. We assume that the CEO is paid in salary and stock; it follows that the CEO makes decisions on corporate account to maximize the current shareholder value subject to any financing constraint.Footnote 10
3. The unhedged firm
Suppose the financial markets are competitive. In the absence of any hedge and letting b denote the promised payment on debt issued to finance the firm's capital investment, the stock market value of the firm may be expressed as:Footnote 11
where max{0, Π + A − L − b} is the firm's payoff in the absence of any hedging instrument.
3.1 No insolvency risk
First, consider the firm with no insolvency risk due to its investment decisions or pension plan. Suppose the firm finances any investment decision with debt. No insolvency provides a base case in which the firm makes decisions in the interests of all the stakeholders, i.e., stockholders, bondholders, and pension holders. The stock value in this case is
The stock value may also be expressed as

where V is the corporate value, Δ is the over or underfunding of the pension plan, and B(b) is the value of the safe debt issue used to finance the firm's investment decision. The firm's constrained maximization problem is
The Lagrange function is
and we have the following first order conditions:
It follows by (6) and (5), that the optimal investment is the socially efficient level I e implicitly defined by
The investment I e maximizes the stock value of the corporation without changing the value of the debt or pension plan. Given the financing constraint in (4), it follows that the stock market value of the firm may also be expressed as
where npv is the risk-adjusted net present value of the investment I e. This investment maximizes the npv while Δ is the over or underfunding of the pension plan; if the plan is underfunded, then the firm invests if and only if the net present value covers the underfunding and provides a return for shareholders.
3.2 Insolvency risk
From the firm's perspective, the pension plan exposes the corporation to the risk that the pensioners live longer than expected, and so we refer to it as longevity risk. The longevity risk may yield insolvency risk if the pension asset portfolio and corporate payoff are insufficient to cover the pension liability.
To note the pension and insolvency risk it is instructive to rewrite the stock value. Observe that max{0, Π + A − L − b} = Π + A − L − b + max{0, L + b − (Π + A)} and so the stock value may also be expressed as follows:

where V is the corporate value, V A and V L are pension asset and liability portfolio values and (P b + P u) is the put option value.Footnote 12 Letting γ be implicitly defined by the condition Π(I, γ) + A(γ) − L(γ) = 0 and δ is implicitly defined by the condition Π(I, δ) + A(δ) − L(δ) − b = 0, these values are
and

The pension liability value and put option values are shown in Figures 1 and 2. Given insolvency risk, the firm puts P b to bondholders and P u to the pension holders.

Figure 1. The Unhedged Firm.
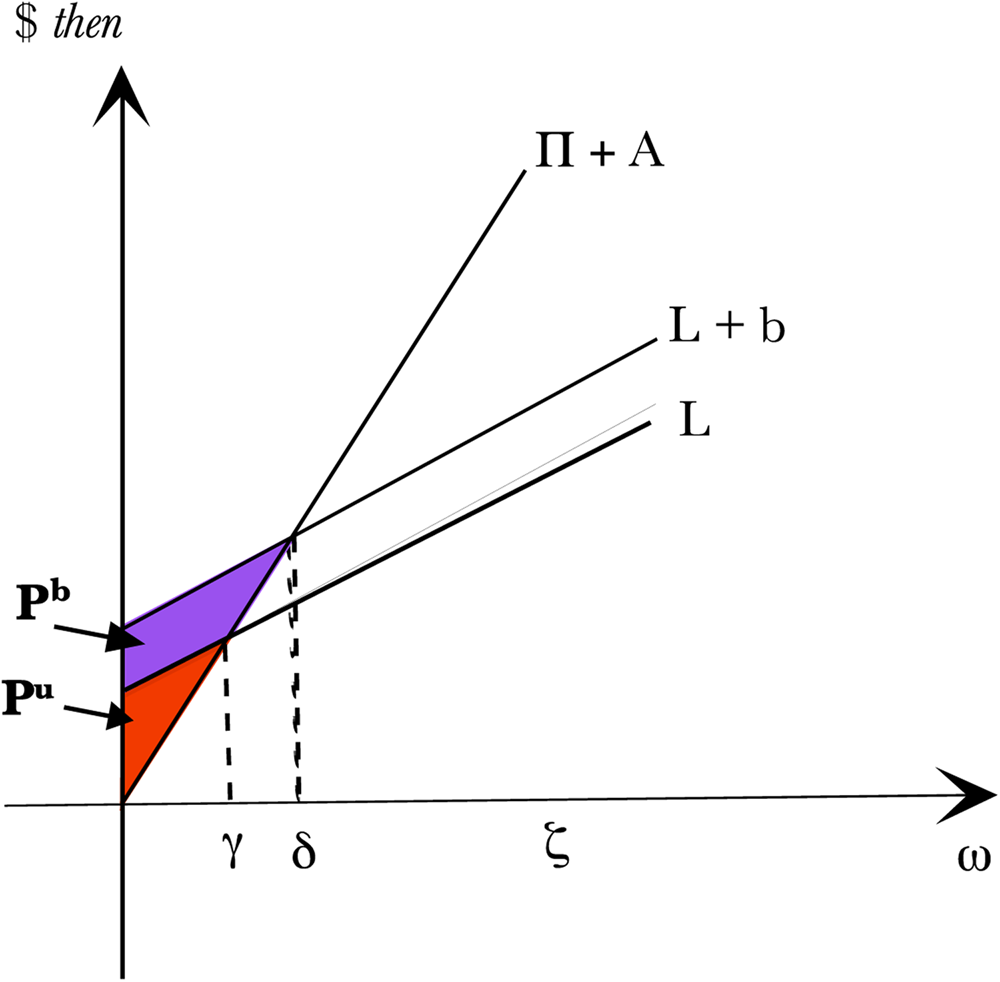
Figure 2. The Option Values.
If there is an implicit or explicit guarantee by the government, then the difference is put to the government rather than the annuity holders.
Now consider the investment decision made by the corporate manager. Given appropriately aligned incentives, the manager will select the investment to maximize the stock value subject to a financing constraint.Footnote 13 Without loss of generality, suppose the firm issues debt to cover the investment expenditure; let D(b) denote the value of the debt, and b represent the promised payment then. The manager's constrained maximization problem is
where
and

Let L(I, b, λ) = S u + λ(D(b) − I) be the Lagrange function for the constrained maximization problem. Then the first order conditions are as follows:

Let I u denote the optimal investment decision for the manager. Note that by (14), the Lagrange multiplier is one, and so the first-order condition for the optimal investment is
It is useful to compare the investment decision with the same decision made in the absence of insolvency risk. Let I e denote that decision and call it the socially efficient investment; the socially efficient decision is made in the interests of all stakeholders in the corporation rather than just the stockholders. The socially efficient investment will satisfy the following condition:
(8) implicitly defines the socially efficient investment. If (17) is evaluated at the optimal investment level for the unhedged firm, i.e., I u, then the first term on the right-hand side of (17) remains and makes (17) positive; it follows by the second-order condition that the manager selects I u < I e. Therefore, the unhedged firm underinvests relative to the socially efficient level of investment. The stock market value of the unhedged firm may also be written as follows:

Given insolvency risk due to the pension fund and debt liabilities, the CEO only makes the capital investment decision if the risk-adjusted net present value exceeds any underfunding plus the pension put value.
It is also possible to obtain the value of the pension fund for the unhedged firm. If the value of the pension asset portfolio is less than the liability value, then the pension plan is underfunded, and Δ is negative. The value of the pension fund is then F u = V L − P u.
4. The buy-in
The liability claims in the pension plan are one source of the insolvency risk for the firm. This risk becomes more of a problem for the firm as longevity increases. The firm can absorb the risk or chose to manage it in some way. Buy-in and buy-out instruments represent a growing segment of the markets for longevity risk transfer. The buy-in is considered first.
A buy-in is a transaction with an insurer in which the firm purchases an annuity that pays the pension claims as they are realized. Without loss of generality, we will suppose that the firm uses debt to finance the annuity purchase from an insurer. Then the constraint in the constrained maximization problem becomes
Hence, a debt instrument replaces the pension liability as well as providing funds for the capital investment. The stock value for the buy-in becomes
where S i denotes the hedged corporate equity value (Figure 3). Similarly, let I i denote the stock value maximizing investment decision. The Lagrange function is

and the first order conditions are as follows:

Figure 3. The Hedged Firm.
From (20) and (19) it follows that I i = I e > I u and so the buy-in resolves the under-investment problem. This under-investment problem is similar to that noted by Myers (Reference Myers1977); also, see Mayers and Smith (Reference Mayers and Smith1987), Garven and MacMinn (Reference Garven and MacMinn1993), MacMinn and Garven (Reference MacMinn, Garven and Dionne2011) for insurance schemes designed to alleviate the under-investment. Also, see Jensen and Meckling (Reference Jensen and Meckling1976), Green (Reference Green1984), MacMinn (Reference MacMinn1993) and MacMinn (Reference MacMinn2005b) for earlier examples of the agency costs of publicly traded firms and the impact of those costs on production, investment and financing decisions.
The shareholder value may also be expressed as

where B(b) is the safe debt value and P i is the put option value, npv is the risk-adjusted net present value of the investment and Δ = V A − V L is the under or over funding of the pension plan. The difference in stock value between the unhedged and hedged firms is

Using (23), it may be noted that if the CEO managed the firm's longevity risk with a buy-in but maintained the same investment level at I u then the difference in stock value would be −P u since the buy-in would eliminate that put option value. Hence, the CEO acting in the interests of current shareholders would not transfer the longevity risk with a buy-in.
The CEO, however, has the ability to make a capital investment on behalf of the firm. Equation (22) shows that the CEO making the investment decision to maximize current shareholder value will equivalently make the corporate investment decision to maximize the risk adjusted net present value of the project. It follows, using (23), that the CEO will make the socially efficient investment decision and complete the buy-in if the increase in risk-adjusted net present value exceeds the loss in pension option value.
5. The buy-out
Consider a buy-out. In this case, the pension plan is transferred to another firm, e.g., insurer or reinsurer. The pension asset and liability portfolios that compose the plan are transferred. Suppose further that the pension plan is under-funded in the sense that the value of the asset portfolio is less than that of the liability portfolio, i.e.,
In the under-funded case, Δ is negative, and the firm must raise −Δ dollars as part of the payment for the buy-out. The buy-out consists of this payment plus the transfer of the pension plan portfolios. The corporate payoff was Π + A − L before the buy-out while it is Π − b after the buy-out, where b is the promised payment on a bond issue needed to raise the −Δ dollars plus any investment expenditure. The constrained maximization problem for the firm in planning the buy-out and investment is
where

Letting the Lagrange function in this case be L(I, b, λ) = S o + λ(D + Δ − I). Then

From (26) it follows that λ = 1 and so by (25) it follows that
Therefore, the investment level that maximizes this constrained maximization problem is I o which is implicitly defined by (25). It also follows then that I o = I i = I e. The buy-out solves the under-investment problem just as the buy-in does.
Next, recall that the stock value of the firm after the buy-out is

It may be noted that the net present value of the firm investment in the buy-in and buy-out cases is the same, and so the stock value of the firm will also be the same in each case.
6. Longevity bonds without basis risk
Finally, consider a longevity bond. Let B(ω) be the longevity bond payoff then in state ω. Suppose there is no basis risk so that the bond payoff matches the pension claims then, i.e., B(ω) = L(ω) for each state ω ∈ Ω. If the corporation hedges the longevity risk with a longevity bond, the corporate payoff becomes Π + A − L + B − b = Π + A − b. Without loss of generality, suppose the corporation raises the money for investment and the longevity bond with a debt issue. Then the financing constraint is
where the debt value is
and the stock value given the longevity bond is
The Lagrange function for this constrained maximization problem is

and the first order conditions are:

It follows from these first order conditions that the optimal investment I l is implicitly defined by the condition:
and so, it also follows that the longevity bond hedge with no basis risk solves the under-investment problem and makes I l = I e > I u. It may be noted that the stock value of the corporation hedged with a longevity bond may be equivalently expressed as

Hence the longevity bond with no basis risk solves the under-investment problem that exists for the unhedged firm and yields I l = I e > I u.
7. Longevity bond with basis risk
If there is basis risk, then the payoff on a longevity bond is tied to a longevity index that is not perfectly correlated with the longevity of the pension fund. The corporation will not be able to perfectly hedge the longevity risk of the pension fund. It may, however, be able to reduce the longevity risk and alleviate the under-investment problem.
Let the basis risk be denoted by L(ω) − L I(ω). This represents the difference between the realized liability of the pension fund in a particular state versus the realized value of a longevity index where the index is based on a similar but not identical population. Suppose that longevity index over-estimates the pension fund liability then for lower states while the index under-estimates the pension fund liability then for higher states as shown in Figure 4. Let B l denote the risk adjusted market value of the longevity bond so that (Figure 5)
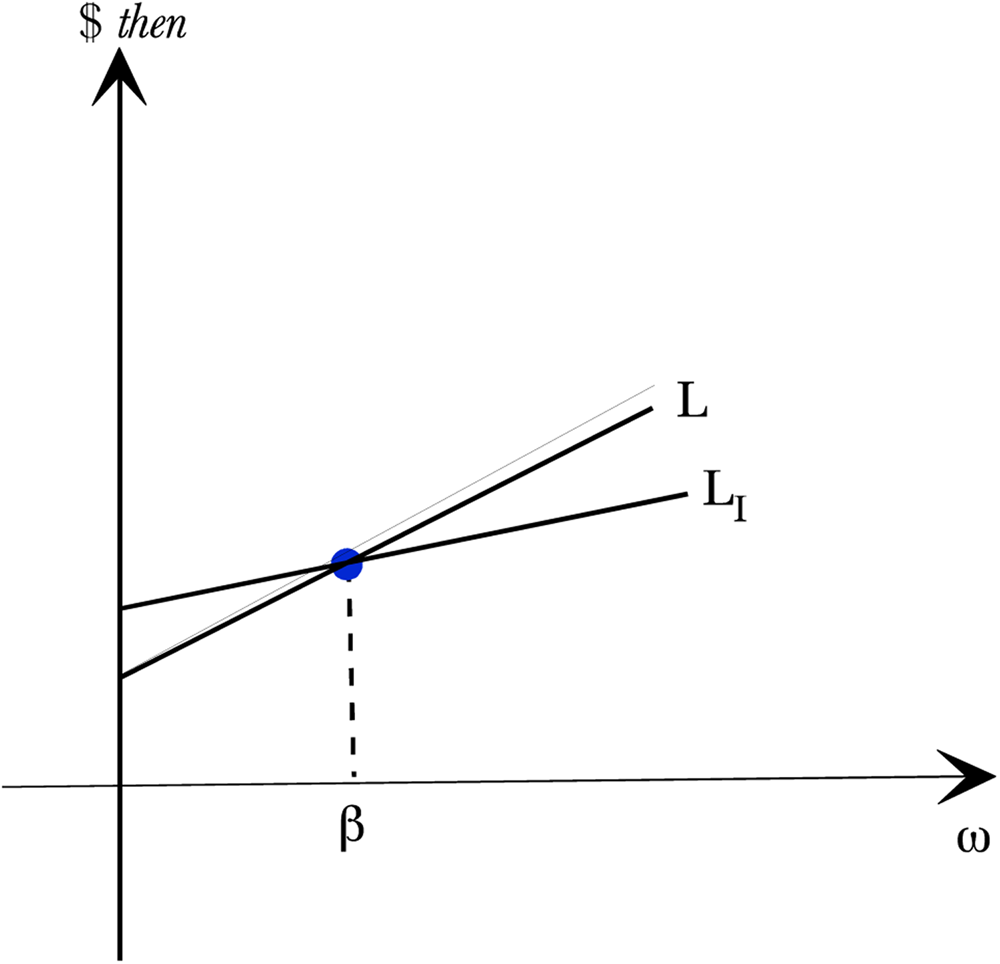
Figure 4. Basis Risk.
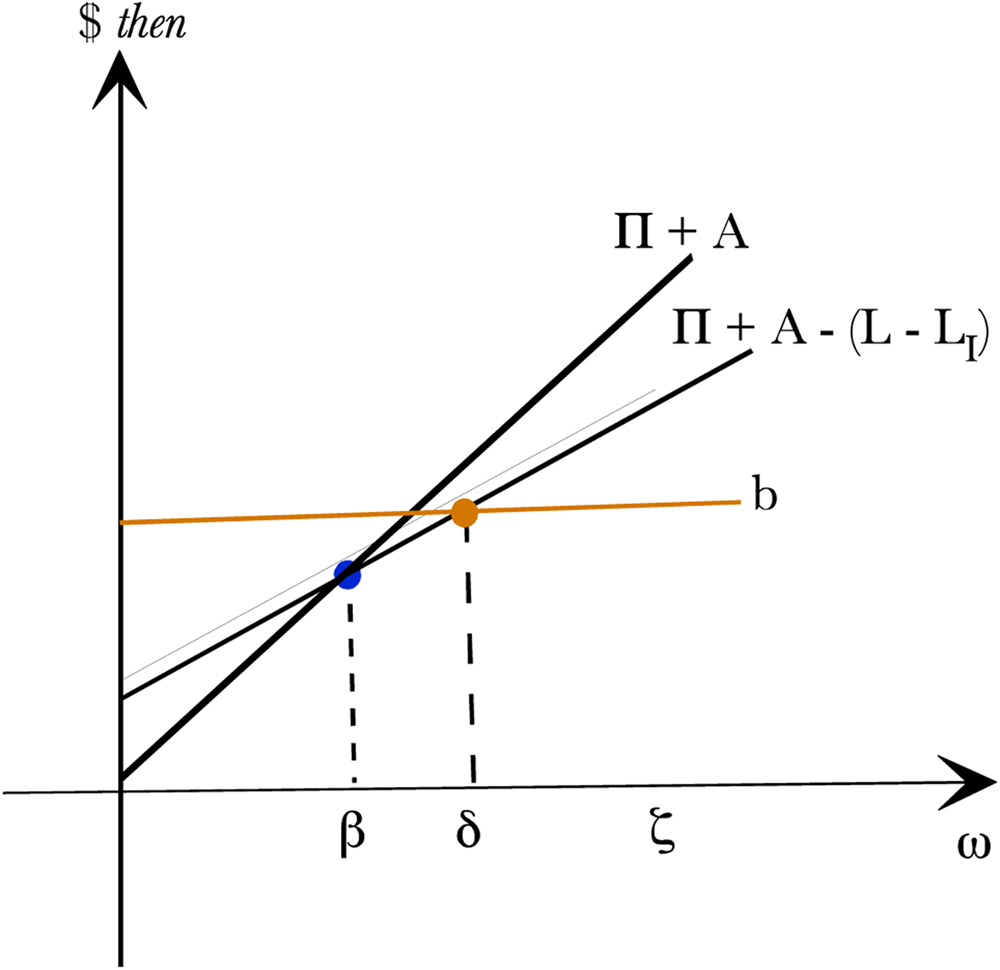
Figure 5. Corporate Payoff with Basis Risk.
If the corporation hedges with this longevity bond, then the shareholder payoff becomes

and the stock market value of the hedged corporation with a longevity bond is
and the bond value of a new issue is
The constrained maximization problem becomes

The Lagrange function is
and the first order conditions are as follows:

As before the stock market value of the corporation hedging with the longevity bond is

where npv(I l) is the risk adjusted net present value of the investment I l = I e. The last equality follows by the financing constraint that makes the debt value equal to the investment expenditure plus the value of the indexed longevity bond. Here again, the longevity bond solves the under-investment problem and allows the firm to select the efficient investment level if the risk adjusted net present value of the efficient investment is greater than the net present value plus the put option value of the unhedged corporation. The basis risk introduces a costFootnote 14 but if the longevity bond hedges the insolvency risk, then the longevity bond will reduce or eliminate the under-investment problem.
8. Concluding remarks
The analysis here has shown that the buy-in and buy-out instruments purchased from insurers can be used to manage the longevity risk of the corporate pension plan. These instruments transfer the risk and increase the value of the pension plan, but ceteris paribus, such hedging was shown to reduce shareholder value. Furthermore, the analysis has shown that if the corporation has an investment opportunity but a pension plan that exposes it to insolvency risk, it faces an under-investment problem. The CEO invests to the point at which the marginal risk-adjusted present value of the investment equals one plus the marginal agency cost of the under-investment problem; the marginal agency cost is represented by the marginal loss in the put option value P u. Effective risk management instruments eliminate or reduce the marginal agency cost. The model introduced here is the first theoretical model to show that buy-ins and buy-outs eliminate the agency cost and so the under-investment.
The model predictions are supported by some empirical evidence. For example, the deals by GM and Verizon with Prudential in 2012. These two deals are two of the largest buy-out transactions to date. Prior to the pension buy-out announcement, GM shares had gone down by 3.4% as it released weaker-than-expected auto sales figures. However, the stock recouped its losses and rose as much as 5.1% on the news of the pension buy-out on June 1, 2012, i.e., see Seetharaman and Klayman (Reference Seetharaman and Klayman2012). Similarly, on October 17, 2012, Verizon shares closed at $44.72, up 1.5%, after it announced its buy-out deal with Prudential.Footnote 15 Also, consistent with the model's prediction, after the pension buy-outs, both companies increased their investments significantly.
The analysis also shows that a longevity bond with or without basis risk can be used to manage the longevity risk. With no basis risk, the results are equivalent to those of the buy-in and buy-out. With basis risk, the risk management becomes more difficult because the index used for the liability payoff does not perfectly match the actual liability; this, in turn, increases the cost of the risk management. Despite this cost, the analysis shows in a simple setting that an instrument with basis risk can be used to manage the longevity risk and eliminate the under-investment problem.
Here the longevity risk was the source of the insolvency risk and so the longevity linked financial instruments could be used to manage that risk. An expanded model in which there are more sources of insolvency risk will make the instruments considered here possibly less effective. Such a model while possibly showing that the longevity linked instruments cannot eliminate the agency cost associated with the under-investment problem may also show that those instruments will reduce the agency cost and that other hedging instruments used in conjunction with them can eliminate the agency cost.







