Published online by Cambridge University Press: 06 May 2022
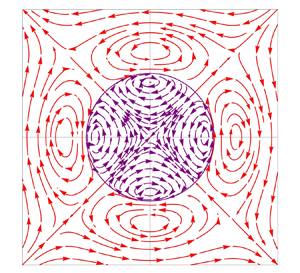
The fluid mechanics problem of an initially spherical drop subjected to a two-directional nonlinear cubic shear flow, under creeping flow conditions, is theoretically studied. This physical situation is described by four dimensionless numbers: the capillary number (Ca), the viscosity ratio (λ), the linear velocity ratio (G) and the nonlinear intensity of the flow (E). Analytical expressions for the external and internal flows of a spherical drop as well as for the shape of the deformed drop were obtained by applying Lamb's general solution to the Stokes equations. The internal and external streamlines of a spherical drop (Ca = 0) suggest interesting and unusual shapes which are sometimes also accompanied by many stagnation points. When λ = ∞, the drop becomes a solid sphere with an angular velocity  $\omega ={-} (1 - G)/2$ independent of E. For the familiar simple shear flow in the x-direction (G = 0, E = 0), Taylor's theory, valid at
$\omega ={-} (1 - G)/2$ independent of E. For the familiar simple shear flow in the x-direction (G = 0, E = 0), Taylor's theory, valid at  $Ca \ll 1$ and λ = O(1), predicts an ellipsoidal drop oriented at an azimuthal angle ϕ = 45°; as Ca increases, the deformation increases and the drop attains a peanut shape. For other linear or nonlinear cases, the drop is aligned at ϕ = ±45°, and a spherical drop with no deformation is possible at Ca ≠ 0. In a highly viscous drop
$Ca \ll 1$ and λ = O(1), predicts an ellipsoidal drop oriented at an azimuthal angle ϕ = 45°; as Ca increases, the deformation increases and the drop attains a peanut shape. For other linear or nonlinear cases, the drop is aligned at ϕ = ±45°, and a spherical drop with no deformation is possible at Ca ≠ 0. In a highly viscous drop  $(\lambda \gg 1)$, the drop remains almost spherical even at Ca = O(1), oriented at ϕ = 0° or 90° (G ≠ 1), and its deformation is independent of Ca.
$(\lambda \gg 1)$, the drop remains almost spherical even at Ca = O(1), oriented at ϕ = 0° or 90° (G ≠ 1), and its deformation is independent of Ca.