Article contents
Experimental investigation of the wave-induced motion of and force distribution along a flexible stem
Published online by Cambridge University Press: 18 October 2019
Abstract
The work presents an experimental investigation into the motion of and hydrodynamic forces along a single flexible stem in regular waves. The experiment covers a large range in relevant non-dimensional parameters: the drag-to-stiffness ratio 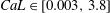 $CaL\in [0.003,3.8]$, the inertia-to-stiffness ratio
$CaL\in [0.003,3.8]$, the inertia-to-stiffness ratio 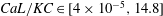 $CaL/KC\in [4\times 10^{-5},14.8]$, the Keulegan–Carpenter number
$CaL/KC\in [4\times 10^{-5},14.8]$, the Keulegan–Carpenter number 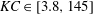 $KC\in [3.8,145]$ and the Reynolds number
$KC\in [3.8,145]$ and the Reynolds number 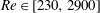 $Re\in [230,2900]$. The two first parameters relate to the response of the stem in waves and thus account for material properties, while the two last parameters are relevant for hydrodynamic forces on the stem. The displacement of the stem was captured with a digital video camera and the displacement along the stem was captured for every 2.5 mm at 25 Hz. This unique laboratory data set allowed for the following analyses: (i) Determination of the relevant non-dimensional parameter to predict the stem motion and shape. (ii) A direct comparison between the measured force for mimics of two lengths (0.15 m and 0.30 m) illustrating the force reduction potential for flexible mimics. (iii) Direct evaluation of the average force coefficients
$Re\in [230,2900]$. The two first parameters relate to the response of the stem in waves and thus account for material properties, while the two last parameters are relevant for hydrodynamic forces on the stem. The displacement of the stem was captured with a digital video camera and the displacement along the stem was captured for every 2.5 mm at 25 Hz. This unique laboratory data set allowed for the following analyses: (i) Determination of the relevant non-dimensional parameter to predict the stem motion and shape. (ii) A direct comparison between the measured force for mimics of two lengths (0.15 m and 0.30 m) illustrating the force reduction potential for flexible mimics. (iii) Direct evaluation of the average force coefficients 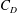 $C_{D}$ (drag) and
$C_{D}$ (drag) and 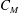 $C_{M}$ (inertia) for the flexible stems. (iv) The distributed external hydrodynamic loading and the internal shear forces were estimated from the laboratory experiments. The distribution of the shear force helped to understand the breakage mechanisms of flexible stems. (v) A linkage between phase lags and internal shear forces was suggested. The data set is considered valuable as validation material for numerical models of stem motion in waves.
$C_{M}$ (inertia) for the flexible stems. (iv) The distributed external hydrodynamic loading and the internal shear forces were estimated from the laboratory experiments. The distribution of the shear force helped to understand the breakage mechanisms of flexible stems. (v) A linkage between phase lags and internal shear forces was suggested. The data set is considered valuable as validation material for numerical models of stem motion in waves.
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © 2019 Cambridge University Press
References
Jacobsen et al. supplementary movie 1
Movie of the stem motion for mimic 2 with $H = 0.04$ m, $T = 2.0$ s and $l = 0.30$ m. The waves propagate from left to right. Top-left panel: Stem and relative velocities; 1.0 m corresponds to 1.0 m/s. Top-right panel: The distributed hydrodynamic force. Bottom-left panel: Stem and relative accelerations; 0.2 m corresponds to 1.0 m/s${}^2$. Bottom-right panel: The internal shear force.
Jacobsen et al. supplementary movie 2
Movie of the stem motion for mimic 2 with $H = 0.11$ m, $T = 3.0$ s and $l = 0.30$ m. The waves propagate from left to right. Top-left panel: Stem and relative velocities; 0.4 m corresponds to 1.0 m/s. Top-right panel: The distributed hydrodynamic force. Bottom-left panel: Stem and relative accelerations; 0.1 m corresponds to 1.0 m/s${}^2$. Bottom-right panel: The internal shear force.
Jacobsen et al. supplementary movie 3
Movie of the stem motion for mimic 3 with $H = 0.04$ m, $T = 2.0$ s and $l = 0.30$ m. The waves propagate from left to right. Top-left panel: Stem and relative velocities; 1.0 m corresponds to 1.0 m/s. Top-right panel: The distributed hydrodynamic force. Bottom-left panel: Stem and relative accelerations; 0.2 m corresponds to 1.0 m/s${}^2$. Bottom-right panel: The internal shear force.
Jacobsen et al. supplementary movie 4
Movie of the stem motion for mimic 3 with $H = 0.11$ m, $T = 3.0$ s and $l = 0.30$ m. The waves propagate from left to right. Top-left panel: Stem and relative velocities; 0.5 m corresponds to 1.0 m/s. Top-right panel: The distributed hydrodynamic force. Bottom-left panel: Stem and relative accelerations; 0.1 m corresponds to 1.0 m/s${}^2$. Bottom-right panel: The internal shear force.
A correction has been issued for this article:
- 35
- Cited by
Linked content
Please note a has been issued for this article.

