Published online by Cambridge University Press: 12 August 2020
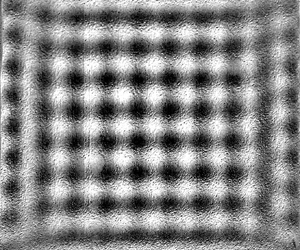
Pattern-forming instability in various fields is an interesting research topic because of its complex physical nature and numerous applications. In this paper, we experimentally study capillary surface waves and patterns formed on a liquid film, cast on a plane substrate without physical walls, but pinned to the substrate edges, and subjected to multi-axis horizontal (lateral) oscillations (55–333 Hz). The effect of single-axis ultrasonic horizontal vibrations (20–170 kHz) was also investigated. We show that using substrates with different geometrical shapes and various travelling paths created by multi-axis vibrations with a phase angle difference between the axes produce a plethora of standing and travelling wave patterns on the liquid film surface. We report perfect standing square and spiral-like patterns for low-frequency multi-axis horizontal vibrations, which are commonly observed for vertical vibrations, while the mechanisms of momentum transfer to the liquid film from the vibrating substrate are different in vertical and horizontal vibrations. Other patterns forming on the liquid film surface in our experiments include lines/stripes, circles, swirls, pentagons, triangles, etc. It is also reported that low-frequency excitations create harmonic travelling waves and standing patterns, while the frequency of response waves generated by the application of ultrasonic horizontal vibrations is several orders of magnitude less than the excitation frequency. No subharmonic cross-waves are observed in this study, which strengthens the idea that plane substrates (without walls) are a good approximation for the theoretical case of a horizontally vibrated liquid film with infinite lateral length.
Movie 3 shows the standing square pattern in a square substrate (s = 25 mm, f = 55 Hz, φ ≈ 0 deg).
Movie 4 shows the line switching pattern in a square substrate (s = 25 mm, f = 55 Hz, φ ≈ 90 deg).