Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Qadri, Ubaid Ali
Chandler, Gary J.
and
Juniper, Matthew P.
2018.
Passive control of global instability in low-density jets.
European Journal of Mechanics - B/Fluids,
Vol. 72,
Issue. ,
p.
311.
Moreno-Boza, Daniel
Coenen, Wilfried
Carpio, Jaime
Sánchez, Antonio L.
and
Williams, Forman A.
2018.
On the critical conditions for pool-fire puffing.
Combustion and Flame,
Vol. 192,
Issue. ,
p.
426.
Lesshafft, Lutz
2018.
Artificial eigenmodes in truncated flow domains.
Theoretical and Computational Fluid Dynamics,
Vol. 32,
Issue. 3,
p.
245.
Meunier, P.
and
Nadal, F.
2018.
From a steady plume to periodic puffs during confined carbon dioxide dissolution.
Journal of Fluid Mechanics,
Vol. 855,
Issue. ,
p.
1.
Coenen, Wilfried
Kolb, Erik J.
Sánchez, Antonio L.
and
Williams, Forman A.
2019.
Observed dependence of characteristics of liquid-pool fires on swirl magnitude.
Combustion and Flame,
Vol. 205,
Issue. ,
p.
1.
Bharadwaj, Kuchimanchi K.
and
Das, Debopam
2019.
Puffing in planar buoyant plumes: BiGlobal instability analysis and experiments.
Journal of Fluid Mechanics,
Vol. 863,
Issue. ,
p.
817.
Cavalieri, André V. G.
Jordan, Peter
and
Lesshafft, Lutz
2019.
Wave-Packet Models for Jet Dynamics and Sound Radiation.
Applied Mechanics Reviews,
Vol. 71,
Issue. 2,
Zhao, Yongling
Zhao, Pengpeng
Liu, Yang
Xu, Yin
and
Torres, Juan F.
2019.
On the selection of perturbations for thermal boundary layer control.
Physics of Fluids,
Vol. 31,
Issue. 10,
Zhu, Yuanhang
Gupta, Vikrant
and
Li, Larry K. B.
2019.
Coherence resonance in low-density jets.
Journal of Fluid Mechanics,
Vol. 881,
Issue. ,
Parente, E.
Robinet, J. C.
De Palma, P.
and
Cherubini, S.
2020.
Modal and nonmodal stability of a stably stratified boundary layer flow.
Physical Review Fluids,
Vol. 5,
Issue. 11,
Demange, S.
and
Pinna, F.
2020.
On the role of thermo-transport properties in the convective/absolute transition of heated round jets.
Physics of Fluids,
Vol. 32,
Issue. 12,
Wimer, N. T.
Lapointe, C.
Christopher, J. D.
Nigam, S. P.
Hayden, T. R. S.
Upadhye, A.
Strobel, M.
Rieker, G. B.
and
Hamlington, P. E.
2020.
Scaling of the puffing Strouhal number for buoyant jets and plumes.
Journal of Fluid Mechanics,
Vol. 895,
Issue. ,
Wimer, Nicholas T.
Day, Marcus S.
Lapointe, Caelan
Meehan, Michael A.
Makowiecki, Amanda S.
Glusman, Jeffrey F.
Daily, John W.
Rieker, Gregory B.
and
Hamlington, Peter E.
2021.
Numerical simulations of buoyancy-driven flows using adaptive mesh refinement: structure and dynamics of a large-scale helium plume.
Theoretical and Computational Fluid Dynamics,
Vol. 35,
Issue. 1,
p.
61.
Rendall, Joseph
Abu-Heiba, Ahmad
Gluesenkamp, Kyle
Nawaz, Kashif
Worek, William
and
Elatar, Ahmed
2021.
Nondimensional convection numbers modeling thermally stratified storage tanks: Richardson's number and hot-water tanks.
Renewable and Sustainable Energy Reviews,
Vol. 150,
Issue. ,
p.
111471.
Chen, Chang Hsin
and
Bhaganagar, Kiran
2021.
New findings in vorticity dynamics of turbulent buoyant plumes.
Physics of Fluids,
Vol. 33,
Issue. 11,
Bharadwaj, Kuchimanchi K.
and
Das, Debopam
2021.
Influence of Coflow on Buoyant Plume Puffing.
Journal of Fluids Engineering,
Vol. 143,
Issue. 9,
Srinivasan, Kumanan
Shrimali, Uttam Sunilbhai
and
Vinoth, B. R.
2021.
BiGlobal stability analysis of planar fountains.
Physics of Fluids,
Vol. 33,
Issue. 12,
Wang, Chuhan
Lesshafft, Lutz
and
Oberleithner, Kilian
2022.
Global linear stability analysis of a flame anchored to a cylinder.
Journal of Fluid Mechanics,
Vol. 951,
Issue. ,
Meehan, Michael A.
Wimer, Nicholas T.
and
Hamlington, Peter E.
2022.
Richardson and Reynolds number effects on the near field of buoyant plumes: temporal variability and puffing.
Journal of Fluid Mechanics,
Vol. 950,
Issue. ,
Demange, S.
Qadri, U.A.
Juniper, M.P.
and
Pinna, F.
2022.
Global modes of viscous heated jets with real gas effects.
Journal of Fluid Mechanics,
Vol. 936,
Issue. ,
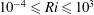 $10^{-4}\leqslant Ri\leqslant 10^{3}$ and density ratios
$10^{-4}\leqslant Ri\leqslant 10^{3}$ and density ratios  $S=\unicode[STIX]{x1D70C}_{\infty }/\unicode[STIX]{x1D70C}_{jet}$ between 1.05 and 7, only axisymmetric perturbations are found to exhibit global instability, consistent with experimental observations in helium jets. By varying the Richardson number over seven decades, the effects of buoyancy on the base flow and on the instability dynamics are characterised, and distinct behaviour is observed in the low-
$S=\unicode[STIX]{x1D70C}_{\infty }/\unicode[STIX]{x1D70C}_{jet}$ between 1.05 and 7, only axisymmetric perturbations are found to exhibit global instability, consistent with experimental observations in helium jets. By varying the Richardson number over seven decades, the effects of buoyancy on the base flow and on the instability dynamics are characterised, and distinct behaviour is observed in the low-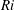 $Ri$ (jet) and in the high-
$Ri$ (jet) and in the high-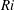 $Ri$ (plume) regimes. A sensitivity analysis indicates that different physical mechanisms are responsible for the global instability dynamics in both regimes. In buoyant jets at low Richardson number, the baroclinic torque enhances the basic shear instability, whereas buoyancy provides the dominant instability mechanism in plumes at high Richardson number. The onset of axisymmetric global instability in both regimes is consistent with the presence of absolute instability. While absolute instability also occurs for helical perturbations, it appears to be too weak or too localised to give rise to a global instability.
$Ri$ (plume) regimes. A sensitivity analysis indicates that different physical mechanisms are responsible for the global instability dynamics in both regimes. In buoyant jets at low Richardson number, the baroclinic torque enhances the basic shear instability, whereas buoyancy provides the dominant instability mechanism in plumes at high Richardson number. The onset of axisymmetric global instability in both regimes is consistent with the presence of absolute instability. While absolute instability also occurs for helical perturbations, it appears to be too weak or too localised to give rise to a global instability.