Article contents
Heat transport by turbulent rotating Rayleigh–Bénard convection and its dependence on the aspect ratio
Published online by Cambridge University Press: 02 September 2011
Abstract
We report on the influence of rotation about a vertical axis on heat transport by turbulent Rayleigh–Bénard convection in a cylindrical vessel with an aspect ratio 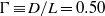 (
(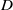 is the diameter and
is the diameter and 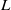 the height of the sample) and compare the results with those for larger
the height of the sample) and compare the results with those for larger  . The working fluid was water at
. The working fluid was water at 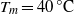 where the Prandtl number
where the Prandtl number 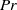 is 4.38. For rotation rates
is 4.38. For rotation rates 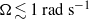 , corresponding to inverse Rossby numbers
, corresponding to inverse Rossby numbers 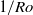 between zero and twenty, we measured the Nusselt number
between zero and twenty, we measured the Nusselt number 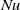 for six Rayleigh numbers
for six Rayleigh numbers 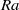 in the range
in the range 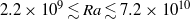 . For small rotation rates and at constant
. For small rotation rates and at constant 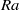 , the reduced Nusselt number
, the reduced Nusselt number 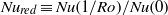 initially increased slightly with increasing
initially increased slightly with increasing 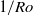 , but at
, but at 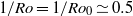 it suddenly became constant or decreased slightly depending on
it suddenly became constant or decreased slightly depending on 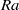 . At
. At  a second sharp transition occurred in
a second sharp transition occurred in 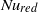 to a state where
to a state where  increased with increasing
increased with increasing  . We know from direct numerical simulation that the transition at
. We know from direct numerical simulation that the transition at  corresponds to the onset of Ekman vortex formation reported before for
corresponds to the onset of Ekman vortex formation reported before for 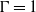 at
at 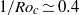 and for
and for  at
at 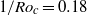 (Weiss et al., Phys. Rev. Lett., vol. 105, 2010, 224501). The
(Weiss et al., Phys. Rev. Lett., vol. 105, 2010, 224501). The  -dependence of
-dependence of 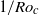 can be explained as a finite-size effect that can be described phenomenologically by a Ginzburg–Landau model; this model is discussed in detail in the present paper. We do not know the origin of the transition at
can be explained as a finite-size effect that can be described phenomenologically by a Ginzburg–Landau model; this model is discussed in detail in the present paper. We do not know the origin of the transition at  . Above
. Above 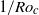 ,
, 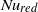 increased with increasing
increased with increasing 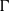 up to
up to 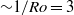 . We discuss the
. We discuss the 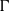 -dependence of
-dependence of 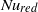 in this range in terms of the average Ekman vortex density as predicted by the model. At even larger
in this range in terms of the average Ekman vortex density as predicted by the model. At even larger 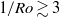 there is a decrease of
there is a decrease of 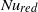 that can be attributed to two possible effects. First, the Ekman pumping might become less efficient when the Ekman layer is significantly smaller than the thermal boundary layer, and second, for rather large
that can be attributed to two possible effects. First, the Ekman pumping might become less efficient when the Ekman layer is significantly smaller than the thermal boundary layer, and second, for rather large 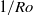 , the Taylor–Proudman effect in combination with boundary conditions suppresses fluid flow in the vertical direction.
, the Taylor–Proudman effect in combination with boundary conditions suppresses fluid flow in the vertical direction.
JFM classification
Information
- Type
- Papers
- Information
- Copyright
- Copyright © Cambridge University Press 2011
References
- 46
- Cited by

