Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Deparday, Julien
and
Mulleners, Karen
2019.
Modeling the interplay between the shear layer and leading edge suction during dynamic stall.
Physics of Fluids,
Vol. 31,
Issue. 10,
Benton, Stuart I.
and
Visbal, Miguel R.
2019.
Extending the Reynolds Number Range of High-Frequency Control of Dynamic Stall.
AIAA Journal,
Vol. 57,
Issue. 7,
p.
2675.
Benton, Stuart I.
and
Visbal, Miguel R.
2019.
Effects of Compressibility on Dynamic-Stall Onset Using Large-Eddy Simulation.
Sato, Makoto
Asada, Kengo
Nonomura, Taku
Aono, Hikaru
Yakeno, Aiko
and
Fujii, Kozo
2019.
Mechanisms for turbulent separation control using plasma actuator at Reynolds number of 1.6 × 106.
Physics of Fluids,
Vol. 31,
Issue. 9,
Sun, Baoyun
Ma, Binghe
Deng, Jinjun
Luo, Jian
Wang, Pengbin
Yan, Yuchao
Yuan, Weizheng
and
Jiang, Chengyu
2019.
High Sensitive Micro Flexible Thermal Sensor for Flow Separation Measurements on Airfoil.
p.
1993.
Visbal, Miguel R.
and
Garmann, Daniel J.
2019.
Effect of Sweep on Dynamic Stall of a Pitching Finite-Aspect-Ratio Wing.
AIAA Journal,
Vol. 57,
Issue. 8,
p.
3274.
Xing, Shi-Long
Xu, He-Yong
Ma, Ming-Sheng
and
Ye, Zheng-Yin
2020.
Inflatable Leading Edge-Based Dynamic Stall Control considering Fluid-Structure Interaction.
International Journal of Aerospace Engineering,
Vol. 2020,
Issue. ,
p.
1.
Taira, Kunihiko
Hemati, Maziar S.
Brunton, Steven L.
Sun, Yiyang
Duraisamy, Karthik
Bagheri, Shervin
Dawson, Scott T. M.
and
Yeh, Chi-An
2020.
Modal Analysis of Fluid Flows: Applications and Outlook.
AIAA Journal,
Vol. 58,
Issue. 3,
p.
998.
Yeh, Chi-An
Benton, Stuart I.
Taira, Kunihiko
and
Garmann, Daniel J.
2020.
Resolvent analysis of an airfoil laminar separation bubble at
Re=500000.
Physical Review Fluids,
Vol. 5,
Issue. 8,
Kim, Taesoon
Kim, Seungtae
Lim, Jiseop
and
Jee, Solkeun
2020.
Numerical investigation of compressibility effect on dynamic stall.
Aerospace Science and Technology,
Vol. 105,
Issue. ,
p.
105918.
Sun, Baoyun
Ma, Binghe
Wang, Pengbin
Luo, Jian
Deng, Jinjun
and
Gao, Chao
2020.
High sensitive flexible hot-film sensor for measurement of unsteady boundary layer flow.
Smart Materials and Structures,
Vol. 29,
Issue. 3,
p.
035023.
Chiereghin, N.
Bull, S.
Cleaver, D. J.
and
Gursul, I.
2020.
Three-dimensionality of leading-edge vortices on high aspect ratio plunging wings.
Physical Review Fluids,
Vol. 5,
Issue. 6,
Wen, Guangwei
and
Gross, Andreas
2020.
Local Linear Stability Analysis of Laminar Separation Bubble for Helicopter Blade Section Undergoing Dynamic Stall.
Visbal, Miguel R.
and
Garmann, Daniel J.
2020.
Mitigation of Dynamic Stall over a Pitching Finite Wing Using High-Frequency Actuation.
AIAA Journal,
Vol. 58,
Issue. 1,
p.
6.
Bull, S.
Chiereghin, N.
Cleaver, D. J.
and
Gursul, I.
2020.
Novel Approach to Leading-Edge Vortex Suppression.
AIAA Journal,
Vol. 58,
Issue. 10,
p.
4212.
Hoerner, Stefan
Bonamy, Cyrille
Cleynen, Olivier
Maître, Thierry
and
Thévenin, Dominique
2020.
Darrieus vertical-axis water turbines: deformation and force measurements on bioinspired highly flexible blade profiles.
Experiments in Fluids,
Vol. 61,
Issue. 6,
Benton, Stuart I.
and
Visbal, Miguel R.
2020.
Effects of Compressibility on Dynamic-Stall Onset Using Large-Eddy Simulation.
AIAA Journal,
Vol. 58,
Issue. 3,
p.
1194.
Gupta, Rohit
and
Ansell, Phillip J.
2020.
Flow Evolution and Unsteady Spectra of Dynamic Stall at Transitional Reynolds Numbers.
AIAA Journal,
Vol. 58,
Issue. 8,
p.
3272.
Shi, Lei
and
Wang, Yefang
2021.
Numerical investigations on transitional flows around forward and reversed hydrofoils.
European Journal of Mechanics - B/Fluids,
Vol. 85,
Issue. ,
p.
24.
Miller, Mark A.
Duvvuri, Subrahmanyam
and
Hultmark, Marcus
2021.
Solidity effects on the performance of vertical-axis wind turbines.
Flow,
Vol. 1,
Issue. ,
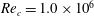 $Re_{c}=1.0\times 10^{6}$ through the use of wall-resolved large-eddy simulation. Emphasis is placed on the unsteady boundary-layer interactions that develop as the airfoil approaches stall. At this Reynolds number it is shown that turbulent separation moves upstream across much of the airfoil suction surface. When turbulent separation reaches the leading-edge separation bubble, a bursting event is initiated leading to a strong coherent leading-edge vortex structure. This vortex wraps up the turbulent shear layer to form a large dynamic stall vortex. The use of large-eddy simulation elucidates the roll of the laminar separation bubble in defining the onset of the dynamic stall process. Comparisons are made to identical simulations at lower Reynolds numbers of
$Re_{c}=1.0\times 10^{6}$ through the use of wall-resolved large-eddy simulation. Emphasis is placed on the unsteady boundary-layer interactions that develop as the airfoil approaches stall. At this Reynolds number it is shown that turbulent separation moves upstream across much of the airfoil suction surface. When turbulent separation reaches the leading-edge separation bubble, a bursting event is initiated leading to a strong coherent leading-edge vortex structure. This vortex wraps up the turbulent shear layer to form a large dynamic stall vortex. The use of large-eddy simulation elucidates the roll of the laminar separation bubble in defining the onset of the dynamic stall process. Comparisons are made to identical simulations at lower Reynolds numbers of 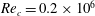 $Re_{c}=0.2\times 10^{6}$ and
$Re_{c}=0.2\times 10^{6}$ and 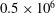 $0.5\times 10^{6}$. This comparison demonstrates trends in the boundary-layer mechanics that explain the sensitivity of the dynamic stall process to Reynolds number.
$0.5\times 10^{6}$. This comparison demonstrates trends in the boundary-layer mechanics that explain the sensitivity of the dynamic stall process to Reynolds number.