Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Garicano-Mena, J.
Li, B.
Ferrer, E.
and
Valero, E.
2019.
A composite dynamic mode decomposition analysis of turbulent channel flows.
Physics of Fluids,
Vol. 31,
Issue. 11,
Wang, Qingyang
Gan, Lian
Xu, Shengjin
and
Zhou, Yu
2020.
Vortex evolution in the near wake behind polygonal cylinders.
Experimental Thermal and Fluid Science,
Vol. 110,
Issue. ,
p.
109940.
Hasegawa, Kazuto
Fukami, Kai
Murata, Takaaki
and
Fukagata, Koji
2020.
CNN-LSTM based reduced order modeling of two-dimensional unsteady flows around a circular cylinder at different Reynolds numbers.
Fluid Dynamics Research,
Vol. 52,
Issue. 6,
p.
065501.
Fukami, Kai
Nakamura, Taichi
and
Fukagata, Koji
2020.
Convolutional neural network based hierarchical autoencoder for nonlinear mode decomposition of fluid field data.
Physics of Fluids,
Vol. 32,
Issue. 9,
Symon, S.
Illingworth, S. J.
and
Marusic, I.
2020.
Large-scale structures predicted by linear models of wall-bounded turbulence.
Journal of Physics: Conference Series,
Vol. 1522,
Issue. 1,
p.
012006.
Li, Binghua
Garicano-Mena, Jesús
Zheng, Yao
and
Valero, Eusebio
2020.
Dynamic Mode Decomposition Analysis of Spatially Agglomerated Flow Databases.
Energies,
Vol. 13,
Issue. 9,
p.
2134.
Kaplan, Oğuzhan
Jordan, Peter
Cavalieri, André V.G.
and
Brès, Guillaume A.
2021.
Nozzle dynamics and wavepackets in turbulent jets.
Journal of Fluid Mechanics,
Vol. 923,
Issue. ,
Fang, Xingjun
Tachie, Mark F.
Bergstrom, Donald J.
Yang, Zixuan
and
Wang, Bing-Chen
2021.
Three-dimensional structural characteristics of flow separation induced by a forward-facing step in a turbulent channel flow.
Journal of Fluid Mechanics,
Vol. 919,
Issue. ,
Hatzissawidis, G
Ludwig, G J
and
Pelz, P F
2021.
Modal Decomposition of Large- and Small-Scale Cloud Cavitation.
IOP Conference Series: Earth and Environmental Science,
Vol. 774,
Issue. 1,
p.
012097.
Ye, Weixiang
Geng, Chen
Ikuta, Akihiro
Hachinota, Shinya
Miyagawa, Kazuyoshi
and
Luo, Xianwu
2021.
Investigation on the impeller-diffuser interaction on the unstable flow in a mixed-flow pump using a modified partially averaged Navier-Stokes model.
Ocean Engineering,
Vol. 238,
Issue. ,
p.
109756.
Symon, Sean
Illingworth, Simon J.
and
Marusic, Ivan
2021.
Energy transfer in turbulent channel flows and implications for resolvent modelling.
Journal of Fluid Mechanics,
Vol. 911,
Issue. ,
Ng, Henry C.-H.
Collignon, Emile
Poole, Robert J.
and
Dennis, David J. C.
2021.
Energetic motions in turbulent partially filled pipe flow.
Physics of Fluids,
Vol. 33,
Issue. 2,
Asim, Taimoor
and
Islam, Sheikh
2021.
Effects of Damaged Rotor on Wake Dynamics of Vertical Axis Wind Turbines.
Energies,
Vol. 14,
Issue. 21,
p.
7060.
Nakamura, Taichi
Fukami, Kai
Hasegawa, Kazuto
Nabae, Yusuke
and
Fukagata, Koji
2021.
Convolutional neural network and long short-term memory based reduced order surrogate for minimal turbulent channel flow.
Physics of Fluids,
Vol. 33,
Issue. 2,
Wang, Hongping
Yang, Zixuan
Wu, Ting
and
Wang, Shizhao
2021.
Coherent structures associated with interscale energy transfer in turbulent channel flows.
Physical Review Fluids,
Vol. 6,
Issue. 10,
Frihat, Mohamed
Podvin, Bérengère
Mathelin, Lionel
Fraigneau, Yann
and
Yvon, François
2021.
Coherent structure identification in turbulent channel flow using latent Dirichlet allocation.
Journal of Fluid Mechanics,
Vol. 920,
Issue. ,
Kristo, Paul J.
Kimber, Mark L.
and
Girimaji, Sharath S.
2021.
Towards Reconstruction of Complex Flow Fields Using Unit Flows.
Fluids,
Vol. 6,
Issue. 7,
p.
255.
Onori, Michel
and
Hills, Nicholas J.
2021.
Reduced order modelling for a rotor-stator cavity using proper orthogonal decomposition.
Computers & Fluids,
Vol. 216,
Issue. ,
p.
104818.
Nekkanti, Akhil
and
Schmidt, Oliver T.
2021.
Frequency–time analysis, low-rank reconstruction and denoising of turbulent flows using SPOD.
Journal of Fluid Mechanics,
Vol. 926,
Issue. ,
Belkadi, Mebarek
Sergent, Anne
Fraigneau, Yann
and
Podvin, Bérengère
2021.
On the role of roughness valleys in turbulent Rayleigh–Bénard convection.
Journal of Fluid Mechanics,
Vol. 923,
Issue. ,
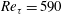 $Re_{\unicode[STIX]{x1D70F}}=590$), so that empirical eigenfunctions are defined in both space and time. Due to the statistical symmetries of the flow, the eigenfunctions are associated with individual wavenumbers and frequencies. Self-similarity of the dominant eigenfunctions, consistent with wall-attached structures transferring energy into the core region, is established. The most energetic modes are characterized by a fundamental time scale in the range 200–300 viscous wall units. The full spatio-temporal decomposition provides a natural measure of the convection velocity of structures, with a characteristic value of 12
$Re_{\unicode[STIX]{x1D70F}}=590$), so that empirical eigenfunctions are defined in both space and time. Due to the statistical symmetries of the flow, the eigenfunctions are associated with individual wavenumbers and frequencies. Self-similarity of the dominant eigenfunctions, consistent with wall-attached structures transferring energy into the core region, is established. The most energetic modes are characterized by a fundamental time scale in the range 200–300 viscous wall units. The full spatio-temporal decomposition provides a natural measure of the convection velocity of structures, with a characteristic value of 12 $u_{\unicode[STIX]{x1D70F}}$ in the wall layer. Finally, we show that the energy budget can be split into specific contributions for each mode, which provides a closed-form expression for nonlinear effects.
$u_{\unicode[STIX]{x1D70F}}$ in the wall layer. Finally, we show that the energy budget can be split into specific contributions for each mode, which provides a closed-form expression for nonlinear effects.