Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Cordasco, Daniel
Yazdani, Alireza
and
Bagchi, Prosenjit
2014.
Comparison of erythrocyte dynamics in shear flow under different stress-free configurations.
Physics of Fluids,
Vol. 26,
Issue. 4,
Cordasco, Daniel
and
Bagchi, Prosenjit
2014.
Intermittency and synchronized motion of red blood cell dynamics in shear flow.
Journal of Fluid Mechanics,
Vol. 759,
Issue. ,
p.
472.
Kaoui, Badr
Jonk, Ruben J. W.
and
Harting, Jens
2014.
Interplay between microdynamics and macrorheology in vesicle suspensions.
Soft Matter,
Vol. 10,
Issue. 26,
p.
4735.
Thiébaud, Marine
Shen, Zaiyi
Harting, Jens
and
Misbah, Chaouqi
2014.
Prediction of Anomalous Blood Viscosity in Confined Shear Flow.
Physical Review Letters,
Vol. 112,
Issue. 23,
Peng, Zhangli
Mashayekh, Adel
and
Zhu, Qiang
2014.
Erythrocyte responses in low-shear-rate flows: effects of non-biconcave stress-free state in the cytoskeleton.
Journal of Fluid Mechanics,
Vol. 742,
Issue. ,
p.
96.
Fischer, Thomas M.
and
Korzeniewski, Rafal
2015.
Angle of Inclination of Tank-Treading Red Cells: Dependence on Shear Rate and Suspending Medium.
Biophysical Journal,
Vol. 108,
Issue. 6,
p.
1352.
Peng, Zhangli
Salehyar, Sara
and
Zhu, Qiang
2015.
Stability of the tank treading modes of erythrocytes and its dependence on cytoskeleton reference states.
Journal of Fluid Mechanics,
Vol. 771,
Issue. ,
p.
449.
Sinha, Kushal
and
Graham, Michael D.
2015.
Dynamics of a single red blood cell in simple shear flow.
Physical Review E,
Vol. 92,
Issue. 4,
Barthès-Biesel, Dominique
2016.
Motion and Deformation of Elastic Capsules and Vesicles in Flow.
Annual Review of Fluid Mechanics,
Vol. 48,
Issue. 1,
p.
25.
Dupont, C.
Delahaye, F.
Barthès-Biesel, D.
and
Salsac, A.-V.
2016.
Stable equilibrium configurations of an oblate capsule in simple shear flow.
Journal of Fluid Mechanics,
Vol. 791,
Issue. ,
p.
738.
Salehyar, Sara
and
Zhu, Qiang
2016.
Deformation and internal stress in a red blood cell as it is driven through a slit by an incoming flow.
Soft Matter,
Vol. 12,
Issue. 13,
p.
3156.
Levant, Michael
and
Steinberg, Victor
2016.
Intermediate regime and a phase diagram of red blood cell dynamics in a linear flow.
Physical Review E,
Vol. 94,
Issue. 6,
Zhang, Hengdi
Shen, Zaiyi
Hogan, Brenna
Barakat, Abdul I.
and
Misbah, Chaouqi
2018.
ATP Release by Red Blood Cells under Flow: Model and Simulations.
Biophysical Journal,
Vol. 115,
Issue. 11,
p.
2218.
Aarab, A.
Guedda, M.
Alaa, N.
and
Misbah, C.
2018.
Mapping vesicle dynamics onto that of a rigid sphere in five dimensions.
Physical Review E,
Vol. 98,
Issue. 4,
Farutin, Alexander
Shen, Zaiyi
Prado, Gael
Audemar, Vassanti
Ez-Zahraouy, Hamid
Benyoussef, Abdelilah
Polack, Benoit
Harting, Jens
Vlahovska, Petia M.
Podgorski, Thomas
Coupier, Gwennou
and
Misbah, Chaouqi
2018.
Optimal cell transport in straight channels and networks.
Physical Review Fluids,
Vol. 3,
Issue. 10,
Minetti, C.
Audemar, V.
Podgorski, T.
and
Coupier, G.
2019.
Dynamics of a large population of red blood cells under shear flow.
Journal of Fluid Mechanics,
Vol. 864,
Issue. ,
p.
408.
Zhang, Hengdi
and
Misbah, Chaouqi
2019.
Lattice Boltzmann simulation of advection-diffusion of chemicals and applications to blood flow.
Computers & Fluids,
Vol. 187,
Issue. ,
p.
46.
Gou, Zhe
Ruan, Xiaodong
Huang, Feng
and
Fu, Xin
2019.
Effects of membrane reference state on shape memory of a red blood cell.
Computer Methods in Biomechanics and Biomedical Engineering,
Vol. 22,
Issue. 5,
p.
465.
Wälchli, Daniel
Martin, Sergio M.
Economides, Athena
Amoudruz, Lucas
Arampatzis, George
Bian, Xin
and
Koumoutsakos, Petros
2020.
Load Balancing in Large Scale Bayesian Inference.
p.
1.
Kaoui, Badr
2020.
Algorithm to implement unsteady jump boundary conditions within the lattice Boltzmann method.
The European Physical Journal E,
Vol. 43,
Issue. 4,
 ${\dot {\gamma } }_{t} $) the red cells rotate with little change of their resting shape. Above that value they become elongated and aligned in the shear field. We measured
${\dot {\gamma } }_{t} $) the red cells rotate with little change of their resting shape. Above that value they become elongated and aligned in the shear field. We measured 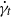 ${\dot {\gamma } }_{t} $ at viscosities (
${\dot {\gamma } }_{t} $ at viscosities ( ${\eta }_{0} $) ranging from 10.7 to 104 mPa s via observation along the vorticity of a Poiseuille flow in a glass capillary;
${\eta }_{0} $) ranging from 10.7 to 104 mPa s via observation along the vorticity of a Poiseuille flow in a glass capillary; 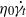 ${\eta }_{0} {\dot {\gamma } }_{t} $ decreased steeply with increasing
${\eta }_{0} {\dot {\gamma } }_{t} $ decreased steeply with increasing 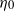 ${\eta }_{0} $ up to a value of 25 mPa s and remained constant for higher values. Present theoretical models are not in keeping with the measured data. Modifications of basic model assumptions are suggested.
${\eta }_{0} $ up to a value of 25 mPa s and remained constant for higher values. Present theoretical models are not in keeping with the measured data. Modifications of basic model assumptions are suggested.