Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-29T11:22:01.699Z Has data issue: false hasContentIssue false
  • English
  • Français

Azimuthal velocity profiles in Rayleigh-stable Taylor–Couette flow and implied axial angular momentum transport

Published online by Cambridge University Press:  09 June 2015

Freja Nordsiek Open the ORCID record for Freja Nordsiek [Opens in a new window] ,
Sander G. Huisman ,
Roeland C. A. van der Veen ,
Chao Sun ,
Detlef Lohse  and
Daniel P. Lathrop
Freja Nordsiek
Affiliation:
Department of Physics and Institute for Research in Electronics and Applied Physics, University of Maryland, College Park, MD 20742, USA
Sander G. Huisman
Affiliation:
Physics of Fluids Group, Faculty of Science and Technology, MESA+ Institute, and Burgers Center for Fluid Dynamics, University of Twente, 7500AE Enschede, The Netherlands
Roeland C. A. van der Veen
Affiliation:
Physics of Fluids Group, Faculty of Science and Technology, MESA+ Institute, and Burgers Center for Fluid Dynamics, University of Twente, 7500AE Enschede, The Netherlands
Chao Sun*
Affiliation:
Physics of Fluids Group, Faculty of Science and Technology, MESA+ Institute, and Burgers Center for Fluid Dynamics, University of Twente, 7500AE Enschede, The Netherlands
Detlef Lohse
Affiliation:
Physics of Fluids Group, Faculty of Science and Technology, MESA+ Institute, and Burgers Center for Fluid Dynamics, University of Twente, 7500AE Enschede, The Netherlands
Daniel P. Lathrop
Affiliation:
Department of Physics and Institute for Research in Electronics and Applied Physics, University of Maryland, College Park, MD 20742, USA
*
†Email address for correspondence: c.sun@utwente.nl
Get access Rights & Permissions [Opens in a new window]

Abstract

We present azimuthal velocity profiles measured in a Taylor–Couette apparatus, which has been used as a model of stellar and planetary accretion disks. The apparatus has a cylinder radius ratio of 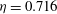${\it\eta}=0.716$, an aspect ratio of 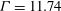${\it\Gamma}=11.74$, and the plates closing the cylinders in the axial direction are attached to the outer cylinder. We investigate angular momentum transport and Ekman pumping in the Rayleigh-stable regime. This regime is linearly stable and is characterized by radially increasing specific angular momentum. We present several Rayleigh-stable profiles for shear Reynolds numbers 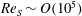$\mathit{Re}_{S}\sim O(10^{5})$, for both 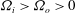${\it\Omega}_{i}>{\it\Omega}_{o}>0$ (quasi-Keplerian regime) and 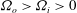${\it\Omega}_{o}>{\it\Omega}_{i}>0$ (sub-rotating regime), where ${\it\Omega}_{i,o}$ is the inner/outer cylinder rotation rate. None of the velocity profiles match the non-vortical laminar Taylor–Couette profile. The deviation from that profile increases as solid-body rotation is approached at fixed 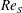$\mathit{Re}_{S}$. Flow super-rotation, an angular velocity greater than those of both cylinders, is observed in the sub-rotating regime. The velocity profiles give lower bounds for the torques required to rotate the inner cylinder that are larger than the torques for the case of laminar Taylor–Couette flow. The quasi-Keplerian profiles are composed of a well-mixed inner region, having approximately constant angular momentum, connected to an outer region in solid-body rotation with the outer cylinder and attached axial boundaries. These regions suggest that the angular momentum is transported axially to the axial boundaries. Therefore, Taylor–Couette flow with closing plates attached to the outer cylinder is an imperfect model for accretion disk flows, especially with regard to their stability.

JFM classification

Instability: Nonlinear instability Geophysical and Geological Flows: Rotating flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 774 , 10 July 2015 , pp. 342 - 362
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Avila, M. 2012 Stability and angular-momentum transport of fluid flows between corotating cylinders. Phys. Rev. Lett. 108 (12), 124501.Google Scholar
Avila, K., Moxey, D., de Lozar, A., Avila, M., Barkley, D. & Hof, B. 2011 The onset of turbulence in pipe flow. Science 333, 192–196.Google Scholar
Balbus, S. A. 2011 Fluid dynamics: a turbulent matter. Nature 470, 475–476.Google Scholar
Borrero-Echeverry, D., Schatz, M. F. & Tagg, R. 2010 Transient turbulence in Taylor–Couette flow. Phys. Rev. E 81 (2), 025301.Google Scholar
Burin, M. J. & Czarnocki, C. J. 2012 Subcritical transition and spiral turbulence in circular Couette flow. J. Fluid Mech. 709, 106–122.Google Scholar
Coles, D. 1965 Transition in circular Couette flow. J. Fluid Mech. 21, 385–425.Google Scholar
Dubrulle, B., Dauchot, O., Daviaud, F., Longaretti, P.-Y., Richard, D. & Zahn, J.-P. 2005a Stability and turbulent transport in Taylor–Couette flow from analysis of experimental data. Phys. Fluids 17 (9), 095103.Google Scholar
Dubrulle, B., Marié, L., Normand, C., Richard, D., Hersant, F. & Zahn, J.-P. 2005b An hydrodynamic shear instability in stratified disks. Astron. Astrophys. 429, 1–13.Google Scholar
Dunst, M. 1972 An experimental and analytical investigation of angular momentum exchange in a rotating fluid. J. Fluid Mech. 55, 301–310.CrossRefGoogle Scholar
Eckhardt, B., Grossmann, S. & Lohse, D. 2007 Torque scaling in turbulent Taylor Couette flow between independently rotating cylinders. J. Fluid Mech. 581, 221–250.CrossRefGoogle Scholar
Edlund, E. M. & Ji, H. 2014 Nonlinear stability of laboratory quasi-Keplerian flows. Phys. Rev. E 89 (2), 021004.Google Scholar
Grossmann, S. 2000 The onset of shear flow turbulence. Rev. Mod. Phys. 72, 603–618.Google Scholar
van Gils, D. P. M., Bruggert, G.-W., Lathrop, D. P., Sun, C. & Lohse, D. 2011a The Twente turbulent Taylor–Couette (T3C) facility: strongly turbulent (multiphase) flow between two independently rotating cylinders. Rev. Sci. Instrum. 82 (2), 025105.Google Scholar
van Gils, D. P. M., Huisman, S. G., Bruggert, G.-W., Sun, C. & Lohse, D. 2011b Torque scaling in turbulent Taylor–Couette flow with co- and counterrotating cylinders. Phys. Rev. Lett. 106 (2), 024502.Google Scholar
van Gils, D. P. M., Huisman, S. G., Grossmann, S., Sun, C. & Lohse, D. 2012 Optimal Taylor–Couette turbulence. J. Fluid Mech. 706, 118–149.CrossRefGoogle Scholar
Huisman, S. G., van Gils, D. P. M., Grossmann, S., Sun, C. & Lohse, D. 2012a Ultimate turbulent Taylor–Couette flow. Phys. Rev. Lett. 108 (2), 024501.Google Scholar
Huisman, S. G., van Gils, D. P. M. & Sun, C. 2012b Applying laser Doppler anemometry inside a Taylor–Couette geometry using a ray-tracer to correct for curvature effects. Eur. J. Mech. (B/Fluids) 36, 115–119.Google Scholar
Huisman, S. G., Scharnowski, S., Cierpka, C., Kähler, C. J., Lohse, D. & Sun, C. 2013 Logarithmic boundary layers in strong Taylor–Couette turbulence. Phys. Rev. Lett. 110 (26), 264501.Google Scholar
Ji, H. & Balbus, S. 2013 Angular momentum transport in astrophysics and in the lab. Phys. Today 66 (8), 27–33.Google Scholar
Ji, H., Burin, M., Schartman, E. & Goodman, J. 2006 Hydrodynamic turbulence cannot transport angular momentum effectively in astrophysical disks. Nature 444, 343–346.Google Scholar
Kageyama, A., Ji, H., Goodman, J., Chen, F. & Shoshan, E. 2004 Numerical and experimental investigation of circulation in short cylinders. J. Phys. Soc. Japan 73, 2424–2437.Google Scholar
Landau, L. D. & Lifshitz, E. M. 1987 Fluid Mechanics, 2nd edn. Course of Theoretical Physics, vol. 6. Pergamon.Google Scholar
Lathrop, D. P., Fineberg, J. & Swinney, H. L. 1992 Transition to shear-driven turbulence in Couette–Taylor flow. Phys. Rev. A 46, 6390–6405.Google Scholar
Le Bars, M. & Le Gal, P. 2007 Experimental analysis of the stratorotational instability in a cylindrical Couette flow. Phys. Rev. Lett. 99 (6), 064502.Google Scholar
Le Dizès, S. & Riedinger, X. 2010 The strato-rotational instability of Taylor–Couette and Keplerian flows. J. Fluid Mech. 660, 147–161.Google Scholar
Maretzke, S., Hof, B. & Avila, M. 2014 Transient growth in linearly stable Taylor–Couette flows. J. Fluid Mech. 742, 254–290.Google Scholar
Ostilla-Mónico, R., Verzicco, R., Grossmann, S. & Lohse, D. 2014 Turbulence decay towards the linearly stable regime of Taylor–Couette flow. J. Fluid Mech. 748, R3.Google Scholar
Paoletti, M. S., van Gils, D. P. M., Dubrulle, B., Sun, C., Lohse, D. & Lathrop, D. P. 2012 Angular momentum transport and turbulence in laboratory models of Keplerian flows. Astron. Astrophys. 547, A64.CrossRefGoogle Scholar
Paoletti, M. S. & Lathrop, D. P. 2011 Angular momentum transport in turbulent flow between independently rotating cylinders. Phys. Rev. Lett. 106 (2), 024501.Google Scholar
Rayleigh, L. 1917 On the dynamics of revolving fluids. Proc. R. Soc. Lond. A 93 (648), 148–154.Google Scholar
Richard, D.2001 Instabilités hydrodynamiques dans les écoulements en rotation différentielle. PhD thesis, Université Paris-Diderot – Paris VII.Google Scholar
Richard, D. & Zahn, J.-P. 1999 Turbulence in differentially rotating flows. What can be learned from the Couette–Taylor experiment. Astron. Astrophys. 347, 734–738.Google Scholar
Schartman, E., Ji, H., Burin, M. J. & Goodman, J. 2012 Stability of quasi-Keplerian shear flow in a laboratory experiment. Astron. Astrophys. 543, A94.Google Scholar
Schlichting, H. T. 1979 Boundary Layer Theory. McGraw-Hill.Google Scholar
Shi, L., Avila, M. & Hof, B. 2013 Scale invariance at the onset of turbulence in Couette flow. Phys. Rev. Lett. 110 (20), 204502.CrossRefGoogle ScholarPubMed
Taylor, G. I. 1923 Stability of a viscous liquid contained between two rotating cylinders. Phil. Trans. R. Soc. Lond. A 223, 289–343.Google Scholar
Taylor, G. I. 1936a Fluid friction between rotating cylinders. I. Torque measurements. Proc. R. Soc. Lond. A 157 (892), 546–564.Google Scholar
Taylor, G. I. 1936b Fluid friction between rotating cylinders. II. Distribution of velocity between concentric cylinders when outer one is rotating and inner one is at rest. Proc. R. Soc. Lond. A 157, 565–578.Google Scholar
Wendt, F. 1933 Turbulente Strömungen zwischen zwei rotierenden konaxialen Zylindern. Ing. Arch. Suisses 4, 577–595.CrossRefGoogle Scholar
Zeldovich, Y. B. 1981 On the friction of fluids between rotating cylinders. Proc. R. Soc. Lond. A 374, 299–312.Google Scholar
Supplementary material: File

Nordsiek supplementary material

Nordsiek supplementary data

Download Nordsiek supplementary material(File)
File 18.8 KB