Published online by Cambridge University Press: 04 October 2023
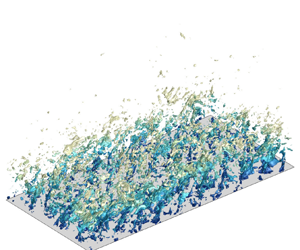
In the present study, we investigate the compressibility effects in supersonic and hypersonic turbulent boundary layers under the influence of wall disturbances by exploiting direct numerical simulation databases at Mach numbers up to 6. Such wall disturbances enforce extra Reynolds shear stress on the wall and induce mean streamline curvature in rough wall turbulence that leads to the intensification of turbulent motions in the outer region. The turbulent and fluctuating Mach numbers, the density and the velocity divergence fluctuation intensities suggest that the compressibility effects are enhanced by the increment of the free-stream Mach number and the implementation of the wall disturbances. The differences between the Reynolds and Favre average due to the density fluctuations constitute approximately  $9\,\%$ of the mean velocity close to the wall and
$9\,\%$ of the mean velocity close to the wall and  $30\,\%$ of the Reynolds stress near the edge of the boundary layer, indicating their non-negligibility in turbulent modelling strategies. The comparatively strong compressive events behaving as eddy shocklets are observed at the free-stream Mach number of
$30\,\%$ of the Reynolds stress near the edge of the boundary layer, indicating their non-negligibility in turbulent modelling strategies. The comparatively strong compressive events behaving as eddy shocklets are observed at the free-stream Mach number of  $6$ only in the cases with wall disturbances. By further splitting the velocity into the solenoidal and dilatational components with the Helmholtz decomposition, we found that the dilatational motions are organized as travelling wave packets in the wall-parallel planes close to the wall and as forward inclined structures in the form of radiated waves in the vertical planes. Despite their increased magnitudes and higher portion in the Reynolds normal and shear stresses, the dilatational motions show no tendency of contributing significantly to the skin friction and the production of turbulent kinetic energy due to their mitigation by the cross-correlation between the solenoidal and dilatational velocity components.
$6$ only in the cases with wall disturbances. By further splitting the velocity into the solenoidal and dilatational components with the Helmholtz decomposition, we found that the dilatational motions are organized as travelling wave packets in the wall-parallel planes close to the wall and as forward inclined structures in the form of radiated waves in the vertical planes. Despite their increased magnitudes and higher portion in the Reynolds normal and shear stresses, the dilatational motions show no tendency of contributing significantly to the skin friction and the production of turbulent kinetic energy due to their mitigation by the cross-correlation between the solenoidal and dilatational velocity components.