Article contents
Compressible Taylor–Couette flow – instability mechanism and codimension 3 points
Published online by Cambridge University Press: 10 June 2014
Abstract
Taylor–Couette flow in a compressible perfect gas is studied. The onset of instability is examined as a function of the Reynolds numbers of the inner and outer cylinder, the Mach number of the flow and the Prandtl number of the gas. We focus on the case of a wide gap, with radius ratio 0.5. We find new modes of instability at high Prandtl number, which can allow oscillatory axisymmetric modes to onset first. We also find that onset can occur even when the angular momentum increases outwards, so that the classical Rayleigh criterion can be violated in the compressible case. We have also considered the case of counter-rotating cylinders, where the 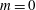 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}m=0$ and
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}m=0$ and 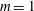 $m=1$ modes can onset simultaneously to give a codimension 2 bifurcation, leading to the formation of complex flow patterns. In compressible flow we also find codimension 3 points. The Mach number and the critical inner and outer Reynolds numbers can be adjusted so that the two neutral curves for the
$m=1$ modes can onset simultaneously to give a codimension 2 bifurcation, leading to the formation of complex flow patterns. In compressible flow we also find codimension 3 points. The Mach number and the critical inner and outer Reynolds numbers can be adjusted so that the two neutral curves for the 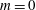 $m=0$ and
$m=0$ and 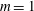 $m=1$ modes touch rather than cross. Complex codimension 3 points occur more readily in the compressible case than in the Boussinesq case, and they are expected to lead to a rich nonlinear behaviour.
$m=1$ modes touch rather than cross. Complex codimension 3 points occur more readily in the compressible case than in the Boussinesq case, and they are expected to lead to a rich nonlinear behaviour.
JFM classification
Information
- Type
- Papers
- Information
- Creative Commons
- This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/3.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
- Copyright
- © 2014 Cambridge University Press
References
- 5
- Cited by

