Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Suzzi, Nicola
and
Croce, Giulio
2021.
Numerical Bifurcation Analysis of a Film Flowing over a Patterned Surface through Enhanced Lubrication Theory.
Fluids,
Vol. 6,
Issue. 11,
p.
405.
Leng, X.-Y.
Krasnov, D.
Li, B.-W.
and
Zhong, J.-Q.
2021.
Flow structures and heat transport in Taylor–Couette systems with axial temperature gradient.
Journal of Fluid Mechanics,
Vol. 920,
Issue. ,
Alghamdi, Abdulaziz S.
Abdel Halim, K. S.
Amin, Mohammed A.
Alshammari, Abdullah S.
Fathy, Naglaa
and
Ramadan, Mohamed
2021.
Interfacial Microstructure and Corrosion Behaviour of Mild Steel Coated with Alumina Nanoparticles Doped Tin Composite via Direct Tinning Route.
Coatings,
Vol. 11,
Issue. 11,
p.
1318.
Leng, X.-Y.
and
Zhong, J.-Q.
2022.
Mutual coherent structures for heat and angular momentum transport in turbulent Taylor-Couette flows.
Physical Review Fluids,
Vol. 7,
Issue. 4,
Voinov, Nikolai A.
Frolov, Alexander S.
Bogatkova, Anastasiya V.
and
Zemtsov, Denis A.
2022.
Experimental Study and Numerical Simulation of Hydrodynamic Parameters of Tangential Swirlers.
ChemEngineering,
Vol. 6,
Issue. 4,
p.
48.
Zhang, Shengqi
Xia, Zhenhua
and
Chen, Shiyi
2022.
Flow structures in spanwise rotating plane Poiseuille flow based on thermal analogy.
Journal of Fluid Mechanics,
Vol. 933,
Issue. ,
Hu, Lehao
Deng, Qinghua
Li, Jun
and
Feng, Zhenping
2022.
Model improvement for shaft-type windage loss with CO2.
The Journal of Supercritical Fluids,
Vol. 190,
Issue. ,
p.
105747.
Xie, Zhongliang
Chen, Rugang
and
Zhu, Weidong
2022.
An insight into the flow field characteristics of the high temperature liquid Sodium (Na) with cavitation effects in the 600 MW fast reactor system.
Annals of Nuclear Energy,
Vol. 165,
Issue. ,
p.
108706.
Zou, Yucheng
You, Chenxi
Tan, Xiangkui
Wang, Yiwei
Wang, Jingzhu
Li, Chaohui
He, Ming
Lv, Kai
Zou, Yong
Song, Huaitong
Lv, Pengyu
and
Li, Hongyuan
2023.
Design and implementation of a gliding cross-domain vehicle.
Ocean Engineering,
Vol. 280,
Issue. ,
p.
114549.
2023.
Effect of micro-grooves on drag reduction in Taylor–Couette flow.
Physics of Fluids,
Vol. 35,
Issue. 4,
Jeganathan, Vignesh
Shannak, Tala
Alba, Kamran
and
Ostilla-Mónico, Rodolfo
2023.
Controlling secondary flows in Taylor–Couette flow using axially spaced superhydrophobic surfaces.
Journal of Fluid Mechanics,
Vol. 969,
Issue. ,
Zhang, Yuhan
Xu, Rui
Song, Yuchen
Wu, Dazhuan
and
Wu, Peng
2024.
Numerical simulation of turbulent Taylor–Couette flow with high Taylor number and large radius ratio in high-speed canned motor pump internals.
Physics of Fluids,
Vol. 36,
Issue. 10,
Shi, Lijuan
Wang, Meng
Tang, Zhanqi
and
Jiang, Nan
2024.
Bursting-process modulation in a turbulent boundary layer via the natural visibility graph method.
Physics of Fluids,
Vol. 36,
Issue. 3,
Zhang, Yuhan
He, Xinxiang
Wu, Dazhuan
Shen, Yuzhe
Lu, Yu
and
Xu, Rui
2024.
Study on Turbulent Taylor-Couette Flow with High Reynolds Number and Large Radius Ratio in High-Speed Canned Motor Pump Internals.
Journal of Physics: Conference Series,
Vol. 2854,
Issue. 1,
p.
012017.
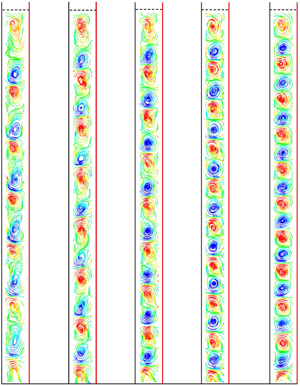
 $d$ between the inner rotating and outer stationary cylinders through manipulating the initial height of the water annulus. We show that the torque exerted on the inner cylinder of the Taylor–Couette system can be reduced by up to 20 % by controlling the flow at a state where fewer than the nominal number of vortices develop between the cylinders. This flow state is achieved by starting the system with an initial water annulus height
$d$ between the inner rotating and outer stationary cylinders through manipulating the initial height of the water annulus. We show that the torque exerted on the inner cylinder of the Taylor–Couette system can be reduced by up to 20 % by controlling the flow at a state where fewer than the nominal number of vortices develop between the cylinders. This flow state is achieved by starting the system with an initial water annulus height  $h_0$ (which nominally corresponds to
$h_0$ (which nominally corresponds to  $h_0/d$ vortices), then gradually adding water into the annulus while the inner cylinder keeps rotating. During this filling process the flow topology is so persistent that the number of vortices does not increase; instead, the vortices are greatly stretched in the axial (vertical) direction. We show that this state with stretched vortices is sustainable until the vortices are stretched to around 2.05 times their nominal size. Our experiments reveal that by manipulating the initial height of the liquid annulus we are able to generate different flow states and demonstrate how the different flow states manifest themselves in global momentum transport.
$h_0/d$ vortices), then gradually adding water into the annulus while the inner cylinder keeps rotating. During this filling process the flow topology is so persistent that the number of vortices does not increase; instead, the vortices are greatly stretched in the axial (vertical) direction. We show that this state with stretched vortices is sustainable until the vortices are stretched to around 2.05 times their nominal size. Our experiments reveal that by manipulating the initial height of the liquid annulus we are able to generate different flow states and demonstrate how the different flow states manifest themselves in global momentum transport.