Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Pereira, G. G.
and
Cleary, P. W.
2013.
Radial segregation of multi-component granular media in a rotating tumbler.
Granular Matter,
Vol. 15,
Issue. 6,
p.
705.
Fan, Yi
Schlick, Conor P.
Umbanhowar, Paul B.
Ottino, Julio M.
and
Lueptow, Richard M.
2014.
Modelling size segregation of granular materials: the roles of segregation, advection and diffusion.
Journal of Fluid Mechanics,
Vol. 741,
Issue. ,
p.
252.
Tunuguntla, D. R.
Bokhove, O.
and
Thornton, A. R.
2014.
A mixture theory for size and density segregation in shallow granular free-surface flows.
Journal of Fluid Mechanics,
Vol. 749,
Issue. ,
p.
99.
Hill, K. M.
and
Tan, Danielle S.
2014.
Segregation in dense sheared flows: gravity, temperature gradients, and stress partitioning.
Journal of Fluid Mechanics,
Vol. 756,
Issue. ,
p.
54.
Gray, John Mark Nicholas Timm
Gajjar, Parmesh
and
Kokelaar, Peter
2015.
Particle-size segregation in dense granular avalanches
.
Comptes Rendus. Physique,
Vol. 16,
Issue. 1,
p.
73.
Gray, J. M. N. T.
and
Ancey, C.
2015.
Particle-size and -density segregation in granular free-surface flows.
Journal of Fluid Mechanics,
Vol. 779,
Issue. ,
p.
622.
Fan, Yi
Umbanhowar, Paul B.
Ottino, Julio M.
and
Lueptow, Richard M.
2015.
Shear-Rate-Independent Diffusion in Granular Flows.
Physical Review Letters,
Vol. 115,
Issue. 8,
Fan, Yi
and
Hill, K. M.
2015.
Shear-induced segregation of particles by material density.
Physical Review E,
Vol. 92,
Issue. 2,
Liao, C.C.
Hsiau, S.S.
and
Nien, H.C.
2015.
Effects of density ratio, rotation speed, and fill level on density-induced granular streak segregation in a rotating drum.
Powder Technology,
Vol. 284,
Issue. ,
p.
514.
Hill, Kimberly M.
and
Fan, Yi
2016.
Granular Temperature and Segregation in Dense Sheared Particulate Mixtures.
KONA Powder and Particle Journal,
Vol. 33,
Issue. 0,
p.
150.
Sun, Jing
Liu, Chuanping
Wu, Ping
Xie, Zi-Ang
Hu, Kaiwei
and
Wang, Li
2016.
Granular core phenomenon induced by convection in a vertically vibrated cylindrical container.
Physical Review E,
Vol. 94,
Issue. 3,
Zhou, Wei
Lai, Zhiqiang
Ma, Gang
Yang, Lifu
and
Chen, Yuan
2016.
Effect of base roughness on size segregation in dry granular flows.
Granular Matter,
Vol. 18,
Issue. 4,
Schlick, Conor P.
Isner, Austin B.
Freireich, Ben J.
Fan, Yi
Umbanhowar, Paul B.
Ottino, Julio M.
and
Lueptow, Richard M.
2016.
A continuum approach for predicting segregation in flowing polydisperse granular materials.
Journal of Fluid Mechanics,
Vol. 797,
Issue. ,
p.
95.
Xiao, Hongyi
Umbanhowar, Paul B.
Ottino, Julio M.
and
Lueptow, Richard M.
2016.
Modelling density segregation in flowing bidisperse granular materials.
Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 472,
Issue. 2191,
p.
20150856.
Liao, Chun-Chung
Lan, Hung-Wen
and
Hsiau, Shu-San
2016.
Density-induced granular segregation in a slurry rotating drum.
International Journal of Multiphase Flow,
Vol. 84,
Issue. ,
p.
1.
Combarros Garcia, M.
Feise, H.J.
Strege, S.
and
Kwade, A.
2016.
Segregation in heaps and silos: Comparison between experiment, simulation and continuum model.
Powder Technology,
Vol. 293,
Issue. ,
p.
26.
Motanated, Kannipa
and
Tice, Michael M.
2016.
Hydraulic evolution of high-density turbidity currents from the Brushy Canyon Formation, Eddy County, New Mexico inferred by comparison to settling and sorting experiments.
Sedimentary Geology,
Vol. 337,
Issue. ,
p.
69.
Liu, Siying
and
McCarthy, Joseph J.
2017.
Transport analogy for segregation and granular rheology.
Physical Review E,
Vol. 96,
Issue. 2,
Tripathi, Anurag
Nema, Mohit
Radjai, F.
Nezamabadi, S.
Luding, S.
and
Delenne, J.Y.
2017.
Size Segregation of Binary Granular Mixtures - Towards more appropriate scaling of percolation velocity.
EPJ Web of Conferences,
Vol. 140,
Issue. ,
p.
03082.
Liao, Chun-Chung
Hsiau, Shu-San
and
Hu, Yu-Ming
2017.
Density-driven sinking dynamics of a granular ring in sheared granular flows.
Advanced Powder Technology,
Vol. 28,
Issue. 10,
p.
2597.
 $\phi $. The buoyancy arises primarily from normal forces acting on the particle, and tangential forces have a negligible contribution. The drag force on a sphere of diameter
$\phi $. The buoyancy arises primarily from normal forces acting on the particle, and tangential forces have a negligible contribution. The drag force on a sphere of diameter 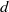 $d$ sinking with a velocity
$d$ sinking with a velocity  $v$ in a granular medium of apparent viscosity
$v$ in a granular medium of apparent viscosity  $\eta $ is given by a modified Stokes law,
$\eta $ is given by a modified Stokes law, 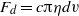 ${F}_{d} = c\pi \eta dv$. The coefficient (
${F}_{d} = c\pi \eta dv$. The coefficient ( $c$) is found to decrease with packing fraction. In the second part of the work, we consider the case of binary granular mixtures of particles of the same size but differing in density. A continuum model for segregation is presented, based on the single-particle results. The number fraction profile for the heavy particles at equilibrium is obtained in terms of the effective temperature, defined by a fluctuation–dissipation relation. The model predicts the equilibrium number fraction profiles at different inclination angles and for different mass ratios of the particles, which match the DEM results very well. Finally, a complete model for the theoretical prediction of the flow and number fraction profiles for a mixture of particles of different density is presented, which combines the segregation model with a model for the rheology of mixtures. The model predictions agree quite well with the simulation results.
$c$) is found to decrease with packing fraction. In the second part of the work, we consider the case of binary granular mixtures of particles of the same size but differing in density. A continuum model for segregation is presented, based on the single-particle results. The number fraction profile for the heavy particles at equilibrium is obtained in terms of the effective temperature, defined by a fluctuation–dissipation relation. The model predicts the equilibrium number fraction profiles at different inclination angles and for different mass ratios of the particles, which match the DEM results very well. Finally, a complete model for the theoretical prediction of the flow and number fraction profiles for a mixture of particles of different density is presented, which combines the segregation model with a model for the rheology of mixtures. The model predictions agree quite well with the simulation results.