Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-29T13:34:33.841Z Has data issue: false hasContentIssue false
  • English
  • Français

Drop squeezing between arbitrary smooth obstacles

Published online by Cambridge University Press:  10 December 2020

Jacob R. Gissinger Open the ORCID record for Jacob R. Gissinger [Opens in a new window] ,
Alexander Z. Zinchenko Open the ORCID record for Alexander Z. Zinchenko [Opens in a new window]  and
Robert H. Davis Open the ORCID record for Robert H. Davis [Opens in a new window]
Jacob R. Gissinger
Affiliation:
Department of Chemical and Biological Engineering, University of Colorado, Boulder, CO80309-0424, USA
Alexander Z. Zinchenko*
Affiliation:
Department of Chemical and Biological Engineering, University of Colorado, Boulder, CO80309-0424, USA
Robert H. Davis*
Affiliation:
Department of Chemical and Biological Engineering, University of Colorado, Boulder, CO80309-0424, USA
*
†Email addresses for correspondence: alexander.zinchenko@colorado.edu, robert.davis@colorado.edu
†Email addresses for correspondence: alexander.zinchenko@colorado.edu, robert.davis@colorado.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

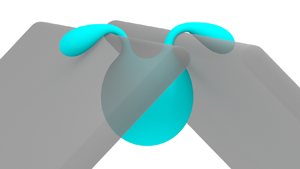
A fully three-dimensional boundary-integral method (BIM) is developed for the interaction of drops, suspended in a uniform far-field flow at small Reynolds number, with arbitrary Lyapunov surfaces. The close approach of fluid interfaces to solid surfaces poses significant challenges for numerical BIM implementations, due to the highly singular behaviour of single- and double-layer boundary integrals. Two new methods are described that generalize the accurate calculation of the highly singular surface integrals used by high-order desingularization techniques. The first method is semi-analytical, and applies to axisymmetric solid obstacles (in an arbitrary three-dimensional configuration). An axisymmetric particle can be divided into a series of characteristic disks along its axis, for which closed-form expressions for single and double layers are derived in terms of elliptic integrals. To accommodate arbitrary smooth surfaces, a multimesh desingularization method is introduced that calculates surface integrals utilizing a hierarchy of embedded mesh resolutions, together with distance-activated mesh interactions. Several particle shapes, including spherocylinders (capsules) and flat plates, are used to represent major classes characteristic of porous media. A droplet approaching a capsule will break up after forming two lobes, connected by a thin filament, on either side of the capsule. The cross-sectional shape of the filament affects lubrication behaviour. A constriction made of two parallel capsules, even of low aspect ratio, significantly retards drop passage compared to two spheres. Trends in drop squeezing between two capsules are summarized over a range of capillary number, viscosity ratio, drop size and capsule length. A constriction of two coplanar plates results in notably different lubrication and squeezing behaviour. Flow rectification is demonstrated for constrictions that are non-symmetrical with respect to flow reversal, for several non-axisymmetric particles.

JFM classification

Low-Reynolds-number flows: Boundary integral methods Drops and Bubbles: Drops Low-Reynolds-number flows: Porous media
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 908 , 10 February 2021 , A33
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Al-Abduwani, F. A. H., Farajzadeh, R., van den Broek, W. M. G. T., Currie, P. K. & Zitha, P. L. J. 2005 Filtration of micron-sized particles in granular media revealed by x-ray computed tomography. Rev. Sci. Instrum. 76 (10), 103704.CrossRefGoogle Scholar
Ardekani, A. M., Dabiri, S. & Rangel, R. H. 2009 Deformation of a droplet in a particulate shear flow. Phys. Fluids 21 (9), 093302.CrossRefGoogle Scholar
Blunt, M. J., Bijeljic, B., Dong, H., Gharbi, O., Iglauer, S., Mostaghimi, P., Paluszny, A. & Pentland, C. 2013 Pore-scale imaging and modelling. Adv. Water Resour. 51, 197–216.CrossRefGoogle Scholar
Bordoloi, A. D. & Longmire, E. K. 2014 Drop motion through a confining orifice. J. Fluid Mech. 759, 520–545.CrossRefGoogle Scholar
Bretherton, F. P. 1961 The motion of long bubbles in tubes. J. Fluid Mech. 10 (2), 166–188.CrossRefGoogle Scholar
Cristini, V., Bławzdziewicz, J. & Loewenberg, M. 2001 An adaptive mesh algorithm for evolving surfaces: simulations of drop breakup and coalescence. J. Comput. Phys. 168 (2), 445–463.CrossRefGoogle Scholar
Davis, H. T. 1962 Introduction to Nonlinear Differential and Integral Equations. Dover.Google Scholar
Davis, R. H. 1999 Buoyancy-driven viscous interaction of a rising drop with a smaller trailing drop. Phys. Fluids 11 (5), 1016–1028.CrossRefGoogle Scholar
Dietsche, L. J. & Neubauer, A. C. 2009 Computational fluid dynamics model of viscous droplet breakup. Chem. Engng Sci. 64 (22), 4543–4552.CrossRefGoogle Scholar
Fan, X.-J., Phan-Thien, N. & Zheng, R. 1998 Completed double layer boundary element method for periodic suspensions. Z. Angew. Math. Phys. 49 (2), 167–193.CrossRefGoogle Scholar
Gradshteyn, I. S. & Ryzhik, I. M. 2014 Table of Integrals, Series, and Products. Academic Press.Google Scholar
Hao, L. & Cheng, P. 2010 Pore-scale simulations on relative permeabilities of porous media by lattice Boltzmann method. Intl J. Heat Mass Transfer 53 (9), 1908–1913.CrossRefGoogle Scholar
Herring, A. L., Harper, E. J., Andersson, L., Sheppard, A., Bay, B. K. & Wildenschild, D. 2013 Effect of fluid topology on residual nonwetting phase trapping: implications for geologic CO2 sequestration. Adv. Water Resour. 62, 47–58.CrossRefGoogle Scholar
Huang, L., Mikolajczyk, G., Küstermann, E, Wilhelm, M., Odenbach, S. & Dreher, W. 2017 Adapted MR velocimetry of slow liquid flow in porous media. J. Magn. Reson 276, 103–112.CrossRefGoogle ScholarPubMed
Janssen, P. J. A. & Anderson, P. D. 2008 A boundary-integral model for drop deformation between two parallel plates with non-unit viscosity ratio drops. J. Comput. Phys. 227 (20), 8807–8819.CrossRefGoogle Scholar
Khalili, A. & Liu, B. 2017 Stokes’ paradox: creeping flow past a two-dimensional cylinder in an infinite domain. J. Fluid. Mech. 817, 374–387.CrossRefGoogle Scholar
Kropinski, M. C. A. 1999 Integral equation methods for particle simulations in creeping flows. Comput. Maths Applics. 38 (5), 67–87.CrossRefGoogle Scholar
Krummel, A. T., Datta, S. S., Münster, S. & Weitz, D. A. 2013 Visualizing multiphase flow and trapped fluid configurations in a model three-dimensional porous medium. AIChE J. 59 (3), 1022–1029.CrossRefGoogle Scholar
Lee, S. H. & Leal, L. G. 1982 The motion of a sphere in the presence of a deformable interface: II. A numerical study of the translation of a sphere normal to an interface. J. Colloid Interface Sci. 87 (1), 81–106.CrossRefGoogle Scholar
Mantle, M. D., Sederman, A. J. & Gladden, L. F. 2001 Single- and two-phase flow in fixed-bed reactors: MRI flow visualisation and Lattice-Boltzmann simulations. Chem. Engng Sci. 56 (2), 523–529.CrossRefGoogle Scholar
Oughanem, R., Youssef, S., Bauer, D., Peysson, Y., Maire, E. & Vizika, O. 2015 A multi-scale investigation of pore structure impact on the mobilization of trapped oil by surfactant injection. Transp. Porous Med. 109 (3), 673–692.CrossRefGoogle Scholar
Pak, T., Butler, I. B., Geiger, S., van Dijke, M. I. J. & Sorbie, K. S. 2015 Droplet fragmentation: 3D imaging of a previously unidentified pore-scale process during multiphase flow in porous media. Proc. Natl Acad. Sci. USA 112 (7), 1947–1952.CrossRefGoogle ScholarPubMed
Pan, C., Hilpert, M. & Miller, C. T. 2004 Lattice-Boltzmann simulation of two-phase flow in porous media. Water Resour. Res. 40, W01501.CrossRefGoogle Scholar
Patel, P. D., Shaqfeh, E. S. G., Butler, J. E., Cristini, V., Bławzdziewicz, J. & Loewenberg, M. 2003 Drop breakup in the flow through fixed fiber beds: an experimental and computational investigation. Phys. Fluids 15 (5), 1146–1157.CrossRefGoogle Scholar
Pozrikidis, C. 1992 Boundary Integral and Singularity Methods for Linearized Viscous Flow (Cambridge Texts in Applied Mathematics). Cambridge University Press.CrossRefGoogle Scholar
Rallison, J. M. 1981 A numerical study of the deformation and burst of a viscous drop in general shear flows. J. Fluid Mech. 109, 465–482.CrossRefGoogle Scholar
Rallison, J. M. & Acrivos, A. 1978 A numerical study of the deformation and burst of a viscous drop in an extensional flow. J. Fluid Mech. 89 (1), 191–200.CrossRefGoogle Scholar
Ratcliffe, T., Zinchenko, A. Z. & Davis, R. H. 2010 Buoyancy-induced squeezing of a deformable drop through an axisymmetric ring constriction. Phys. Fluids 22 (8), 082101.CrossRefGoogle Scholar
Ratcliffe, T., Zinchenko, A. Z. & Davis, R. H. 2012 Simulations of gravity-induced trapping of a deformable drop in a three-dimensional constriction. J. Colloid Interface Sci. 383 (1), 167–176.CrossRefGoogle Scholar
Smith, S. H. 1990 The Jeffery paradox as the limit of a three-dimensional Stokes flow. Phys. Fluids A 2 (5), 661–665.CrossRefGoogle Scholar
Yiotis, A. G., Talon, L. & Salin, D. 2013 Blob population dynamics during immiscible two-phase flows in reconstructed porous media. Phys. Rev. E 87 (3), 033001.CrossRefGoogle Scholar
Zarikos, I., Terzis, A., Hassanizadeh, S. M. & Weigand, B. 2018 Velocity distributions in trapped and mobilized non-wetting phase ganglia in porous media. Sci. Rep. 8 (1), 1–11.CrossRefGoogle ScholarPubMed
Zinchenko, A. Z. & Davis, R. H. 2005 A multipole-accelerated algorithm for close interaction of slightly deformable drops. J. Comput. Phys. 207 (2), 695–735.CrossRefGoogle Scholar
Zinchenko, A. Z. & Davis, R. H. 2006 A boundary-integral study of a drop squeezing through interparticle constrictions. J. Fluid. Mech. 564, 227–266.CrossRefGoogle Scholar
Zinchenko, A. Z. & Davis, R. H. 2013 Emulsion flow through a packed bed with multiple drop breakup. J. Fluid. Mech. 725, 611–663.CrossRefGoogle Scholar
Zinchenko, A. Z., Rother, M. A. & Davis, R. H. 1997 A novel boundary-integral algorithm for viscous interaction of deformable drops. Phys. Fluids 9 (6), 1493–1511.CrossRefGoogle Scholar