Hostname: page-component-78c5997874-t5tsf Total loading time: 0 Render date: 2024-11-13T01:55:26.934Z Has data issue: false hasContentIssue false
  • English
  • Français

Dynamics of zonally elongated transient flows

Published online by Cambridge University Press:  03 February 2021

M.V. Rudko Open the ORCID record for M.V. Rudko [Opens in a new window]  and
I.V. Kamenkovich
M.V. Rudko*
Affiliation:
Cooperative Institute for Marine and Atmospheric Studies, University of Miami, Miami, FL 33149, USA Atlantic Oceanic and Meteorological Laboratory, National Oceanographic and Atmospheric Administration, Miami, FL 33149, USA
I.V. Kamenkovich
Affiliation:
Department of Ocean Sciences, RSMAS/University of Miami, Miami, FL 33149, USA
*
†Email address for correspondence: mrudko@miami.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

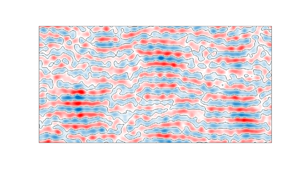
This study examines the dynamics of zonally elongated transient flows (ZELTs) in the context of quasi-geostrophic turbulence. Unlike stationary zonal jets considered in previous studies, these flow features do not span the entire oceanic basin and propagate in the zonal direction at a speed slower than baroclinic Rossby waves. The analysis of potential vorticity balance in a statistically steady state shows that ZELTs are maintained by the vorticity flux divergences associated with the eddy–eddy interactions whereas the eddy–mean flow interactions play a secondary role. The divergences of the eddy fluxes of barotropic relative vorticity and buoyancy are shown to be the dominant contributors. The importance of specific eddy–eddy interactions are further studied using a dynamical model with the removed eddy–eddy vorticity flux divergences, which is equivalent to the fluid system with an absent cascade of eddy energy. Simulations with this reduced-dynamics model exhibit a complete disappearance of ZELTs, confirming the expectations from the analysis of the vorticity balance. Additional reduced-dynamics simulations demonstrate that the eddy kinetic energy transport leads to the emergence of ZELTs, with the transport in the baroclinic mode playing the leading role, while the potential energy transport acts to damp them.

JFM classification

Geophysical and Geological Flows: Baroclinic flows Geophysical and Geological Flows: Geostrophic turbulence Geophysical and Geological Flows: Quasi-geostrophic flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 911 , 25 March 2021 , A61
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Abramov, R.V. & Majda, A.J. 2003 Statistically relevant conserved quantities for truncated quasigeostrophic flow. Proc. Natl Acad. Sci. USA 100 (7), 3841–3846.CrossRefGoogle ScholarPubMed
Berloff, P. & Kamenkovich, I. 2013 a On spectral analysis of mesoscale eddies. Part I: linear analysis. J. Phys. Oceanogr. 43 (12), 2505–2527.CrossRefGoogle Scholar
Berloff, P. & Kamenkovich, I. 2013 b On spectral analysis of mesoscale eddies. Part II: nonlinear analysis. J. Phys. Oceanogr. 43 (12), 2528–2544.CrossRefGoogle Scholar
Berloff, P., Kamenkovich, I. & Pedlosky, J. 2009 a A mechanism of formation of multiple zonal jets in the oceans. J. Fluid Mech. 628, 395–425.CrossRefGoogle Scholar
Berloff, P., Kamenkovich, I. & Pedlosky, J. 2009 b A model of multiple zonal jets in the oceans: dynamical and kinematical analysis. J. Phys. Oceanogr. 39 (11), 2711–2734.CrossRefGoogle Scholar
Chen, C., Kamenkovich, I. & Berloff, P. 2016 Eddy trains and striations in quasigeostrophic simulations and the ocean. J. Phys. Oceanogr. 46 (9), 2807–2825.CrossRefGoogle Scholar
Connaughton, C.P., Nadiga, B.T., Nazarenko, S.V. & Quinn, B.E. 2010 Modulational instability of rossby and drift waves and generation of zonal jets. J. Fluid Mech. 654, 207–231.CrossRefGoogle Scholar
Danilov, S. & Gurarie, D. 2004 Scaling, spectra and zonal jets in beta-plane turbulence. Phys. Fluids 16 (7), 2592–2603.CrossRefGoogle Scholar
Danilov, S.D. & Gurarie, D. 2000 Quasi-two-dimensional turbulence. Usp. Fiz. Nauk. 170, 921–968.CrossRefGoogle Scholar
Farrell, B.F. & Ioannou, P.J. 2007 Structure and spacing of jets in barotropic turbulence. J. Atmos. Sci. 64 (10), 3652–3665.CrossRefGoogle Scholar
Farrell, B.F. & Ioannou, P.J. 2008 Formation of jets by baroclinic turbulence. J. Atmos. Sci. 65 (11), 3353–3375.CrossRefGoogle Scholar
Galperin, B., Nakano, H., Huang, H.-P. & Sukoriansky, S. 2004 The ubiquitous zonal jets in the atmospheres of giant planets and earth's oceans. Geophys. Res. Lett. 31, L13303.CrossRefGoogle Scholar
Galperin, B., Sukoriansky, S. & Dikovskaya, N. 2010 Geophysical flows with anisotropic turbulence and dispersive waves: flows with a $\beta$-effect. Ocean Dyn. 60 (2), 427–441.CrossRefGoogle Scholar
Huang, H.-P., Galperin, B. & Sukoriansky, S. 2001 Anisotropic spectra in two-dimensional turbulence on the surface of a rotating sphere. Phys. Fluids 13 (1), 225–240.CrossRefGoogle Scholar
Huang, H.-P., Kaplan, A., Curchitser, E.N. & Maximenko, N.A. 2007 The degree of anisotropy for mid-ocean currents from satellite observations and an eddy-permitting model simulation. J. Geophys. Res.: Oceans 112, C09005.Google Scholar
Kamenkovich, I., Berloff, P. & Pedlosky, J. 2009 Role of eddy forcing in the dynamics of multiple zonal jets in a model of the north atlantic. J. Phys. Oceanogr. 39 (6), 1361–1379.CrossRefGoogle Scholar
Kamenkovich, I.V., Rypina, I.I. & Berloff, P.S. 2015 Properties and origins of the anisotropic eddy-induced transport in the north atlantic. J. Phys. Oceanogr. 45 (3), 778–791.CrossRefGoogle Scholar
Khatri, H. & Berloff, P. 2018 a A mechanism for jet drift over topography. J. Fluid Mech. 845, 392–416.CrossRefGoogle Scholar
Khatri, H. & Berloff, P. 2018 b Role of eddies in the maintenance of multiple jets embedded in eastward and westward baroclinic shears. Fluids 3 (4), 91.CrossRefGoogle Scholar
Khatri, H. & Berloff, P. 2019 Tilted drifting jets over a zonally sloped topography: effects of vanishing eddy viscosity. J. Fluid Mech. 876, 939–961.CrossRefGoogle Scholar
Maltrud, M.E. & Vallis, G.K. 1991 Energy spectra and coherent structures in forced two-dimensional and beta-plane turbulence. J. Fluid Mech. 228, 321–342.Google Scholar
Marston, J.B., Conover, E. & Schneider, T. 2008 Statistics of an unstable barotropic jet from a cumulant expansion. J. Atmos. Sci. 65 (6), 1955–1966.CrossRefGoogle Scholar
Maximenko, N.A., Bang, B. & Sasaki, H. 2005 Observational evidence of alternating zonal jets in the world ocean. Geophys. Res. Lett. 32, L12607.CrossRefGoogle Scholar
Melnichenko, O.V., Maximenko, N.A., Schneider, N. & Sasaki, H. 2010 Quasi-stationary striations in basin-scale oceanic circulation: vorticity balance from observations and eddy-resolving model. Ocean Dyn. 60 (3), 653–666.CrossRefGoogle Scholar
O'Gorman, P.A. & Schneider, T. 2007 Recovery of atmospheric flow statistics in a general circulation model without nonlinear eddy-eddy interactions. Geophys. Res. Lett. 34 (22).CrossRefGoogle Scholar
Panetta, R.L. 1993 Zonal jets in wide baroclinically unstable regions: persistence and scale selection. J. Atmos. Sci. 50 (14), 2073–2106.2.0.CO;2>CrossRefGoogle Scholar
Pedlosky, J. 2013 Geophysical Fluid Dynamics. Springer Science & Business Media.Google Scholar
Rhines, P.B. 1975 Waves and turbulence on a beta-plane. J. Fluid Mech. 69 (3), 417–443.CrossRefGoogle Scholar
Rhines, P.B. 1994 Jets. Chaos 4 (2), 313–339.CrossRefGoogle ScholarPubMed
Richards, K.J., Maximenko, N.A., Bryan, F.O. & Sasaki, H. 2006 Zonal jets in the pacific ocean. Geophys. Res. Lett. 33 (3).CrossRefGoogle Scholar
Rudko, M.V., Kamenkovich, I.V., Iskadarani, M. & Mariano, A.J. 2018 Zonally elongated transient flows: phenomenology and sensitivity analysis. J. Geophys. Res.: Oceans 123 (6), 3982–4002.CrossRefGoogle Scholar
Rypina, I.I., Kamenkovich, I., Berloff, P. & Pratt, L.J. 2012 Eddy-induced particle dispersion in the near-surface north atlantic. J. Phys. Oceanogr. 42 (12), 2206–2228.CrossRefGoogle Scholar
Schlax, M.G. & Chelton, D.B. 2008 The influence of mesoscale eddies on the detection of quasi-zonal jets in the ocean. Geophys. Res. Lett. 35, L24602.CrossRefGoogle Scholar
Scott, R.B. & Arbic, B.K. 2007 Spectral energy fluxes in geostrophic turbulence: implications for ocean energetics. J. Phys. Oceanogr. 37 (3), 673–688.CrossRefGoogle Scholar
Scott, R.B., Arbic, B.K., Holland, C.L., Sen, A. & Qiu, B. 2008 Zonal versus meridional velocity variance in satellite observations and realistic and idealized ocean circulation models. Ocean Model. 23 (3–4), 102–112.CrossRefGoogle Scholar
Scott, R.B. & Wang, F. 2005 Direct evidence of an oceanic inverse kinetic energy cascade from satellite altimetry. J. Phys. Oceanogr. 35 (9), 1650–1666.CrossRefGoogle Scholar
Srinivasan, K. & Young, W.R. 2012 Zonostrophic instability. J. Atmos. Sci. 69 (5), 1633–1656.CrossRefGoogle Scholar
Stewart, K.D., Spence, P., Waterman, S., Le Sommer, J., Molines, J.-M., Lilly, J.M. & England, M.H. 2015 Anisotropy of eddy variability in the global ocean. Ocean Model. 95, 53–65.CrossRefGoogle Scholar
Sukoriansky, S., Dikovskaya, N. & Galperin, B. 2007 On the arrest of inverse energy cascade and the rhines scale. J. Atmos. Sci. 64 (9), 3312–3327.CrossRefGoogle Scholar
Sukoriansky, S., Dikovskaya, N. & Galperin, B. 2008 Nonlinear waves in zonostrophic turbulence. Phys. Rev. Lett. 101 (17), 178501.CrossRefGoogle ScholarPubMed
Sukoriansky, S., Galperin, B. & Chekhlov, A. 1999 Large scale drag representation in simulations of two-dimensional turbulence. Phys. Fluids 11 (10), 3043–3053.CrossRefGoogle Scholar
Vallis, G.K. & Maltrud, M.E. 1993 Generation of mean flows and jets on a beta plane and over topography. J. Phys. Oceanogr. 23 (7), 1346–1362.2.0.CO;2>CrossRefGoogle Scholar
Wang, J., Spall, M.A., Flierl, G.R. & Malanotte-Rizzoli, P. 2012 A new mechanism for the generation of quasi-zonal jets in the ocean. Geophys. Res. Lett. 39, L10601.CrossRefGoogle Scholar