Hostname: page-component-78c5997874-s2hrs Total loading time: 0 Render date: 2024-11-10T17:00:57.413Z Has data issue: false hasContentIssue false
  • English
  • Français

Effects of pore scale and conjugate heat transfer on thermal convection in porous media

Published online by Cambridge University Press:  29 June 2022

David Korba Open the ORCID record for David Korba [Opens in a new window]  and
Like Li Open the ORCID record for Like Li [Opens in a new window]
David Korba
Affiliation:
Department of Mechanical Engineering, Mississippi State University, Mississippi State, MS 39762, USA Center for Advanced Vehicular Systems, Mississippi State University, Mississippi State, MS 39762, USA
Like Li*
Affiliation:
Department of Mechanical Engineering, Mississippi State University, Mississippi State, MS 39762, USA Center for Advanced Vehicular Systems, Mississippi State University, Mississippi State, MS 39762, USA
*
†Email address for correspondence: likeli@me.msstate.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

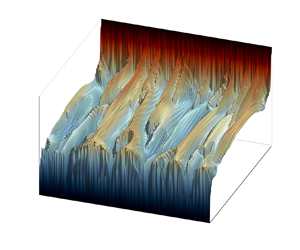
The study of thermal convection in porous media is of both fundamental and practical interest. Typically, numerical studies have relied on the volume-averaged Darcy–Oberbeck–Boussinesq (DOB) equations, where convection dynamics are assumed to be controlled solely by the Rayleigh number (Ra). Nusselt numbers (Nu) from these models predict Nu–Ra scaling exponents of 0.9–0.95. However, experiments and direct numerical simulations (DNS) have suggested scaling exponents as low as 0.319. Recent findings for solutal convection between DNS and DOB models have demonstrated that the ‘pore-scale parameters’ not captured by the DOB equations greatly influence convection. Thermal convection also has the additional complication of different thermal transport properties (e.g. solid-to-fluid thermal conductivity ratio ks/kf and heat capacity ratio σ) in different phases. Thus, in this work we compare results for thermal convection from the DNS and DOB equations. On the effects of pore size, DNS results show that Nu increases as pore size decreases. Mega-plumes are also found to be more frequent and smaller for reduced pore sizes. On the effects of conjugate heat transfer, two groups of cases (Group 1 with varying ks/kf at σ = 1 and Group 2 with varying σ at ks/kf = 1) are examined to compare the Nu–Ra relations at different porosity (ϕ) and ks/kf and σ values. Furthermore, we report that the boundary layer thickness is determined by the pore size in DNS results, while by both the Rayleigh number and the effective heat capacity ratio, $\bar{\phi } = \phi + (1 - \phi )\sigma$, in the DOB model.

JFM classification

Convection: Convection in porous media Convection: Plumes/thermals Convection: Buoyant boundary layers
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 944 , 10 August 2022 , A28
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81 (2), 503–537.CrossRefGoogle Scholar
Aidun, C.K. & Clausen, J.R. 2010 Lattice-Boltzmann method for complex flows. Annu. Rev. Fluid Mech. 42, 439–472.CrossRefGoogle Scholar
Benzi, R., Succi, S. & Vergassola, M. 1992 The lattice Boltzmann equation: theory and applications. Phys. Rep. 222 (3), 145–197.CrossRefGoogle Scholar
Chen, S. & Doolen, G.D. 1998 Lattice Boltzmann method. Annu. Rev. Fluid Mech. 30, 329–364.CrossRefGoogle Scholar
Chillà, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh–Bénard convection. Eur. Phys. J. E 35 (7), 58.CrossRefGoogle ScholarPubMed
Cinar, Y., Riaz, A. & Tchelepi, H.A. 2009 Experimental study of CO2 injection into saline formations. SPE J. 14 (4), 588–594.CrossRefGoogle Scholar
Davidson, J.H., Kulacki, F.A. & Savela, D. 2009 Natural convection in water-saturated reticulated vitreous carbon foam. Intl J. Heat Mass Transfer 52, 4479–4483.CrossRefGoogle Scholar
De Paoli, M., Zonta, F. & Soldati, A. 2016 Influence of anisotropic permeability on convection in porous media: implications for geological Co2 sequestration. Phys. Fluids 28 (5), 056601.CrossRefGoogle Scholar
Gasow, S., Kuznetsov, A.V., Avila, M. & Jin, Y. 2021 A macroscopic two-length-scale model for natural convection in porous media driven by a species-concentration gradient. J. Fluid Mech. 926, A8.CrossRefGoogle Scholar
Gasow, S., Lin, Z., Zhang, H.C., Kuznetsov, A.V., Avila, M. & Jin, Y. 2020 Effects of pore scale on the macroscopic properties of natural convection in porous media. J. Fluid Mech. 891, A25.CrossRefGoogle Scholar
Gasparini, P. & Mantovani, M.S.M. 1984 Heat Transfer Geothermal Areas 14, 9–39.Google Scholar
Hassanzadeh, H., Pooladi-Darvish, M. & Keith, D. 2012 Scaling behavior of convective mixing, with application to geological storage of CO2. AIChE J. 53 (5), 1121–1131.CrossRefGoogle Scholar
He, X., Funfschilling, D., Bodenschatz, E. & Ahlers, G. 2012 a Heat transport by turbulent Rayleigh–Bénard convection for $Pr \simeq 0.8$ and $4\times10\; 11 \lesssim Ra \lesssim 2\times 10\;14$: ultimate-state transition for aspect ratio $\varGamma$ = 1.00. New J. Phys. 14, 063030.CrossRefGoogle Scholar
He, X., Funfschilling, D., Nobach, H., Bodenschatz, E. & Ahlers, G. 2012 b Transition to the ultimate state of turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 108 (2), 024502.CrossRefGoogle Scholar
He, X. & Luo, L.-S. 1997 Lattice Boltzmann model for the incompressible Navier–Stokes equation. J. Stat. Phys. 88 (3/4), 927–944.CrossRefGoogle Scholar
Hewitt, D.R. 2020 Vigorous convection in porous media. Proc. R. Soc. A 476, 20200111.CrossRefGoogle ScholarPubMed
Hewitt, D.R., Neufeld, J.A. & Lister, J.R. 2012 Ultimate regime of high Rayleigh number convection in a porous medium. Phys. Rev. Lett. 108, 224503.CrossRefGoogle Scholar
Hewitt, D.R., Neufeld, J.A. & Lister, J.R. 2013 Convective shutdown in a porous medium at high Rayleigh number. J. Fluid Mech. 719, 551–586.CrossRefGoogle Scholar
Hewitt, D.R., Neufeld, J.A. & Lister, J.R. 2014 High Rayleigh number convection in a three-dimensional porous medium. J. Fluid Mech. 748, 879–895.CrossRefGoogle Scholar
Huppert, H.E. & Neufeld, J.A. 2014 The fluid mechanics of carbon dioxide sequestration. Annu. Rev. Fluid Mech. 46, 255–272.CrossRefGoogle Scholar
Iyer, K.P., Scheel, J.D., Schumacher, J. & Sreenivasan, K.R. 2020 Classical 1/3 scaling of convection holds up to Ra = 1015. Proc. Natl Acad. Sci. USA 117 (14), 7594–7598.CrossRefGoogle ScholarPubMed
Jonsson, T. & Catton, I. 1985 Prandtl number dependence of natural convection in porous medium. Am. Soc. Mech. Engrs, Heat Transfer Div. (Publ.) HTD 46 (May 1987), 21–29.Google Scholar
Karani, H. & Huber, C. 2017 Role of thermal disequilibrium on natural convection in porous media: insights from pore-scale study. Phys. Rev. E 95, 033123.CrossRefGoogle ScholarPubMed
Karani, H., Rashtbehesht, M., Huber, C. & Magin, R.L. 2017 Onset of fractional-order thermal convection in porous media. Phys. Rev. E 96, 063105.CrossRefGoogle ScholarPubMed
Keene, D.J. & Goldstein, R.J. 2015 Thermal convection in porous media at high Rayleigh numbers. Am. Soc. Mech. Engrs, Heat Transfer Div. (Publ.) HTD 137 (3), 034503.Google Scholar
Kladias, N. & Prasad, V. 1991 Experimental verification of Darcy-Brinkman-Forchheimer flow model for natural convection in porous media. J. Thermophys. Heat Transfer 5 (4), 560–576.CrossRefGoogle Scholar
Korba, D., Wang, N. & Li, L. 2020 Accuracy of interface schemes for conjugate heat and mass transfer in the lattice Boltzmann method. Intl J. Heat Mass Transfer 156, 119694.CrossRefGoogle Scholar
Kränzien, P.U. & Jin, Y. 2018 Natural convection in a two-dimensional cell filled with a porous medium: a direct numerical simulation study. Heat Transfer Engng 40, 487–496.CrossRefGoogle Scholar
Lallemand, P. & Luo, L.-S. 2000 Theory of the lattice Boltzmann method: dispersion, dissipation, isotropy. Galilean invariance, and stability. Phys. Rev. E 61 (6), 6546–6562.CrossRefGoogle ScholarPubMed
de Lemos, M.J.S. 2012 Turbulence in Porous Media: Modeling and Applications, 2nd edn. Elsevier.Google Scholar
Le Reun, T. & Hewitt, D.R. 2021 High-Rayleigh-number convection in porous-fluid layers. J. Fluid Mech. 920, A35.CrossRefGoogle Scholar
Li, L., Chen, C., Mei, R. & Klausner, J.F. 2014 Conjugate heat and mass transfer in the lattice Boltzmann equation method. Phys. Rev. E – Stat. Nonlinear Soft Matt. Phys. 89 (4), 043308.CrossRefGoogle ScholarPubMed
Li, X., He, J., Tian, Y., Hao, P. & Huang, S. 2021 Effects of Prandtl number in quasi- two-dimensional Rayleigh–Bénard convection. J. Fluid Mech. 915, A60.CrossRefGoogle Scholar
Li, L., Mei, R. & Klausner, J.F. 2013 Boundary conditions for thermal lattice Boltzmann equation method. J. Comput. Phys. 237, 366–395.CrossRefGoogle Scholar
Li, L., Mei, R. & Klausner, J.F. 2017 Lattice Boltzmann models for the convection-diffusion equation: D2Q5 vs D2Q9. Intl J. Heat Mass Transfer 108, 41–62.CrossRefGoogle Scholar
Liang, Y., Wen, B., Hesse, M.A. & DiCarlo, D. 2018 Effect of dispersion on solutal convection in porous media. Geophys. Res. Lett. 45 (18), 9690–9698.CrossRefGoogle Scholar
Lister, C.R.B. 1990 An explanation for the multivalued heat transport found experimentally for convection in a porous medium. J. Fluid Mech. 214 (6), 287–320.Google Scholar
Liu, S., Jiang, L., Chong, K.L. & Zhu, X. 2020 From Rayleigh–Bénard convection to porous-media convection: how porosity affects heat transfer and flow structure. J. Fluid Mech. 895, A18.CrossRefGoogle Scholar
Lohse, D. & Xia, K.Q. 2010 Small-scale properties of turbulent Rayleigh-Benard convection. Annu. Rev. Fluid Mech. 42, 335–364.CrossRefGoogle Scholar
Luo, L.-S. 1993 Lattice-gas automata and lattice Boltzmann equations for two-dimensional hydrodynamics, 274. PhD Thesis, Georgia Institute of Technology.Google Scholar
Miansari, M., Gorji, M., Ganji, D.D. & Hooman, K. 2015 Comparison between continuum and porous continuum models in studying natural convection in porous cavity with random distribution of solid obstacles. Intl J. Numer. Meth. Heat Fluid Flow 25 (3), 484–503.CrossRefGoogle Scholar
Nield, D.A. & Bejan, A. 2017 Convection in Porous Media, 5th edn. Springer.CrossRefGoogle Scholar
Niemela, J.J., Skrbek, L., Sreenivasan, K.S. & Donnelly, R.J. 2000 Turbulent convection in very high Rayleigh numbers. Nature 404 (April), 837–840.CrossRefGoogle ScholarPubMed
Otero, J., Dontcheva, L.A., Johnston, H., Worthing, R.A., Kurganov, A., Petrova, G. & Doering, C.R. 2004 High-Rayleigh-number convection in a fluid-saturated porous layer. J. Fluid Mech. 500, 263–281.CrossRefGoogle Scholar
van der Poel, E.P., Stevens, R.J.A.M. & Lohse, D. 2013 Comparison between two- and three-dimensional Rayleigh–Bénard convection. J. Fluid Mech. 736, 177–194.CrossRefGoogle Scholar
Qiang, W., Xie, M., Liu, Z., Wang, Y., Cao, H. & Zhou, H. 2021 Asymptotic behaviour of heat transfer in two-dimensional turbulent convection with high-porosity fluid-saturated media. J. Fluid Mech. 923, A30.CrossRefGoogle Scholar
Ratouis, T.M.P. & Zarrouk, S.J. 2016 Factors controlling large-scale hydrodynamic convection in the Taupo Volcanic Zone (TVZ), New Zealand. Geothermics 59, 236–251.Google Scholar
Roche, P., Castaing, B., Chabaud, B. & Hebral, B. 2001 Observation of the 1/2 power law in Rayleigh–Bénard convection. Phys. Rev. E 63 (4 Pt 2), 045303.Google Scholar
Shishkina, O., Jam Stevens, R., Grossmann, S. & Lohse, D. 2010 Boundary layer structure in turbulent thermal convection and its consequences for the required numerical resolution. New J. Phys. 12, 075022.CrossRefGoogle Scholar
Siggia, E. 1994 High Rayleigh number convection. Annu. Rev. Fluid Mech. 26, 137–168.CrossRefGoogle Scholar
Toppaladoddi, S., Succi, S. & Wettlaufer, J.S. 2017 Roughness as a route to the ultimate regime of thermal convection. Phys. Rev. Lett. 118 (7), 074503.CrossRefGoogle Scholar
Tummers, M.J. & Steunebrink, M. 2019 Effect of surface roughness on heat transfer in Rayleigh-Bénard convection. Intl J. Heat Mass Transfer 139, 1056–1064.CrossRefGoogle Scholar
Wang, M. & Pan, N. 2008 Predictions of effective physical properties of complex multiphase materials. Mater. Sci. Engng R: Rep. 63 (1), 1–30.CrossRefGoogle Scholar
Wen, B., Chang, K.W. & Hesse, M.A. 2018 Rayleigh-Darcy convection with hydrodynamic dispersion. Phys. Rev. Fluids 3 (12), 123801.CrossRefGoogle Scholar
Wen, B., Corson, L.T. & Chini, G.P. 2015 Structure and stability of steady porous medium convection at large Rayleigh number. J. Fluid Mech. 772, 197–224.CrossRefGoogle Scholar
Wilson, A. & Ruppel, C. 2007 Salt tectonics and shallow subseafloor fluid convection: models of coupled fluid-heat-salt transport. Geofluids 7 (4), 377–386.CrossRefGoogle Scholar
Yoshida, H. & Nagaoka, M. 2010 Multiple-relaxation-time lattice Boltzmann model for the convection and anisotropic diffusion equation. J. Comput. Phys. 229 (20), 7774–7795.CrossRefGoogle Scholar
Yu, D., Mei, R., Luo, L. & Shyy, W. 2003 Viscous flow computations with the method of lattice Boltzmann equation. Prog. Aerosp. Sci. 39, 329–367.Google Scholar
Zhang, Y., Sun, C., Bao, Y. & Zhou, Q. 2018 How surface roughness reduces heat transport for small roughness heights in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 836, R2.CrossRefGoogle Scholar
Zhang, L., Yang, S., Zeng, Z., Chen, J., Wang, L. & Chew, J.W. 2017 A comparative study of the axisymmetric lattice Boltzmann models under the incompressible limit. Comput. Math. Appl. 74 (4), 817–841.CrossRefGoogle Scholar
Zhu, X., Mathai, V., Stevens, R.J.A.M., Verzicco, R. & Lohse, D. 2018 Transition to the ultimate regime in two-dimensional Rayleigh-Bénard convection. Phys. Rev. Lett. 120 (14), 144502.CrossRefGoogle Scholar
Zhu, X., Stevens, R.J.A.M., Verzicco, R. & Lohse, D. 2017 Roughness-facilitated local 1/2 scaling does not imply the onset of the ultimate regime of thermal convection. Phys. Rev. Lett. 119 (15), 154501.CrossRefGoogle Scholar

Korba and Li supplementary movie 1

Temperature evolution for DOB simulation at $Ra = 5000$, $k_s/k_f = 1$ and $\sigma = 1$

Download Korba and Li supplementary movie 1(Video)
Video 7.9 MB

Korba and Li supplementary movie 2

Temperature evolution for DOB simulation at $Ra = 10 \: 000$, $k_s/k_f = 1$ and $\sigma = 1$

Download Korba and Li supplementary movie 2(Video)
Video 8.6 MB

Korba and Li supplementary movie 3

Temperature evolution for DOB simulation at $Ra = 20 \: 000$, $k_s/k_f = 1$ and $\sigma = 1$

Download Korba and Li supplementary movie 3(Video)
Video 9.4 MB

Korba and Li supplementary movie 4

Temperature evolution for DNS at $Ra = 5000$, $k_s/k_f = 1$, $\sigma = 1$, $H/m = 25$ and $\phi = 0.56$

Download Korba and Li supplementary movie 4(Video)
Video 8.9 MB

Korba and Li supplementary movie 5

Temperature evolution for DNS at $Ra = 10 \: 000$, $k_s/k_f = 1$, $\sigma = 1$, $H/m = 25$ and $\phi = 0.56$

Download Korba and Li supplementary movie 5(Video)
Video 10 MB

Korba and Li supplementary movie 6

Temperature evolution for DNS at $Ra = 20 \: 000$, $k_s/k_f = 1$, $\sigma = 1$, $H/m = 25$ and $\phi = 0.56$

Download Korba and Li supplementary movie 6(Video)
Video 9.2 MB