Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Khosh Aghdam, Sohrab
and
Ricco, Pierre
2016.
Laminar and turbulent flows over hydrophobic surfaces with shear-dependent slip length.
Physics of Fluids,
Vol. 28,
Issue. 3,
Patlazhan, Stanislav
and
Vagner, Sergei
2017.
Apparent slip of shear thinning fluid in a microchannel with a superhydrophobic wall.
Physical Review E,
Vol. 96,
Issue. 1,
Watanabe, Sho
Mamori, Hiroya
and
Fukagata, Koji
2017.
Drag-reducing performance of obliquely aligned superhydrophobic surface in turbulent channel flow.
Fluid Dynamics Research,
Vol. 49,
Issue. 2,
p.
025501.
Im, Hyung Jae
and
Lee, Jae Hwa
2017.
Comparison of superhydrophobic drag reduction between turbulent pipe and channel flows.
Physics of Fluids,
Vol. 29,
Issue. 9,
2017.
Wall Turbulence Control.
p.
167.
Abu Rowin, W.
Hou, J.
and
Ghaemi, S.
2017.
Inner and outer layer turbulence over a superhydrophobic surface with low roughness level at low Reynolds number.
Physics of Fluids,
Vol. 29,
Issue. 9,
Rastegari, Amirreza
and
Akhavan, Rayhaneh
2018.
The common mechanism of turbulent skin-friction drag reduction with superhydrophobic longitudinal microgrooves and riblets.
Journal of Fluid Mechanics,
Vol. 838,
Issue. ,
p.
68.
Lee, Jungjin
Kim, Hyunseok
and
Park, Hyungmin
2018.
Effects of superhydrophobic surfaces on the flow around an NACA0012 hydrofoil at low Reynolds numbers.
Experiments in Fluids,
Vol. 59,
Issue. 7,
Seo, Jongmin
García-Mayoral, Ricardo
and
Mani, Ali
2018.
Turbulent flows over superhydrophobic surfaces: flow-induced capillary waves, and robustness of air–water interfaces.
Journal of Fluid Mechanics,
Vol. 835,
Issue. ,
p.
45.
Castagna, Marco
Mazellier, Nicolas
and
Kourta, Azeddine
2018.
Wake of super-hydrophobic falling spheres: influence of the air layer deformation.
Journal of Fluid Mechanics,
Vol. 850,
Issue. ,
p.
646.
Qi, Hongyuan
Liang, Aiguo
Jiang, Huayi
Chong, Xinmin
and
Wang, Yulong
2018.
Effect of Pipe Surface Wettability on Flow Slip Property.
Industrial & Engineering Chemistry Research,
Vol. 57,
Issue. 37,
p.
12543.
Seo, Jongmin
and
Mani, Ali
2018.
Effect of texture randomization on the slip and interfacial robustness in turbulent flows over superhydrophobic surfaces.
Physical Review Fluids,
Vol. 3,
Issue. 4,
Khashi’ie, Najiyah Safwa
Md Arifin, Norihan
Nazar, Roslinda
Hafidzuddin, Ezad Hafidz
Wahi, Nadihah
and
Pop, Ioan
2019.
A Stability Analysis for Magnetohydrodynamics Stagnation Point Flow with Zero Nanoparticles Flux Condition and Anisotropic Slip.
Energies,
Vol. 12,
Issue. 7,
p.
1268.
Chang, Jaehee
Jung, Taeyong
Choi, Haecheon
and
Kim, John
2019.
Predictions of the effective slip length and drag reduction with a lubricated micro-groove surface in a turbulent channel flow.
Journal of Fluid Mechanics,
Vol. 874,
Issue. ,
p.
797.
Choi, Hongseok
Lee, Jungjin
and
Park, Hyungmin
2019.
Wake structures behind a rotor with superhydrophobic-coated blades at low Reynolds number.
Physics of Fluids,
Vol. 31,
Issue. 1,
Alamé, Karim
and
Mahesh, Krishnan
2019.
Wall-bounded flow over a realistically rough superhydrophobic surface.
Journal of Fluid Mechanics,
Vol. 873,
Issue. ,
p.
977.
Shahsavari, Ali
Nejat, Amir
and
Chini, Seyed Farshid
2019.
Advances in Contact Angle, Wettability and Adhesion.
p.
239.
Arenas, Isnardo
García, Edgardo
Fu, Matthew K.
Orlandi, Paolo
Hultmark, Marcus
and
Leonardi, Stefano
2019.
Comparison between super-hydrophobic, liquid infused and rough surfaces: a direct numerical simulation study.
Journal of Fluid Mechanics,
Vol. 869,
Issue. ,
p.
500.
Lee, Sang Joon
Kim, Hae Nyeok
Choi, Woorak
Yoon, Gun Young
and
Seo, Eunseok
2019.
A nature-inspired lubricant-infused surface for sustainable drag reduction.
Soft Matter,
Vol. 15,
Issue. 42,
p.
8459.
Wu, Xiaohua
Cruickshank, Mike
and
Ghaemi, Sina
2020.
Negative skin friction during transition in a zero-pressure-gradient flat-plate boundary layer and in pipe flows with slip and no-slip boundary conditions.
Journal of Fluid Mechanics,
Vol. 887,
Issue. ,
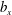 $b_{x}$) is larger than the spanwise one (
$b_{x}$) is larger than the spanwise one (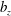 $b_{z}$). From the joint probability density function of the slip velocities and velocity gradients at the interface, we confirm the anisotropy of the slip lengths and obtain their relative magnitude (
$b_{z}$). From the joint probability density function of the slip velocities and velocity gradients at the interface, we confirm the anisotropy of the slip lengths and obtain their relative magnitude (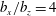 $b_{x}/b_{z}=4$) for the present idealized superhydrophobic surface. It is also shown that the Navier slip model is valid only in the mean sense, and it is generally not applicable to fluctuating quantities.
$b_{x}/b_{z}=4$) for the present idealized superhydrophobic surface. It is also shown that the Navier slip model is valid only in the mean sense, and it is generally not applicable to fluctuating quantities.


