Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Kim, Hyeonseong
Kang, Sohyeun
and
Kim, Daegyoum
2017.
Dynamics of a flag behind a bluff body.
Journal of Fluids and Structures,
Vol. 71,
Issue. ,
p.
1.
Orrego, Santiago
Shoele, Kourosh
Ruas, Andre
Doran, Kyle
Caggiano, Brett
Mittal, Rajat
and
Kang, Sung Hoon
2017.
Harvesting ambient wind energy with an inverted piezoelectric flag.
Applied Energy,
Vol. 194,
Issue. ,
p.
212.
Kim, Junyoung
Song, Minho
and
Kim, Daegyoum
2018.
Large-amplitude flow-induced vibration of cylindrical pendulums.
Journal of Fluids and Structures,
Vol. 79,
Issue. ,
p.
39.
Goza, Andres
Colonius, Tim
and
Sader, John E.
2018.
Global modes and nonlinear analysis of inverted-flag flapping.
Journal of Fluid Mechanics,
Vol. 857,
Issue. ,
p.
312.
Huang, Haibo
Wei, Heng
and
Lu, Xi-Yun
2018.
Coupling performance of tandem flexible inverted flags in a uniform flow.
Journal of Fluid Mechanics,
Vol. 837,
Issue. ,
p.
461.
Ryu, Jaeha
Park, Sung Goon
and
Sung, Hyung Jin
2018.
Flapping dynamics of inverted flags in a side-by-side arrangement.
International Journal of Heat and Fluid Flow,
Vol. 70,
Issue. ,
p.
131.
Abdelbaki, A.R.
Païdoussis, M.P.
and
Misra, A.K.
2018.
A nonlinear model for a free-clamped cylinder subjected to confined axial flow.
Journal of Fluids and Structures,
Vol. 80,
Issue. ,
p.
390.
Elahi, Hassan
Eugeni, Marco
and
Gaudenzi, Paolo
2018.
A Review on Mechanisms for Piezoelectric-Based Energy Harvesters.
Energies,
Vol. 11,
Issue. 7,
p.
1850.
Dou, Zhongwang
Rips, Aaron
Welsh, Nathaniel
Seo, Jung-Hee
and
Mittal, Rajat
2018.
Flow-Induced Flutter of Hanging Banners: Experiments and Validated Computational Models.
Serry, Mohamed
and
Tuffaha, Amjad
2018.
Static stability analysis of a thin plate with a fixed trailing edge in axial subsonic flow: Possio integral equation approach.
Applied Mathematical Modelling,
Vol. 63,
Issue. ,
p.
644.
Huertas-Cerdeira, Cecilia
Fan, Boyu
and
Gharib, Morteza
2018.
Coupled motion of two side-by-side inverted flags.
Journal of Fluids and Structures,
Vol. 76,
Issue. ,
p.
527.
Lan, Chunbo
Tang, Lihua
Hu, Guobiao
and
Qin, Weiyang
2019.
Dynamics and performance of a two degree-of-freedom galloping-based piezoelectric energy harvester.
Smart Materials and Structures,
Vol. 28,
Issue. 4,
p.
045018.
Huber, Ryan A.
Campbell, Matthew
Doughramaji, Nicole
and
Derby, Melanie M.
2019.
Vibration-Enhanced Droplet Motion Modes: Simulations of Rocking, Ratcheting, Ratcheting With Breakup, and Ejection.
Journal of Fluids Engineering,
Vol. 141,
Issue. 7,
Olivieri, S.
Boragno, C.
Verzicco, R.
and
Mazzino, A.
2019.
Constructive interference in a network of elastically-bounded flapping plates.
Journal of Fluids and Structures,
Vol. 90,
Issue. ,
p.
334.
Ojo, Oluwafemi
Tan, David
Wang, Yu-Cheng
Shoele, Kourosh
Erturk, Alper
and
Erturk, Alper
2019.
Aspect ratio effects in wind energy harvesting using piezoelectric inverted flags.
p.
23.
Chawdhury, Samir
and
Morgenthal, Guido
2019.
A partitioned solver to simulate large-displacement fluid–structure interaction of thin plate systems for vibration energy harvesting.
Computers & Structures,
Vol. 224,
Issue. ,
p.
106110.
Yu, Yuelong
Liu, Yingzheng
and
Amandolese, Xavier
2019.
A Review on Fluid-Induced Flag Vibrations.
Applied Mechanics Reviews,
Vol. 71,
Issue. 1,
Jia, Kang
Fang, Le
and
Huang, Wei-Xi
2019.
Coupled states of dual side-by-side inverted flags in a uniform flow.
Journal of Fluids and Structures,
Vol. 91,
Issue. ,
p.
102768.
Huang, Wei-Xi
and
Tian, Fang-Bao
2019.
Recent trends and progress in the immersed boundary method.
Proceedings of the Institution of Mechanical Engineers, Part C: Journal of Mechanical Engineering Science,
Vol. 233,
Issue. 23-24,
p.
7617.
Silva-Leon, Jorge
Cioncolini, Andrea
Nabawy, Mostafa R.A.
Revell, Alistair
and
Kennaugh, Andrew
2019.
Simultaneous wind and solar energy harvesting with inverted flags.
Applied Energy,
Vol. 239,
Issue. ,
p.
846.
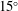 $15^{\circ }$. The state with large symmetric flutter is identified as being most promising for energy harvesting, and the effect of piezoelectric material parameters on the energy harvesting performance of this flutter state is examined in detail. The maximum energy efficiency of the flags is found to be approximately
$15^{\circ }$. The state with large symmetric flutter is identified as being most promising for energy harvesting, and the effect of piezoelectric material parameters on the energy harvesting performance of this flutter state is examined in detail. The maximum energy efficiency of the flags is found to be approximately  $7\,\%$, and the maximum occurs when there is a match between the time scales of flutter and the intrinsic time scale of the piezoelectric circuit. The simulations are used to examine a simple scaling law that could be used to predict the energy harvesting performance of such devices.
$7\,\%$, and the maximum occurs when there is a match between the time scales of flutter and the intrinsic time scale of the piezoelectric circuit. The simulations are used to examine a simple scaling law that could be used to predict the energy harvesting performance of such devices.