1. Introduction
Exact solutions of vortices are of fundamental importance in fluid dynamics. In particular, in geophysical fluid dynamics research, which includes the physical processes in the Earth's oceans and atmosphere, there is a large interest in understanding the persistence of large-scale, mesoscale and submesoscale vortices. An important step in the theoretical effort to find exact vortex solutions was the discovery, in two-dimensional flows and circular geometry, of the Lamb–Chaplygin dipole (Chaplygin Reference Chaplygin1903; Meleshko & van Heijst Reference Meleshko and van Heijst1994). The Lamb–Chaplygin vortex solution has relevant applications to ocean eddies (e.g. Flierl, Stern & Whitehead Reference Flierl, Stern and Whitehead1983; Gonzalez & Zavala Sansón Reference Gonzalez and Zavala Sansón2021). The essential property of the two-dimensional Lamb–Chaplygin streamfunction solution ![]() $\psi (\rho,\varphi )$ lies in the separation of the radial (
$\psi (\rho,\varphi )$ lies in the separation of the radial (![]() $\rho$) and angular (
$\rho$) and angular (![]() $\varphi$) dependence of the vertical vorticity
$\varphi$) dependence of the vertical vorticity ![]() $\zeta (\rho,\varphi )$ in cylindrical Bessel functions of the first kind,
$\zeta (\rho,\varphi )$ in cylindrical Bessel functions of the first kind, ![]() ${\rm J}_m(k\rho )$, and sinusoidal modes
${\rm J}_m(k\rho )$, and sinusoidal modes ![]() $\exp (-{\rm i}m\varphi )$, for azimuthal wavenumbers
$\exp (-{\rm i}m\varphi )$, for azimuthal wavenumbers ![]() $m=0$ and
$m=0$ and ![]() $m=1$, in such a way that
$m=1$, in such a way that ![]() $\psi$ satisfies the two-dimensional Helmholtz equation
$\psi$ satisfies the two-dimensional Helmholtz equation ![]() $\nabla ^2\psi = - k^2\psi$. The Lamb–Chaplygin vortex solution may be generalized to include an arbitrary number of Bessel-sinusoidal modes (Velasco Fuentes Reference Velasco Fuentes2000; Viúdez Reference Viúdez2019). In three-dimensional flows, a similar decomposition of the velocity field into spherical Bessel functions
$\nabla ^2\psi = - k^2\psi$. The Lamb–Chaplygin vortex solution may be generalized to include an arbitrary number of Bessel-sinusoidal modes (Velasco Fuentes Reference Velasco Fuentes2000; Viúdez Reference Viúdez2019). In three-dimensional flows, a similar decomposition of the velocity field into spherical Bessel functions ![]() ${\rm j}_m(kr)$ for the radial (
${\rm j}_m(kr)$ for the radial (![]() $r$) and angular (
$r$) and angular (![]() $\theta,\varphi$) and spherical harmonics
$\theta,\varphi$) and spherical harmonics ![]() $Y_{\ell }^{m}(\theta,\varphi )$, in the particular case
$Y_{\ell }^{m}(\theta,\varphi )$, in the particular case ![]() $\ell =1$ and
$\ell =1$ and ![]() $m=0$, lead to the discovery of the Hicks–Moffatt spherical vortex (Hicks Reference Hicks1899; Moffatt Reference Moffatt1969, Reference Moffatt2017), whose limit for vanishing radial wavenumber
$m=0$, lead to the discovery of the Hicks–Moffatt spherical vortex (Hicks Reference Hicks1899; Moffatt Reference Moffatt1969, Reference Moffatt2017), whose limit for vanishing radial wavenumber ![]() $k \rightarrow 0$ is Hill's spherical vortex (Hill Reference Hill1894). These steady solutions were generalized recently to multipolar spherical vortices with any degree
$k \rightarrow 0$ is Hill's spherical vortex (Hill Reference Hill1894). These steady solutions were generalized recently to multipolar spherical vortices with any degree ![]() $\ell$ and order
$\ell$ and order ![]() $m$ (Viúdez Reference Viúdez2022). These steady-state, or rigidly translating, vortex solutions are further generalized in this work. Here, we provide a family of time-dependent oscillating velocity fields
$m$ (Viúdez Reference Viúdez2022). These steady-state, or rigidly translating, vortex solutions are further generalized in this work. Here, we provide a family of time-dependent oscillating velocity fields ![]() $\boldsymbol {u}(\boldsymbol {x},t)$, with vorticity
$\boldsymbol {u}(\boldsymbol {x},t)$, with vorticity ![]() $\boldsymbol {\omega }\equiv \boldsymbol {\nabla }\times \boldsymbol {u}$, which is an exact solution, in spherical geometry, to the time-dependent nonlinear vorticity Euler equation
$\boldsymbol {\omega }\equiv \boldsymbol {\nabla }\times \boldsymbol {u}$, which is an exact solution, in spherical geometry, to the time-dependent nonlinear vorticity Euler equation
The velocity field ![]() $\boldsymbol {u}(\boldsymbol {x},t)$ of the new time-dependent spherical vortex family is described in § 2. First,
$\boldsymbol {u}(\boldsymbol {x},t)$ of the new time-dependent spherical vortex family is described in § 2. First, ![]() $\boldsymbol {u}(\boldsymbol {x},t)$ is defined in § 2.1 as the sum of a time and space oscillating velocity function
$\boldsymbol {u}(\boldsymbol {x},t)$ is defined in § 2.1 as the sum of a time and space oscillating velocity function ![]() $\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$ and a constant background rigid flow
$\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$ and a constant background rigid flow ![]() $\bar {\boldsymbol {u}}(\boldsymbol {x})$. The proof that
$\bar {\boldsymbol {u}}(\boldsymbol {x})$. The proof that ![]() $\boldsymbol {u}(\boldsymbol {x},t)$ satisfies (1.1) is given in § 2.2, and next the streamfunction
$\boldsymbol {u}(\boldsymbol {x},t)$ satisfies (1.1) is given in § 2.2, and next the streamfunction ![]() $\psi (\boldsymbol {x},t)$ of the total flow (§ 2.3), the different frequencies and phase speed of the motion (§ 2.4), the divergence of the Lamb vector (§ 2.5), and the acceleration potential (§ 2.6) are provided explicitly. Next, oscillating velocity solutions to the Navier–Stokes equation are given (§ 3) in terms of
$\psi (\boldsymbol {x},t)$ of the total flow (§ 2.3), the different frequencies and phase speed of the motion (§ 2.4), the divergence of the Lamb vector (§ 2.5), and the acceleration potential (§ 2.6) are provided explicitly. Next, oscillating velocity solutions to the Navier–Stokes equation are given (§ 3) in terms of ![]() $\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$ and
$\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$ and ![]() $\bar {\boldsymbol {u}}(\boldsymbol {x})$. The transformations of these solutions under a change of reference frame translating with constant axial velocity and rotating with constant angular speed are discussed in §§ 4 and 5, respectively. These solutions satisfy several well-known wave equations in physics, described in § 6, as well as the Maxwell equations for the propagation of electromagnetic waves in vacuum (§ 7). The velocity solutions
$\bar {\boldsymbol {u}}(\boldsymbol {x})$. The transformations of these solutions under a change of reference frame translating with constant axial velocity and rotating with constant angular speed are discussed in §§ 4 and 5, respectively. These solutions satisfy several well-known wave equations in physics, described in § 6, as well as the Maxwell equations for the propagation of electromagnetic waves in vacuum (§ 7). The velocity solutions ![]() $\boldsymbol {u}(\boldsymbol {x},t)$ may be considered velocity modes, and they satisfy a superposition condition explained in § 8. The stability of the zonal vortex solutions (where the order of the spherical harmonics vanishes,
$\boldsymbol {u}(\boldsymbol {x},t)$ may be considered velocity modes, and they satisfy a superposition condition explained in § 8. The stability of the zonal vortex solutions (where the order of the spherical harmonics vanishes, ![]() $m=0$) is addressed in § 9. Piecewise vortex solutions describing spatially bounded vorticity fields are considered in § 10, and finally, conclusions are summarized in § 11.
$m=0$) is addressed in § 9. Piecewise vortex solutions describing spatially bounded vorticity fields are considered in § 10, and finally, conclusions are summarized in § 11.
2. The time-dependent spherical vortex family
2.1. Definition of the time-dependent velocity solution
In order to define the time-dependent velocity solution ![]() $\boldsymbol {u}(\boldsymbol {x},t)$, it is convenient to introduce first, in spherical coordinates
$\boldsymbol {u}(\boldsymbol {x},t)$, it is convenient to introduce first, in spherical coordinates ![]() $(r,\theta,\varphi )$ and time
$(r,\theta,\varphi )$ and time ![]() $t$, the oscillating, in both space and time, function
$t$, the oscillating, in both space and time, function
 $$\begin{gather} \boldsymbol{\mathcal{U}}(r,\theta,\varphi,t)\equiv u_1 \left[\ell(\ell+1)\,\frac{{\rm j}_{\ell}(kr)}{kr}\,\boldsymbol{Y}_{\ell}^{m}(\theta,\varphi) +\left( (\ell+1)\,\frac{ {\rm j}_{\ell}(kr)}{kr} - {\rm j}_{\ell+1}(kr) \right) \boldsymbol{\varPsi}_{\ell}^{m}(\theta,\varphi) \right.\nonumber\\ \left.{}+ {\rm j}_{\ell}(kr)\,\boldsymbol{\varPhi}_{\ell}^{m}(\theta,\varphi)\vphantom{\frac{{\rm j}_{\ell}(kr)}{kr}}\right] {\rm e}^{-{\rm i} m \mathfrak{w}t} , \end{gather}$$
$$\begin{gather} \boldsymbol{\mathcal{U}}(r,\theta,\varphi,t)\equiv u_1 \left[\ell(\ell+1)\,\frac{{\rm j}_{\ell}(kr)}{kr}\,\boldsymbol{Y}_{\ell}^{m}(\theta,\varphi) +\left( (\ell+1)\,\frac{ {\rm j}_{\ell}(kr)}{kr} - {\rm j}_{\ell+1}(kr) \right) \boldsymbol{\varPsi}_{\ell}^{m}(\theta,\varphi) \right.\nonumber\\ \left.{}+ {\rm j}_{\ell}(kr)\,\boldsymbol{\varPhi}_{\ell}^{m}(\theta,\varphi)\vphantom{\frac{{\rm j}_{\ell}(kr)}{kr}}\right] {\rm e}^{-{\rm i} m \mathfrak{w}t} , \end{gather}$$
where ![]() ${\rm j}_{\ell }(\,{\cdot}\, )$ is the spherical Bessel function of the first kind and degree
${\rm j}_{\ell }(\,{\cdot}\, )$ is the spherical Bessel function of the first kind and degree ![]() $\ell$, and the vector set
$\ell$, and the vector set ![]() $\{ \boldsymbol {Y}_{\ell }^{m}(\theta,\varphi ), \boldsymbol {\varPsi }_{\ell }^{m}(\theta,\varphi ), \boldsymbol {\varPhi }_{\ell }^{m}(\theta,\varphi )\}$, with integers
$\{ \boldsymbol {Y}_{\ell }^{m}(\theta,\varphi ), \boldsymbol {\varPsi }_{\ell }^{m}(\theta,\varphi ), \boldsymbol {\varPhi }_{\ell }^{m}(\theta,\varphi )\}$, with integers ![]() $\ell \ge 0$ and
$\ell \ge 0$ and ![]() $m\in \{ -\ell,\ldots,\ell \}$, is the vector spherical harmonics basis (Barrera, Estevez & Giraldo Reference Barrera, Estevez and Giraldo1985). The remaining parameters are the radial wavenumber
$m\in \{ -\ell,\ldots,\ell \}$, is the vector spherical harmonics basis (Barrera, Estevez & Giraldo Reference Barrera, Estevez and Giraldo1985). The remaining parameters are the radial wavenumber ![]() $k$, the oscillation velocity amplitude
$k$, the oscillation velocity amplitude ![]() $u_1$, and the frequency, or angular speed,
$u_1$, and the frequency, or angular speed, ![]() $\mathfrak {w}$. To simplify the notation, it is only shown explicitly in (2.1) the function in terms of the spherical Bessel functions of the first kind (hence the subscript 1 in the velocity amplitude
$\mathfrak {w}$. To simplify the notation, it is only shown explicitly in (2.1) the function in terms of the spherical Bessel functions of the first kind (hence the subscript 1 in the velocity amplitude ![]() $u_1$). This function assumes implicitly the addition of a similar function but whose radial dependence is given in terms of the spherical Bessel functions of the second kind
$u_1$). This function assumes implicitly the addition of a similar function but whose radial dependence is given in terms of the spherical Bessel functions of the second kind ![]() ${\rm y}_{\ell }(\,{\cdot}\, )$ instead of
${\rm y}_{\ell }(\,{\cdot}\, )$ instead of ![]() ${\rm j}_{\ell }(\,{\cdot}\, )$, and with a new constant velocity amplitude, say
${\rm j}_{\ell }(\,{\cdot}\, )$, and with a new constant velocity amplitude, say ![]() $u_2$ instead of
$u_2$ instead of ![]() $u_1$. Also, the indices
$u_1$. Also, the indices ![]() $\ell, m$ will generally be omitted from the symbols
$\ell, m$ will generally be omitted from the symbols ![]() $\boldsymbol {\mathcal {U}}$, etc. The oscillating function (2.1) is divergence-free (
$\boldsymbol {\mathcal {U}}$, etc. The oscillating function (2.1) is divergence-free (![]() $\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {\mathcal {U}}=0$), and when
$\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {\mathcal {U}}=0$), and when ![]() $\mathfrak {w} \mapsto 0$, equals the steady multipolar vortex solutions given in Viúdez (Reference Viúdez2022). We notice that in the general time-dependent case (
$\mathfrak {w} \mapsto 0$, equals the steady multipolar vortex solutions given in Viúdez (Reference Viúdez2022). We notice that in the general time-dependent case (![]() $m\mathfrak {w}\neq 0$), the time-dependent function (2.1) is not a solution of the vorticity equation (1.1).
$m\mathfrak {w}\neq 0$), the time-dependent function (2.1) is not a solution of the vorticity equation (1.1).
Next, we define the rigid motion
where the last equality, which provides the cylindrical components in cylindrical coordinates ![]() $(\rho,\varphi,z)$, shows more clearly that (2.2) is a cylindrical axial motion with swirl, having azimuthal velocity
$(\rho,\varphi,z)$, shows more clearly that (2.2) is a cylindrical axial motion with swirl, having azimuthal velocity ![]() $\rho \mathfrak {w}\hat {\boldsymbol {\varphi }}$ and axial velocity
$\rho \mathfrak {w}\hat {\boldsymbol {\varphi }}$ and axial velocity ![]() $(-2\mathfrak {w}/k)\hat {\boldsymbol {z}}$. Hence
$(-2\mathfrak {w}/k)\hat {\boldsymbol {z}}$. Hence ![]() $\mathfrak {w}$ is the angular speed of the rigid motion. Finally, from (2.1) and (2.2), we define the time-dependent velocity field family
$\mathfrak {w}$ is the angular speed of the rigid motion. Finally, from (2.1) and (2.2), we define the time-dependent velocity field family
which is a time-dependent multipolar solution of (1.1) in spherical geometry. Since the time-dependent velocity oscillations have a local frequency (![]() $m\mathfrak {w}$) proportional to the angular speed of the background flow (
$m\mathfrak {w}$) proportional to the angular speed of the background flow (![]() $\mathfrak {w}$), the time oscillations in (2.3) may be interpreted as inertial oscillations in background flow. The next subsection proves that the family of flows (2.3) satisfies the vorticity equation (1.1).
$\mathfrak {w}$), the time oscillations in (2.3) may be interpreted as inertial oscillations in background flow. The next subsection proves that the family of flows (2.3) satisfies the vorticity equation (1.1).
2.2. Proof that the velocity (2.3) satisfies (1.1)
First, we notice that the vorticity fields of (2.1) and (2.2) are
and therefore the vorticity ![]() $\boldsymbol {\omega }(\boldsymbol {x},t)$ of the total flow is
$\boldsymbol {\omega }(\boldsymbol {x},t)$ of the total flow is
Thus ![]() $\boldsymbol {\mathcal {U}}$ is a Beltrami function (an eigenfunction of the curl operator), and
$\boldsymbol {\mathcal {U}}$ is a Beltrami function (an eigenfunction of the curl operator), and ![]() $\bar {\boldsymbol {\omega }}$ is a constant vertical vorticity. Consequently,
$\bar {\boldsymbol {\omega }}$ is a constant vertical vorticity. Consequently,
that is, the Lamb vector of the oscillating function vanishes, and the Lamb vector of the rigid motion is radial. Therefore, the Lamb vector of the total flow ![]() $\boldsymbol {l}$ simplifies to
$\boldsymbol {l}$ simplifies to
which is the sum of the Lamb vector of the rigid motion ![]() $\bar {\boldsymbol {l}}$ and a nonlinear contribution from the oscillating and rigid motions. We notice from (2.7) and (2.6b) the important property that the azimuthal component of the Lamb vector of this vortex family vanishes:
$\bar {\boldsymbol {l}}$ and a nonlinear contribution from the oscillating and rigid motions. We notice from (2.7) and (2.6b) the important property that the azimuthal component of the Lamb vector of this vortex family vanishes:
Property (2.8) implies that the azimuthal component of the acceleration equation simplifies to
where ![]() $\mathcal {U}_{\varphi }\equiv \boldsymbol {\mathcal {U}}\boldsymbol {\cdot }\boldsymbol {\varphi }$ is the azimuthal component of the oscillating function, and
$\mathcal {U}_{\varphi }\equiv \boldsymbol {\mathcal {U}}\boldsymbol {\cdot }\boldsymbol {\varphi }$ is the azimuthal component of the oscillating function, and ![]() $P$ is the acceleration potential (
$P$ is the acceleration potential (![]() $\boldsymbol {a}=\boldsymbol {\nabla } P$). Clearly, the curl of the Lamb vector of the rigid motion vanishes,
$\boldsymbol {a}=\boldsymbol {\nabla } P$). Clearly, the curl of the Lamb vector of the rigid motion vanishes,
and the curl of the Lamb vector of the total flow (see Appendix A) is
This is a remarkably property. It states that for the family of vortex solutions (2.3), the curl of the Lamb vector ![]() $\boldsymbol {l}$, which is defined through the nonlinear relation
$\boldsymbol {l}$, which is defined through the nonlinear relation ![]() $\boldsymbol {l}\equiv \boldsymbol {\omega }\times \boldsymbol {u}$, satisfies in fact a very simply linear relation with
$\boldsymbol {l}\equiv \boldsymbol {\omega }\times \boldsymbol {u}$, satisfies in fact a very simply linear relation with ![]() $\boldsymbol {\mathcal {U}}$. The curl of the Lamb vector of the total flow
$\boldsymbol {\mathcal {U}}$. The curl of the Lamb vector of the total flow ![]() $\boldsymbol {l}$ is a rotation by an azimuthal angle increment
$\boldsymbol {l}$ is a rotation by an azimuthal angle increment ![]() $\Delta \varphi = {\rm \pi}/2$ of the oscillating velocity function
$\Delta \varphi = {\rm \pi}/2$ of the oscillating velocity function ![]() $-\boldsymbol {\mathcal {U}}$ times
$-\boldsymbol {\mathcal {U}}$ times ![]() $m\mathfrak {w}k$. Expression (2.11) is consistent with
$m\mathfrak {w}k$. Expression (2.11) is consistent with ![]() $\boldsymbol {\mathcal {U}}$ being solenoidal (
$\boldsymbol {\mathcal {U}}$ being solenoidal (![]() $\boldsymbol {\nabla } \boldsymbol {\cdot } \boldsymbol {\mathcal {U}}=0$). Generalized Beltrami flows (
$\boldsymbol {\nabla } \boldsymbol {\cdot } \boldsymbol {\mathcal {U}}=0$). Generalized Beltrami flows (![]() $\boldsymbol {\nabla } \times \boldsymbol {l}=\boldsymbol {0}$) correspond to the steady states with
$\boldsymbol {\nabla } \times \boldsymbol {l}=\boldsymbol {0}$) correspond to the steady states with ![]() $m=0$ (Beltrami flow and rigid flow), or
$m=0$ (Beltrami flow and rigid flow), or ![]() $\mathfrak {w}=0$ (only Beltrami flow), or
$\mathfrak {w}=0$ (only Beltrami flow), or ![]() $k=0$ (Hill's spherical vortex).
$k=0$ (Hill's spherical vortex).
The local rate of change of (2.3) is
and the local rate of change of the total vorticity ![]() $\boldsymbol {\omega }$ is, using (2.4a),
$\boldsymbol {\omega }$ is, using (2.4a),
which cancels with (2.11) and therefore proves that ![]() ${\boldsymbol {u}}$ from (2.3) satisfies (1.1).
${\boldsymbol {u}}$ from (2.3) satisfies (1.1).
2.3. Streamfunctions
Here, we provide the streamfunction ![]() $\boldsymbol {\psi }(\boldsymbol {x},t)$ of the total flow
$\boldsymbol {\psi }(\boldsymbol {x},t)$ of the total flow ![]() $\boldsymbol {u}$. First, we notice that for the oscillating function
$\boldsymbol {u}$. First, we notice that for the oscillating function ![]() $\boldsymbol {\mathcal {U}}$, there exists a function
$\boldsymbol {\mathcal {U}}$, there exists a function
while for the streamfunction ![]() $\bar {\boldsymbol {\psi }}(\rho )$ of the rigid motion, we have
$\bar {\boldsymbol {\psi }}(\rho )$ of the rigid motion, we have
From (2.14) and (2.15), we obtain the streamfunction ![]() $\boldsymbol {\psi }$ of the total flow:
$\boldsymbol {\psi }$ of the total flow:
Using the mathematical identity
applied to ![]() $\boldsymbol {\psi }$, we obtain readily the relation between vorticity and the Laplacian of the streamfunction:
$\boldsymbol {\psi }$, we obtain readily the relation between vorticity and the Laplacian of the streamfunction:
2.4. Frequencies and phase speed
The angular phase of ![]() $\boldsymbol {u}$ from (2.3) is the phase of
$\boldsymbol {u}$ from (2.3) is the phase of ![]() $\boldsymbol {\mathcal {U}}$ from (2.1) and is given by
$\boldsymbol {\mathcal {U}}$ from (2.1) and is given by
The local frequency ![]() $\omega _l$ of the oscillating motion is
$\omega _l$ of the oscillating motion is
while the physical ![]() $\boldsymbol {\nabla }\varTheta$ wavenumber is only azimuthal:
$\boldsymbol {\nabla }\varTheta$ wavenumber is only azimuthal:
The intrinsic frequency ![]() $\omega _i$, defined as the rate of change of the phase
$\omega _i$, defined as the rate of change of the phase ![]() $\varTheta$ from (2.19) for an observer moving with the background rigid flow
$\varTheta$ from (2.19) for an observer moving with the background rigid flow ![]() $\bar {\boldsymbol {u}}$ vanishes since
$\bar {\boldsymbol {u}}$ vanishes since
The phase velocity ![]() $\boldsymbol {\sigma }$ is the velocity satisfying
$\boldsymbol {\sigma }$ is the velocity satisfying
and therefore the phases of the oscillating motion ![]() $\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$ move with the constant azimuthal flow
$\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$ move with the constant azimuthal flow ![]() $\rho \mathfrak {w}\hat {\boldsymbol {\varphi }}$, while the angular phase velocity is
$\rho \mathfrak {w}\hat {\boldsymbol {\varphi }}$, while the angular phase velocity is ![]() $\mathfrak {w}\hat {\boldsymbol {\varphi }}$.
$\mathfrak {w}\hat {\boldsymbol {\varphi }}$.
2.5. Divergence of the Lamb vector
Besides the curl of the Lamb vector given in (2.11), the divergence of the Lamb vector ![]() $\boldsymbol {\nabla } \boldsymbol {\cdot } \boldsymbol {l}$ is also relevant (Hamman, Klewicki & Kirby Reference Hamman, Klewicki and Kirby2008) because for isochoric flows, it equals the Laplacian of the acceleration potential. Introducing the potential
$\boldsymbol {\nabla } \boldsymbol {\cdot } \boldsymbol {l}$ is also relevant (Hamman, Klewicki & Kirby Reference Hamman, Klewicki and Kirby2008) because for isochoric flows, it equals the Laplacian of the acceleration potential. Introducing the potential ![]() $\chi (\boldsymbol {x},t)$ given by
$\chi (\boldsymbol {x},t)$ given by
in Lagrange's expression for the acceleration field ![]() $\boldsymbol {a}(\boldsymbol {x},t)$,
$\boldsymbol {a}(\boldsymbol {x},t)$,
we may write Euler's equation of motion as
and therefore the divergence of the Lamb vector
equals the Laplacian of the potential ![]() $\chi$. If
$\chi$. If ![]() $\boldsymbol {l}(\boldsymbol {x},t)$ is known, then the potential
$\boldsymbol {l}(\boldsymbol {x},t)$ is known, then the potential ![]() $\chi (\boldsymbol {x},t)$ can be obtained by solving the Poisson equation (2.27). Noticing that the divergence of the Lamb vector of the rigid motion is
$\chi (\boldsymbol {x},t)$ can be obtained by solving the Poisson equation (2.27). Noticing that the divergence of the Lamb vector of the rigid motion is
that ![]() $\boldsymbol {\nabla } \times (\rho \hat {\boldsymbol {\varphi }}) = 2 \hat {\boldsymbol {z}}$, and using the mathematical identity
$\boldsymbol {\nabla } \times (\rho \hat {\boldsymbol {\varphi }}) = 2 \hat {\boldsymbol {z}}$, and using the mathematical identity
which implies that
we finally obtain
Thus the divergence of the Lamb vector is the sum of a constant term (![]() $-4\mathfrak {w}^2$) associated with the rigid flow and a term that depends only on the axial and azimuthal components of the oscillating flow
$-4\mathfrak {w}^2$) associated with the rigid flow and a term that depends only on the axial and azimuthal components of the oscillating flow ![]() $\boldsymbol {\mathcal {U}}$. The divergence of
$\boldsymbol {\mathcal {U}}$. The divergence of ![]() $\boldsymbol {l}$ is therefore independent of the
$\boldsymbol {l}$ is therefore independent of the ![]() $\rho$-component of the oscillating flow
$\rho$-component of the oscillating flow ![]() $\boldsymbol {\mathcal {U}}\boldsymbol {\cdot }\hat {\boldsymbol {\rho }}$.
$\boldsymbol {\mathcal {U}}\boldsymbol {\cdot }\hat {\boldsymbol {\rho }}$.
2.6. Acceleration potential
It is possible to obtain the acceleration potential ![]() $P$ for the velocity flow solutions
$P$ for the velocity flow solutions ![]() $\boldsymbol {u}$ in the general case. We introduce the spherical velocity components
$\boldsymbol {u}$ in the general case. We introduce the spherical velocity components
From the azimuthal component of (2.26), using the properties (2.8) and (2.12), we obtain
where ![]() $\mathcal {W}_s(r,\theta ) \exp ({{\rm i} m (\varphi - \mathfrak {w}t)})$ is the azimuthal component of
$\mathcal {W}_s(r,\theta ) \exp ({{\rm i} m (\varphi - \mathfrak {w}t)})$ is the azimuthal component of ![]() $\boldsymbol {\mathcal {U}}$. Since the acceleration potential of the rigid motion satisfies
$\boldsymbol {\mathcal {U}}$. Since the acceleration potential of the rigid motion satisfies
Integration of (2.33) leads to
and therefore the acceleration potential ![]() $P(\boldsymbol {x},t)$ is simply
$P(\boldsymbol {x},t)$ is simply
is the kinetic energy density of the total flow.
3. Solutions satisfying the Navier–Stokes equation
The family of vortex solutions ![]() $\hat {\boldsymbol {u}}(\boldsymbol {x},t)$ defined from
$\hat {\boldsymbol {u}}(\boldsymbol {x},t)$ defined from ![]() $\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$ in (2.1) and
$\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$ in (2.1) and ![]() $\bar {\boldsymbol {u}}(\boldsymbol {x})$ in (2.2) as
$\bar {\boldsymbol {u}}(\boldsymbol {x})$ in (2.2) as
satisfies the Navier–Stokes equation
where ![]() $\nu$ is a constant, assumed complex-valued, momentum diffusivity. We notice that in (3.1), the diffusivity affects the oscillating motion and not the rigid motion
$\nu$ is a constant, assumed complex-valued, momentum diffusivity. We notice that in (3.1), the diffusivity affects the oscillating motion and not the rigid motion ![]() $\bar {\boldsymbol {u}}$ in (2.2), whose Laplacian vanishes. In order to prove that (3.1) satisfies (3.2), we define the new velocity
$\bar {\boldsymbol {u}}$ in (2.2), whose Laplacian vanishes. In order to prove that (3.1) satisfies (3.2), we define the new velocity ![]() $\hat {\boldsymbol {\mathcal {U}}}(\boldsymbol {x},t)$ and vorticity
$\hat {\boldsymbol {\mathcal {U}}}(\boldsymbol {x},t)$ and vorticity ![]() $\hat {\boldsymbol {\mathcal {W}}}(\boldsymbol {x},t)$ fields of the oscillating motion as
$\hat {\boldsymbol {\mathcal {W}}}(\boldsymbol {x},t)$ fields of the oscillating motion as
which imply that
Therefore, the local rate of change of vorticity is
while the curl of the Lamb vector and the Laplacian of the vorticity are
which immediately prove (3.2). For complex-valued ![]() $\nu =\nu _r + {\rm i}\nu _i$, where
$\nu =\nu _r + {\rm i}\nu _i$, where ![]() $\nu _r,\nu _i \in \mathbb {R}$, the imaginary part
$\nu _r,\nu _i \in \mathbb {R}$, the imaginary part ![]() $\nu _i$ represents oscillations of frequency
$\nu _i$ represents oscillations of frequency
in the flow ![]() $\hat {\boldsymbol {u}}(\boldsymbol {x},t)$, which may take place even in the particular case where the oscillations correspond to the zonal spherical harmonics solution (
$\hat {\boldsymbol {u}}(\boldsymbol {x},t)$, which may take place even in the particular case where the oscillations correspond to the zonal spherical harmonics solution (![]() $m=0$).
$m=0$).
4. Transformation under a change of frame translating with constant axial velocity
The velocity solution ![]() $\boldsymbol {u}$ in (2.3) includes the rigid motion
$\boldsymbol {u}$ in (2.3) includes the rigid motion ![]() $\bar {\boldsymbol {u}}(\rho )$ in (2.2), which includes a constant vertical velocity
$\bar {\boldsymbol {u}}(\rho )$ in (2.2), which includes a constant vertical velocity ![]() $(-2\mathfrak {w}/k)\hat {\boldsymbol {z}}$, and an azimuthal velocity
$(-2\mathfrak {w}/k)\hat {\boldsymbol {z}}$, and an azimuthal velocity ![]() $\mathfrak {w}\rho \hat {\boldsymbol {\varphi }}$. It therefore becomes interesting to generalize the solution
$\mathfrak {w}\rho \hat {\boldsymbol {\varphi }}$. It therefore becomes interesting to generalize the solution ![]() $\boldsymbol {u}$ so as to make it valid in a reference frame translating with an arbitrary constant axial velocity (addressed in this section) and rotating with an arbitrary constant angular velocity (addressed in the next section). The mathematical expressions involving changes of frame moving with constant axial velocity become simpler in cylindrical coordinates. We introduce the cylindrical velocity components of the steady multipolar solution
$\boldsymbol {u}$ so as to make it valid in a reference frame translating with an arbitrary constant axial velocity (addressed in this section) and rotating with an arbitrary constant angular velocity (addressed in the next section). The mathematical expressions involving changes of frame moving with constant axial velocity become simpler in cylindrical coordinates. We introduce the cylindrical velocity components of the steady multipolar solution ![]() $\boldsymbol {\mathcal {U}}_0(\boldsymbol {x})\equiv \boldsymbol {\mathcal {U}}(\boldsymbol {x},t;\mathfrak {w}\mapsto 0)$ from (2.1) as
$\boldsymbol {\mathcal {U}}_0(\boldsymbol {x})\equiv \boldsymbol {\mathcal {U}}(\boldsymbol {x},t;\mathfrak {w}\mapsto 0)$ from (2.1) as
In a reference frame translating with constant axial velocity ![]() $-w \hat {\boldsymbol {z}}$, the time-dependent solution (2.3), now written using (4.1) as
$-w \hat {\boldsymbol {z}}$, the time-dependent solution (2.3), now written using (4.1) as
is
Since this is a Galilean transformation of ![]() $\boldsymbol {u}(\boldsymbol {x},t)$, the acceleration
$\boldsymbol {u}(\boldsymbol {x},t)$, the acceleration ![]() $\boldsymbol {a}(\boldsymbol {x},t)$ of the flow remains invariant, which can be verified by noticing that the local and advective rates of change of velocity transform as
$\boldsymbol {a}(\boldsymbol {x},t)$ of the flow remains invariant, which can be verified by noticing that the local and advective rates of change of velocity transform as
where functions ![]() $\boldsymbol {\mathcal {U}}_{0}$ are evaluated at
$\boldsymbol {\mathcal {U}}_{0}$ are evaluated at ![]() $(\rho,\varphi,z-wt)$, so that the acceleration
$(\rho,\varphi,z-wt)$, so that the acceleration ![]() $\tilde {\boldsymbol {a}}(\boldsymbol {x},t)$ remains unchanged,
$\tilde {\boldsymbol {a}}(\boldsymbol {x},t)$ remains unchanged,
and, since ![]() $\boldsymbol {u}$ is a flow solution (
$\boldsymbol {u}$ is a flow solution (![]() $\boldsymbol {\nabla } \times {\boldsymbol {a}} = \boldsymbol {0}$),
$\boldsymbol {\nabla } \times {\boldsymbol {a}} = \boldsymbol {0}$), ![]() $\tilde {\boldsymbol {u}}$ is a flow solution as well (
$\tilde {\boldsymbol {u}}$ is a flow solution as well (![]() $\boldsymbol {\nabla } \times \tilde {\boldsymbol {a}} = \boldsymbol {0}$). We notice also that the phase of this solution,
$\boldsymbol {\nabla } \times \tilde {\boldsymbol {a}} = \boldsymbol {0}$). We notice also that the phase of this solution,
is invariant as well. The velocity solution ![]() $\tilde {\boldsymbol {u}}(\boldsymbol {x},t)$ in (4.3) has a new free parameter
$\tilde {\boldsymbol {u}}(\boldsymbol {x},t)$ in (4.3) has a new free parameter ![]() $w$, which may be interpreted as the (minus) axial velocity of an axially translating reference frame relative to which the velocity solution
$w$, which may be interpreted as the (minus) axial velocity of an axially translating reference frame relative to which the velocity solution ![]() $\boldsymbol {u}(\boldsymbol {x},t)$ in (2.3) becomes
$\boldsymbol {u}(\boldsymbol {x},t)$ in (2.3) becomes ![]() $\tilde {\boldsymbol {u}}(\boldsymbol {x},t)$ in (4.3). Alternatively,
$\tilde {\boldsymbol {u}}(\boldsymbol {x},t)$ in (4.3). Alternatively, ![]() $w\hat {\boldsymbol {z}}$ in
$w\hat {\boldsymbol {z}}$ in ![]() $\tilde {\boldsymbol {u}}(\boldsymbol {x},t)$ from (4.3) may be interpreted as the velocity of displacement of
$\tilde {\boldsymbol {u}}(\boldsymbol {x},t)$ from (4.3) may be interpreted as the velocity of displacement of ![]() $\boldsymbol {u}(\boldsymbol {x},t)$ from (2.3). Regardless of the interpretation, the particular case where
$\boldsymbol {u}(\boldsymbol {x},t)$ from (2.3). Regardless of the interpretation, the particular case where
is characterized by a velocity ![]() $\tilde {\boldsymbol {u}}(\boldsymbol {x},t)$ from (4.3) without rigid, or background, axial velocity.
$\tilde {\boldsymbol {u}}(\boldsymbol {x},t)$ from (4.3) without rigid, or background, axial velocity.
5. Transformation under a change of frame translating and rotating with constant axial and angular velocities
In this section, we extend the results in the previous section to a reference frame that is both translating and rotating with constant axial and angular velocities. Using the cylindrical velocity components of the steady spatially oscillating vortex solution (4.1), we define the time-dependent velocity field
where ![]() $w_0$ is a new vertical velocity parameter. We define, in the usual way, the vorticity
$w_0$ is a new vertical velocity parameter. We define, in the usual way, the vorticity ![]() $\hat {\boldsymbol {\omega }} \equiv \boldsymbol {\nabla } \times \hat {\boldsymbol {u}}$ and Lamb vector
$\hat {\boldsymbol {\omega }} \equiv \boldsymbol {\nabla } \times \hat {\boldsymbol {u}}$ and Lamb vector ![]() $\hat {\boldsymbol {l}} \equiv \hat {\boldsymbol {\omega }} \times \hat {\boldsymbol {u}}$. From these definitions and applying the chain rule to (5.1), we obtain
$\hat {\boldsymbol {l}} \equiv \hat {\boldsymbol {\omega }} \times \hat {\boldsymbol {u}}$. From these definitions and applying the chain rule to (5.1), we obtain
and
where ![]() $\boldsymbol {\mathcal {U}}_0$ in the right-hand side of these and following equations is always evaluated at
$\boldsymbol {\mathcal {U}}_0$ in the right-hand side of these and following equations is always evaluated at ![]() $(\rho,\varphi -\mathfrak {w}t,z-wt)$, whereas those in the left-hand side are evaluated at
$(\rho,\varphi -\mathfrak {w}t,z-wt)$, whereas those in the left-hand side are evaluated at ![]() $(\rho,\varphi,z,t)$. Since the curl of the centripetal acceleration vanishes (
$(\rho,\varphi,z,t)$. Since the curl of the centripetal acceleration vanishes (![]() $\boldsymbol {\nabla } \times (-\varOmega ^2\rho \hat {\boldsymbol {\rho }})=\boldsymbol {0}$), we need only to add to the vorticity equation the curl
$\boldsymbol {\nabla } \times (-\varOmega ^2\rho \hat {\boldsymbol {\rho }})=\boldsymbol {0}$), we need only to add to the vorticity equation the curl ![]() $\boldsymbol {\mathcal {C}}$ of the Coriolis acceleration
$\boldsymbol {\mathcal {C}}$ of the Coriolis acceleration ![]() $2 \varOmega \hat {\boldsymbol {z}} \times \hat {\boldsymbol {u}}$,
$2 \varOmega \hat {\boldsymbol {z}} \times \hat {\boldsymbol {u}}$,
to obtain the vorticity equation in the rotating non-inertial frame
which implies
Therefore, the velocity ![]() $\hat {\boldsymbol {u}}$ in (5.1) is a solution of the vorticity equation in a frame of reference rotating with constant angular velocity
$\hat {\boldsymbol {u}}$ in (5.1) is a solution of the vorticity equation in a frame of reference rotating with constant angular velocity ![]() $\varOmega \hat {\boldsymbol {z}}$ relative to the inertial reference frame when
$\varOmega \hat {\boldsymbol {z}}$ relative to the inertial reference frame when
Thus ![]() $\hat {\boldsymbol {u}}$ in (5.1) is a flow solution in an inertial reference frame (
$\hat {\boldsymbol {u}}$ in (5.1) is a flow solution in an inertial reference frame (![]() $\varOmega =0$) when
$\varOmega =0$) when ![]() $w_0 = -2\mathfrak {w}/k$, which corresponds to the time-dependent oscillating vortex solution
$w_0 = -2\mathfrak {w}/k$, which corresponds to the time-dependent oscillating vortex solution ![]() $\boldsymbol {u}(\boldsymbol {x},t)$ in (2.3). Replacing
$\boldsymbol {u}(\boldsymbol {x},t)$ in (2.3). Replacing ![]() $w_0$ from (5.7) in (5.1), we finally write the solution
$w_0$ from (5.7) in (5.1), we finally write the solution
Thus ![]() $\hat {\boldsymbol {u}}$ in (5.8), with the free parameters
$\hat {\boldsymbol {u}}$ in (5.8), with the free parameters ![]() $u_1,k,l,m,\mathfrak {w} , w , \varOmega$, is a flow solution of the vorticity equation in a reference frame (which is non-inertial when
$u_1,k,l,m,\mathfrak {w} , w , \varOmega$, is a flow solution of the vorticity equation in a reference frame (which is non-inertial when ![]() $\varOmega \neq 0$) moving with velocity
$\varOmega \neq 0$) moving with velocity ![]() $\rho \varOmega \hat {\boldsymbol {\varphi }}$ relative to the inertial one. We notice that now all the terms in the solution
$\rho \varOmega \hat {\boldsymbol {\varphi }}$ relative to the inertial one. We notice that now all the terms in the solution ![]() $\hat {\boldsymbol {u}}$ in (5.8) are relative to the rotating reference frame; for example,
$\hat {\boldsymbol {u}}$ in (5.8) are relative to the rotating reference frame; for example, ![]() $\mathfrak {w}$ in (5.8) is the relative angular speed of the background flow. Solution (5.8) is relevant to geophysical flows as it describes inertial oscillations in vortices relative to a rotating sphere.
$\mathfrak {w}$ in (5.8) is the relative angular speed of the background flow. Solution (5.8) is relevant to geophysical flows as it describes inertial oscillations in vortices relative to a rotating sphere.
Though inertial oscillations are frequently associated with flows observed in a rotating non-inertial reference frame, we have seen here that this is not the case, since the waves exist in an inertial reference frame as long as there is a background rotation ![]() $\mathfrak {w}\ne 0$ and the oscillating function has a wavenumber
$\mathfrak {w}\ne 0$ and the oscillating function has a wavenumber ![]() $m \ne 0$. When the flow is steady in the inertial reference frame (and in the general case the oscillating flow has any wavenumber
$m \ne 0$. When the flow is steady in the inertial reference frame (and in the general case the oscillating flow has any wavenumber ![]() $m$), we have that
$m$), we have that ![]() $\mathfrak {w}=-\varOmega$ in the rotating frame (we notice that this is consistent with
$\mathfrak {w}=-\varOmega$ in the rotating frame (we notice that this is consistent with ![]() $\mathfrak {w}=0$ in the inertial reference frame characterized by
$\mathfrak {w}=0$ in the inertial reference frame characterized by ![]() $\varOmega =0$), and
$\varOmega =0$), and ![]() $w=0$, so solution (5.8) reduces to
$w=0$, so solution (5.8) reduces to
which clearly differs from the solution ![]() $\boldsymbol {u}$ in (2.3) after the substitution
$\boldsymbol {u}$ in (2.3) after the substitution ![]() $\mathfrak {w} \rightarrow -\varOmega$. The fact that (5.9) represents steady motion in the inertial reference frame is immediately proven by setting
$\mathfrak {w} \rightarrow -\varOmega$. The fact that (5.9) represents steady motion in the inertial reference frame is immediately proven by setting ![]() $\varOmega =0$.
$\varOmega =0$.
Clearly, for zonal spherical harmonics (![]() $m=0$) the flow has no azimuthal dependence, so we may write
$m=0$) the flow has no azimuthal dependence, so we may write ![]() $\boldsymbol {\mathcal {U}}(\rho,\varphi -\mathfrak {w}t,z-wt)=\boldsymbol {\mathcal {U}}(\rho,\cdot,z-wt)$ in (5.8), and the relative angular speed
$\boldsymbol {\mathcal {U}}(\rho,\varphi -\mathfrak {w}t,z-wt)=\boldsymbol {\mathcal {U}}(\rho,\cdot,z-wt)$ in (5.8), and the relative angular speed ![]() $\mathfrak {w}$ has no effect in
$\mathfrak {w}$ has no effect in ![]() $\boldsymbol {\mathcal {U}}$, so the vortex remains steady in a reference frame translating with velocity
$\boldsymbol {\mathcal {U}}$, so the vortex remains steady in a reference frame translating with velocity ![]() $w\hat {\boldsymbol {z}}$. This fact, and the resulting exterior inertial waves associated with the axial translation of the vortex
$w\hat {\boldsymbol {z}}$. This fact, and the resulting exterior inertial waves associated with the axial translation of the vortex ![]() $w$ and with the angular speed of the rotating frame
$w$ and with the angular speed of the rotating frame ![]() $\varOmega$, was addressed by Scase & Terry (Reference Scase and Terry2018) in the particular case
$\varOmega$, was addressed by Scase & Terry (Reference Scase and Terry2018) in the particular case ![]() $\ell =1$ for the spherical vortex of Hill (
$\ell =1$ for the spherical vortex of Hill (![]() $k=0$) and the swirling spherical vortex of Hicks–Moffatt (
$k=0$) and the swirling spherical vortex of Hicks–Moffatt (![]() $k\ne 0$).
$k\ne 0$).
Let us now consider a solution ![]() $\hat {\boldsymbol {u}}$ from (5.1) in an inertial reference frame (
$\hat {\boldsymbol {u}}$ from (5.1) in an inertial reference frame (![]() $\varOmega =0$). The flow with
$\varOmega =0$). The flow with
is a velocity solution with vanishing background axial flow. The velocity ![]() $\check {\boldsymbol {u}}(\boldsymbol {x},t)$ of this particular solution is
$\check {\boldsymbol {u}}(\boldsymbol {x},t)$ of this particular solution is
Applying the chain rule to the solution with no background axial flow ![]() $\check {\boldsymbol {u}}(\boldsymbol {x},t)$ in (5.11), we find that the displacement velocity
$\check {\boldsymbol {u}}(\boldsymbol {x},t)$ in (5.11), we find that the displacement velocity ![]() $\check {\boldsymbol {v}}$ of the velocity field
$\check {\boldsymbol {v}}$ of the velocity field ![]() $\check {\boldsymbol {u}}$, that is, satisfying the equation
$\check {\boldsymbol {u}}$, that is, satisfying the equation
Thus an observer moving with velocity ![]() $\check {\boldsymbol {v}}$ from (5.12b) will see no rate of change of velocity, as is also inferred directly from (5.11). The phase velocity
$\check {\boldsymbol {v}}$ from (5.12b) will see no rate of change of velocity, as is also inferred directly from (5.11). The phase velocity ![]() $\boldsymbol {\sigma }=\rho \mathfrak {w}\hat {\boldsymbol {\varphi }}$ in (2.23) is azimuthal, therefore transverse to the axial direction, and it remains invariant to any inertial observer moving with an arbitrary axial velocity.
$\boldsymbol {\sigma }=\rho \mathfrak {w}\hat {\boldsymbol {\varphi }}$ in (2.23) is azimuthal, therefore transverse to the axial direction, and it remains invariant to any inertial observer moving with an arbitrary axial velocity.
The velocity fields found here – ![]() $\boldsymbol {u}$ in (2.3), and its generalization to a translating and rotating reference frame
$\boldsymbol {u}$ in (2.3), and its generalization to a translating and rotating reference frame ![]() $\hat {\boldsymbol {u}}$ in (5.8) – are solutions to the nonlinear vorticity equation (1.1). However, since the nonlinear term
$\hat {\boldsymbol {u}}$ in (5.8) – are solutions to the nonlinear vorticity equation (1.1). However, since the nonlinear term ![]() $\boldsymbol {\nabla }\times \boldsymbol {l}=-{\rm i}m\mathfrak {w}k\boldsymbol {\mathcal {U}}$ in (2.11) becomes a linear function of the velocity oscillation
$\boldsymbol {\nabla }\times \boldsymbol {l}=-{\rm i}m\mathfrak {w}k\boldsymbol {\mathcal {U}}$ in (2.11) becomes a linear function of the velocity oscillation ![]() $\boldsymbol {\mathcal {U}}$, these solutions satisfy different linear wave equations as well. These wave equations are considered in the next section.
$\boldsymbol {\mathcal {U}}$, these solutions satisfy different linear wave equations as well. These wave equations are considered in the next section.
6. Wave equations
In order to obtain the wave equations for ![]() $\boldsymbol {u}$ and
$\boldsymbol {u}$ and ![]() $\boldsymbol {\omega }$ (as well as for all their higher-degree curl fields), we notice that since
$\boldsymbol {\omega }$ (as well as for all their higher-degree curl fields), we notice that since ![]() $\boldsymbol {\nabla }\times \boldsymbol {\mathcal {U}}=-k\boldsymbol {\mathcal {U}}$ from (2.4a), and
$\boldsymbol {\nabla }\times \boldsymbol {\mathcal {U}}=-k\boldsymbol {\mathcal {U}}$ from (2.4a), and ![]() $\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {\mathcal {U}}=0$, the velocity oscillations
$\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {\mathcal {U}}=0$, the velocity oscillations ![]() $\boldsymbol {\mathcal {U}}$,
$\boldsymbol {\mathcal {U}}$, ![]() $\boldsymbol {\mathcal {W}}$, and all successive curls, satisfy the Helmholtz equations
$\boldsymbol {\mathcal {W}}$, and all successive curls, satisfy the Helmholtz equations
Using the local rate of change of ![]() $\boldsymbol {u}$ from (2.12) and
$\boldsymbol {u}$ from (2.12) and ![]() $\boldsymbol {\omega }$ from (2.13), we see that
$\boldsymbol {\omega }$ from (2.13), we see that ![]() $\boldsymbol {\mathcal {U}}$, and hence
$\boldsymbol {\mathcal {U}}$, and hence ![]() $\boldsymbol {u}$,
$\boldsymbol {u}$, ![]() $\boldsymbol {\omega }$, and all successive curls, satisfy
$\boldsymbol {\omega }$, and all successive curls, satisfy
These equations are similar to the time-dependent Schrödinger equations for a massive free particle. The similarity of these equations to the Schrödinger and Klein–Gordon equations is addressed in Appendix B. The second partial time derivatives of (6.2) imply that
and therefore ![]() $\boldsymbol {u}$ and
$\boldsymbol {u}$ and ![]() $\boldsymbol {\omega }$ satisfy the classical wave equations
$\boldsymbol {\omega }$ satisfy the classical wave equations
where the squared wave speed is
The fact that both velocity ![]() $\boldsymbol {u}$ and vorticity
$\boldsymbol {u}$ and vorticity ![]() $\boldsymbol {\omega }$ satisfy the wave equations (6.4a,b) makes it appealing to investigate the analogy between the propagation of these vortex oscillations and the propagation of electromagnetic waves in vacuum (see § 7). This analogy is interesting also because the angular phase velocity
$\boldsymbol {\omega }$ satisfy the wave equations (6.4a,b) makes it appealing to investigate the analogy between the propagation of these vortex oscillations and the propagation of electromagnetic waves in vacuum (see § 7). This analogy is interesting also because the angular phase velocity ![]() $\mathfrak {w}\hat {\boldsymbol {\varphi }}$ is transverse to the axial velocity and therefore is independent of the inertial reference frame translating with constant axial velocity addressed in § 4.
$\mathfrak {w}\hat {\boldsymbol {\varphi }}$ is transverse to the axial velocity and therefore is independent of the inertial reference frame translating with constant axial velocity addressed in § 4.
7. Maxwell equations for the propagation of electromagnetic waves in vacuum
We define the time-dependent fields ![]() $\boldsymbol {E}$ and
$\boldsymbol {E}$ and ![]() $\boldsymbol {B}$ from the velocity
$\boldsymbol {B}$ from the velocity ![]() $\boldsymbol {u}(\rho,\varphi,z,t)$ as
$\boldsymbol {u}(\rho,\varphi,z,t)$ as
where ![]() ${\mathrm{c}}$ is a constant identified with the speed of the electromagnetic waves in vacuum, but in principle independent of
${\mathrm{c}}$ is a constant identified with the speed of the electromagnetic waves in vacuum, but in principle independent of ![]() $c$. Fields
$c$. Fields ![]() $\boldsymbol {E}$ and
$\boldsymbol {E}$ and ![]() $\boldsymbol {B}$ are solenoidal and therefore satisfy Gauss's laws for the magnetic (
$\boldsymbol {B}$ are solenoidal and therefore satisfy Gauss's laws for the magnetic (![]() $\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {B}=0$) and electric (
$\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {B}=0$) and electric (![]() $\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {E}=0$) fields in the absence of electric charges. Fields (7.1), in the Gaussian units convention, satisfy also Faraday's law of induction
$\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {E}=0$) fields in the absence of electric charges. Fields (7.1), in the Gaussian units convention, satisfy also Faraday's law of induction
for any ![]() $\boldsymbol {u}(\boldsymbol {x},t)$. We note that
$\boldsymbol {u}(\boldsymbol {x},t)$. We note that
If the density current ![]() $\boldsymbol {J}$ is defined as
$\boldsymbol {J}$ is defined as
then Ampère's law,
is also satisfied. Though the current density is ![]() $\boldsymbol {J} \neq \boldsymbol {0}$, there is no charge density since
$\boldsymbol {J} \neq \boldsymbol {0}$, there is no charge density since ![]() $\boldsymbol {\nabla } \boldsymbol {\cdot } \boldsymbol {J}=0$. The fulfilment of the Maxwell equations in the absence of charge density, using the definitions (7.1), does not suffice to obtain wave equations for
$\boldsymbol {\nabla } \boldsymbol {\cdot } \boldsymbol {J}=0$. The fulfilment of the Maxwell equations in the absence of charge density, using the definitions (7.1), does not suffice to obtain wave equations for ![]() $\boldsymbol {E}$ and
$\boldsymbol {E}$ and ![]() $\boldsymbol {B}$. The additional constraint that the density current vanishes,
$\boldsymbol {B}$. The additional constraint that the density current vanishes, ![]() $\boldsymbol {J}=\boldsymbol {0}$, must be assumed in (7.4), or equivalently set the wave speed
$\boldsymbol {J}=\boldsymbol {0}$, must be assumed in (7.4), or equivalently set the wave speed
then Ampère's law in the absence of charge density,
is also satisfied. In this case, both ![]() $\boldsymbol {E}$ and
$\boldsymbol {E}$ and ![]() $\boldsymbol {B}$, as defined by (7.1), satisfy wave equations with phase velocity
$\boldsymbol {B}$, as defined by (7.1), satisfy wave equations with phase velocity ![]() $c$,
$c$,
which are the Maxwell equations for electromagnetic waves in vacuum. The phase speed ![]() $c$ in (7.6) does not depend on the
$c$ in (7.6) does not depend on the ![]() $w$ reference frame, but depends on the radial
$w$ reference frame, but depends on the radial ![]() $k$ and azimuthal
$k$ and azimuthal ![]() $m$ wavenumbers. This is so because the velocity solution
$m$ wavenumbers. This is so because the velocity solution ![]() $\boldsymbol {u}(r,\theta,\varphi,t)$ in (2.3), with free parameters
$\boldsymbol {u}(r,\theta,\varphi,t)$ in (2.3), with free parameters ![]() $u_1, \mathfrak {w}, k , \ell , m$, is still too general to be applied directly to any particular physical process. In order to apply the hydrodynamical flow solution (2.3) as a model for the propagation of electromagnetic waves in ‘vacuum’, which is experimentally known to have a constant – and independent of the inertial reference frame – wave speed
$u_1, \mathfrak {w}, k , \ell , m$, is still too general to be applied directly to any particular physical process. In order to apply the hydrodynamical flow solution (2.3) as a model for the propagation of electromagnetic waves in ‘vacuum’, which is experimentally known to have a constant – and independent of the inertial reference frame – wave speed ![]() $c$, we establish an additional condition, namely, that frequency
$c$, we establish an additional condition, namely, that frequency ![]() $\mathfrak {w}$ and wavenumbers
$\mathfrak {w}$ and wavenumbers ![]() $k,m$ are related through a dispersion relation
$k,m$ are related through a dispersion relation
where ![]() $c_0$ is a constant wave speed. Constraint (7.9) is independent of the
$c_0$ is a constant wave speed. Constraint (7.9) is independent of the ![]() $w$ reference frame. This condition immediately gives
$w$ reference frame. This condition immediately gives ![]() $\mathfrak {w} = \pm c_0 k/m$ and therefore
$\mathfrak {w} = \pm c_0 k/m$ and therefore ![]() $c=c_0$ in (7.6) is a constant independent of the
$c=c_0$ in (7.6) is a constant independent of the ![]() $w$ reference frame. The constant
$w$ reference frame. The constant
(positive relation assumed) is identified with the speed of the electromagnetic waves in ‘vacuum’. Once ![]() $c_0$ is given a universal value, say
$c_0$ is given a universal value, say ![]() $c_0=1$, the remaining free parameters of the hydrodynamical model
$c_0=1$, the remaining free parameters of the hydrodynamical model ![]() $\boldsymbol {u}(r,\theta,\varphi,t)$ from (2.3) are the amplitude velocity of the oscillating term
$\boldsymbol {u}(r,\theta,\varphi,t)$ from (2.3) are the amplitude velocity of the oscillating term ![]() $u_1$, the frequency
$u_1$, the frequency ![]() $\mathfrak {w}$ (or the radial wavenumber
$\mathfrak {w}$ (or the radial wavenumber ![]() $k = m\mathfrak {w}/c_0$), and the degree
$k = m\mathfrak {w}/c_0$), and the degree ![]() $\ell$ (or number of nodal lines). Interestingly, in the particular case of azimuthal wavenumber
$\ell$ (or number of nodal lines). Interestingly, in the particular case of azimuthal wavenumber ![]() $m=2$, the wave speed in (7.10),
$m=2$, the wave speed in (7.10), ![]() $c_0=2\mathfrak {w}/k$, equals the axial velocity of displacement
$c_0=2\mathfrak {w}/k$, equals the axial velocity of displacement ![]() $w$ in (5.10) for the velocity solution with vanishing background axial flow (vanishing axial flow at infinity). For such a velocity solution, the wave speed and axial speed of displacement coincide. Another interesting property of these hydrodynamical models is that they admit piecewise solutions, as explained in § 10, making it possible to assign to these flows both continuous field and discrete particle-like properties.
$w$ in (5.10) for the velocity solution with vanishing background axial flow (vanishing axial flow at infinity). For such a velocity solution, the wave speed and axial speed of displacement coincide. Another interesting property of these hydrodynamical models is that they admit piecewise solutions, as explained in § 10, making it possible to assign to these flows both continuous field and discrete particle-like properties.
8. Superposition
This section addresses the relevant question of whether the superposition of two members of the vortex family ![]() $\boldsymbol {u}(\boldsymbol {x},t)$ in (2.3) is a flow solution as well. First, we denote a particular member
$\boldsymbol {u}(\boldsymbol {x},t)$ in (2.3) is a flow solution as well. First, we denote a particular member ![]() $\boldsymbol {u}_{i}(\boldsymbol {x},t)$ of this vortex family with the index
$\boldsymbol {u}_{i}(\boldsymbol {x},t)$ of this vortex family with the index ![]() $i$ as
$i$ as
and we want to know whether the superposition
satisfies the vorticity equation (1.1). We define in the usual way ![]() $\boldsymbol {u}_{i} \equiv \boldsymbol {\mathcal {U}}_i + \bar {\boldsymbol {u}}_{i}$, the vorticity
$\boldsymbol {u}_{i} \equiv \boldsymbol {\mathcal {U}}_i + \bar {\boldsymbol {u}}_{i}$, the vorticity ![]() $\boldsymbol {\omega }_{i} \equiv \boldsymbol {\nabla } \times \boldsymbol {u}_{i} = \boldsymbol {\mathcal {W}}_i + \bar {\boldsymbol {\omega }}_{i}$, and the Lamb vector
$\boldsymbol {\omega }_{i} \equiv \boldsymbol {\nabla } \times \boldsymbol {u}_{i} = \boldsymbol {\mathcal {W}}_i + \bar {\boldsymbol {\omega }}_{i}$, and the Lamb vector ![]() $\boldsymbol {l}_{i} \equiv \boldsymbol {\omega }_{i} \times \boldsymbol {u}_{i}$. Since the particular solutions satisfy the relations
$\boldsymbol {l}_{i} \equiv \boldsymbol {\omega }_{i} \times \boldsymbol {u}_{i}$. Since the particular solutions satisfy the relations
All that remains from the vorticity equation for (8.2) is the curl of the crossing terms
 \begin{align} & \boldsymbol{\nabla} \times \left( \boldsymbol{\omega}_1 \times \boldsymbol{u}_2 +\boldsymbol{\omega}_2 \times \boldsymbol{u}_1 \right) \nonumber\\ &\quad = \boldsymbol{\nabla} \times \left[ \left( \boldsymbol{\mathcal{W}}_1 + \bar{\boldsymbol{\omega}}_1 \right) \times \left( \boldsymbol{\mathcal{U}}_2 + \bar{\boldsymbol{u}}_2 \right) + \left( \boldsymbol{\mathcal{W}}_2 + \bar{\boldsymbol{\omega}}_2 \right) \times \left( \boldsymbol{\mathcal{U}}_1 + \bar{\boldsymbol{u}}_1 \right) \right] \nonumber\\ &\quad = \boldsymbol{\nabla} \times \left[ \left( k_2 - k_1 \right) \left( \boldsymbol{\mathcal{U}}_1 \times \boldsymbol{\mathcal{U}}_2 \right) + \left( \bar{\boldsymbol{\omega}}_1 \times \bar{\boldsymbol{u}}_2 + \bar{\boldsymbol{\omega}}_2 \times \bar{\boldsymbol{u}}_1 \right) \right. \nonumber\\ &\left.\qquad {}- \boldsymbol{\mathcal{U}}_1 \times \left( k_1 \bar{\boldsymbol{u}}_2 + \bar{\boldsymbol{\omega}}_2 \right) - \boldsymbol{\mathcal{U}}_2 \times \left( k_2 \bar{\boldsymbol{u}}_1 + \bar{\boldsymbol{\omega}}_1 \right) \right] . \end{align}
\begin{align} & \boldsymbol{\nabla} \times \left( \boldsymbol{\omega}_1 \times \boldsymbol{u}_2 +\boldsymbol{\omega}_2 \times \boldsymbol{u}_1 \right) \nonumber\\ &\quad = \boldsymbol{\nabla} \times \left[ \left( \boldsymbol{\mathcal{W}}_1 + \bar{\boldsymbol{\omega}}_1 \right) \times \left( \boldsymbol{\mathcal{U}}_2 + \bar{\boldsymbol{u}}_2 \right) + \left( \boldsymbol{\mathcal{W}}_2 + \bar{\boldsymbol{\omega}}_2 \right) \times \left( \boldsymbol{\mathcal{U}}_1 + \bar{\boldsymbol{u}}_1 \right) \right] \nonumber\\ &\quad = \boldsymbol{\nabla} \times \left[ \left( k_2 - k_1 \right) \left( \boldsymbol{\mathcal{U}}_1 \times \boldsymbol{\mathcal{U}}_2 \right) + \left( \bar{\boldsymbol{\omega}}_1 \times \bar{\boldsymbol{u}}_2 + \bar{\boldsymbol{\omega}}_2 \times \bar{\boldsymbol{u}}_1 \right) \right. \nonumber\\ &\left.\qquad {}- \boldsymbol{\mathcal{U}}_1 \times \left( k_1 \bar{\boldsymbol{u}}_2 + \bar{\boldsymbol{\omega}}_2 \right) - \boldsymbol{\mathcal{U}}_2 \times \left( k_2 \bar{\boldsymbol{u}}_1 + \bar{\boldsymbol{\omega}}_1 \right) \right] . \end{align}Clearly, the curl of the terms
vanishes, and also we can express
Thus for identical radial wavenumbers ![]() $k_1 = k_2 = k$, the curl of the crossing terms (8.4) reduces to the simple expression
$k_1 = k_2 = k$, the curl of the crossing terms (8.4) reduces to the simple expression
Term (8.7) vanishes, and therefore ![]() $\boldsymbol {u}_1$ and
$\boldsymbol {u}_1$ and ![]() $\boldsymbol {u}_2$ are superposable, in different cases. (i) The steady oscillating flows vanish (
$\boldsymbol {u}_2$ are superposable, in different cases. (i) The steady oscillating flows vanish (![]() $u_{1,1} = u_{1,2} = 0$), leaving only the steady rigid flows (
$u_{1,1} = u_{1,2} = 0$), leaving only the steady rigid flows (![]() $\mathfrak {w}_1 \ne 0$ and/or
$\mathfrak {w}_1 \ne 0$ and/or ![]() $\mathfrak {w}_2 \ne 0$). (ii) The trivial case in which the steady oscillating flows cancel,
$\mathfrak {w}_2 \ne 0$). (ii) The trivial case in which the steady oscillating flows cancel, ![]() $\boldsymbol {\mathcal {U}}_{1}=-\boldsymbol {\mathcal {U}}_{2}$ (
$\boldsymbol {\mathcal {U}}_{1}=-\boldsymbol {\mathcal {U}}_{2}$ (![]() $u_{1,1} = -u_{1,2}$,
$u_{1,1} = -u_{1,2}$, ![]() $m_1 = m_2$ and
$m_1 = m_2$ and ![]() $\mathfrak {w}_1=\mathfrak {w}_2$). (iii) Both flows are steady because
$\mathfrak {w}_1=\mathfrak {w}_2$). (iii) Both flows are steady because ![]() $m_1=m_2=0$ and there is rigid flow (
$m_1=m_2=0$ and there is rigid flow (![]() $\mathfrak {w}_1 \ne 0$ and/or
$\mathfrak {w}_1 \ne 0$ and/or ![]() $\mathfrak {w}_2 \ne 0$). (iv) Both flows are steady because
$\mathfrak {w}_2 \ne 0$). (iv) Both flows are steady because ![]() $\mathfrak {w}_1 = \mathfrak {w}_2 = 0$ and therefore there is no rigid flow (this case corresponds to the superposition of solutions
$\mathfrak {w}_1 = \mathfrak {w}_2 = 0$ and therefore there is no rigid flow (this case corresponds to the superposition of solutions ![]() $\boldsymbol {\mathcal {U}}(\boldsymbol {x},0)$). (v) One of the flows is steady with no rigid motion (say
$\boldsymbol {\mathcal {U}}(\boldsymbol {x},0)$). (v) One of the flows is steady with no rigid motion (say ![]() $\mathfrak {w}_1=0$ and
$\mathfrak {w}_1=0$ and ![]() $m_1 \ne 0$), and the other one is steady with rigid motion (
$m_1 \ne 0$), and the other one is steady with rigid motion (![]() $m_2=0$ and
$m_2=0$ and ![]() $\mathfrak {w}_2\ne 0$).
$\mathfrak {w}_2\ne 0$).
9. Stability of the steady vortices with  $m=0$
$m=0$
The time-dependent vortex solution ![]() $\boldsymbol {u}(\boldsymbol {x},t)$ in (2.3) is very useful to investigate the stability of spherical vortices and in particular the stability of the steady Hicks–Moffatt vortex. The Hicks–Moffatt vortex is a three-dimensional piecewise vortex whose interior vortical velocity is given by
$\boldsymbol {u}(\boldsymbol {x},t)$ in (2.3) is very useful to investigate the stability of spherical vortices and in particular the stability of the steady Hicks–Moffatt vortex. The Hicks–Moffatt vortex is a three-dimensional piecewise vortex whose interior vortical velocity is given by ![]() $\boldsymbol {u}$ in (2.3) for the particular case of degree
$\boldsymbol {u}$ in (2.3) for the particular case of degree ![]() $\ell =1$ (one nodal line) and order
$\ell =1$ (one nodal line) and order ![]() $m=0$ (zonal spherical harmonics solution). Thus the stationarity of this vortex is due not to the absence of background rotation
$m=0$ (zonal spherical harmonics solution). Thus the stationarity of this vortex is due not to the absence of background rotation ![]() $\mathfrak {w}\rho$, but to the fact that since
$\mathfrak {w}\rho$, but to the fact that since ![]() $m=0$, the velocity field does not depend on the azimuthal angle
$m=0$, the velocity field does not depend on the azimuthal angle ![]() $\varphi$. If the vortex boundary
$\varphi$. If the vortex boundary ![]() $r_n$ is taken at any zero
$r_n$ is taken at any zero ![]() $j_{5/2,n}$ of
$j_{5/2,n}$ of ![]() ${\rm j}_2(\,{\cdot}\, )$ – that is, if
${\rm j}_2(\,{\cdot}\, )$ – that is, if ![]() $k r_n = j_{5/2,n}$, and the ratio
$k r_n = j_{5/2,n}$, and the ratio ![]() $\mathfrak {w}/u_1 = \sqrt {3/{\rm \pi} }\,{\rm j}_0(k r_n)/6$ (employing the parameter
$\mathfrak {w}/u_1 = \sqrt {3/{\rm \pi} }\,{\rm j}_0(k r_n)/6$ (employing the parameter ![]() $u_1$ in (2.3)) – then these spherical vortex boundary surfaces are stagnation surfaces, and the exterior irrotational flow vanishes. The stability of these zonal vortices is investigated next.
$u_1$ in (2.3)) – then these spherical vortex boundary surfaces are stagnation surfaces, and the exterior irrotational flow vanishes. The stability of these zonal vortices is investigated next.
Let us consider the oscillating function ![]() $\bar {\boldsymbol {\mathcal {U}}}(r,\theta )$ defined from
$\bar {\boldsymbol {\mathcal {U}}}(r,\theta )$ defined from ![]() $\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$ in (2.1) by
$\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$ in (2.1) by
where the degree ![]() $\ell$ is shown for clarity. In such a flow, having an amplitude term
$\ell$ is shown for clarity. In such a flow, having an amplitude term ![]() $u_1(t)$,
$u_1(t)$,
is in the form (2.3), and therefore (9.2) is a velocity solution when ![]() $u_1(t)=\hat {u}_1$ is a constant. We notice that since the spherical harmonics basis vectors are not normalized, the weight
$u_1(t)=\hat {u}_1$ is a constant. We notice that since the spherical harmonics basis vectors are not normalized, the weight ![]() $u_1(t)$ is not the total velocity amplitude of the oscillation. We then force
$u_1(t)$ is not the total velocity amplitude of the oscillation. We then force ![]() $\boldsymbol {u}_{1}$ to be steady by setting
$\boldsymbol {u}_{1}$ to be steady by setting ![]() $m_1=0$, but keep the background flow
$m_1=0$, but keep the background flow ![]() $\mathfrak {w}_1 \ne 0$. We then add to the steady velocity
$\mathfrak {w}_1 \ne 0$. We then add to the steady velocity ![]() $\boldsymbol {u}_1$ a perturbation velocity with time-dependent amplitude
$\boldsymbol {u}_1$ a perturbation velocity with time-dependent amplitude ![]() $u_2(t)$, azimuthal wavenumber
$u_2(t)$, azimuthal wavenumber ![]() $m_2\ne 0$, and local frequency
$m_2\ne 0$, and local frequency ![]() $m_2\mathfrak {w}_2\ne 0$:
$m_2\mathfrak {w}_2\ne 0$:
Adding (9.3) to (9.2), we obtain the total velocity
and we want to know when this velocity ![]() $\boldsymbol {u}(\boldsymbol {x},t)$ is a flow solution of the vorticity equation. It turns out that
$\boldsymbol {u}(\boldsymbol {x},t)$ is a flow solution of the vorticity equation. It turns out that ![]() $\boldsymbol {u}(\boldsymbol {x},t)$ in (9.4) is already in the form (2.3), and therefore (9.4) is a velocity solution when
$\boldsymbol {u}(\boldsymbol {x},t)$ in (9.4) is already in the form (2.3), and therefore (9.4) is a velocity solution when ![]() $m_2\in \{ -\ell,\ldots,-1,1,\ldots,\ell \}$, the angular frequency
$m_2\in \{ -\ell,\ldots,-1,1,\ldots,\ell \}$, the angular frequency ![]() $\mathfrak {w}_2$ of the oscillation equals the angular speed of the background flow
$\mathfrak {w}_2$ of the oscillation equals the angular speed of the background flow ![]() $\mathfrak {w}_1$,
$\mathfrak {w}_1$,
and the velocity amplitude is constant,
Condition (9.6) is satisfied when
where ![]() $\hat {u}_1$ and
$\hat {u}_1$ and ![]() $\hat {u}_2$ are constant amplitudes, in such a way that
$\hat {u}_2$ are constant amplitudes, in such a way that ![]() $\boldsymbol {u}(\boldsymbol {x},t)$ in (9.4) may be written as
$\boldsymbol {u}(\boldsymbol {x},t)$ in (9.4) may be written as
which means that the addition of any perturbation with constant amplitude ![]() $\hat {u}_2$, no matter how small and with any permissible azimuthal wavenumber
$\hat {u}_2$, no matter how small and with any permissible azimuthal wavenumber ![]() $m_2\neq 0$, to the steady velocity
$m_2\neq 0$, to the steady velocity ![]() $\boldsymbol {u}_1$ that has a background flow characterized by the angular speed
$\boldsymbol {u}_1$ that has a background flow characterized by the angular speed ![]() $\mathfrak {w}$, will cause inertial oscillations of amplitude
$\mathfrak {w}$, will cause inertial oscillations of amplitude ![]() $\|\hat {u}_1\bar {\boldsymbol {\mathcal {U}}}_{\ell }\|$ and local frequency
$\|\hat {u}_1\bar {\boldsymbol {\mathcal {U}}}_{\ell }\|$ and local frequency ![]() $m_2\mathfrak {w}$.
$m_2\mathfrak {w}$.
10. Piecewise solutions
The spatial domain of the rotational flows described in the previous sections is an unbounded region. It is often convenient, however, to work with spatially bounded velocity solutions by assuming that the rotational flow is confined within a spherical region – usually a sphere, but a spherical shell is also possible – while outside this vortical region the flow is potential. The natural choice for selecting the radius of any spherical boundary surface to the velocity ![]() $\boldsymbol {\mathcal {U}}$ in (2.1) is at the zeros of
$\boldsymbol {\mathcal {U}}$ in (2.1) is at the zeros of ![]() ${\rm j}_{\ell }(\,{\cdot}\, )$, i.e.
${\rm j}_{\ell }(\,{\cdot}\, )$, i.e. ![]() $k r_p = \jmath _{\ell,p} \equiv j_{\ell +1/2,p}$, because at these radial distances the velocity oscillation has only
$k r_p = \jmath _{\ell,p} \equiv j_{\ell +1/2,p}$, because at these radial distances the velocity oscillation has only ![]() $\boldsymbol {\varPsi }$-component,
$\boldsymbol {\varPsi }$-component,
and vanishing exterior flow conditions are consistent because there is no velocity jump in the radial direction, so there are no shock waves, which would not be permissible for isochoric flows. The vortex boundary, regarding the discontinuity in the azimuthal component of the background flow ![]() $\rho \mathfrak {w}\hat {\boldsymbol {\varphi }}$, is a vortex sheet (first-order singular surface where the velocity jump is only tangential). The axial component of the background flow
$\rho \mathfrak {w}\hat {\boldsymbol {\varphi }}$, is a vortex sheet (first-order singular surface where the velocity jump is only tangential). The axial component of the background flow ![]() $w=-2\mathfrak {w}/k$ is not problematic since it vanishes in the steadily translating solutions
$w=-2\mathfrak {w}/k$ is not problematic since it vanishes in the steadily translating solutions ![]() $\check {\boldsymbol {u}}(\boldsymbol {x},t)$ of (5.11).
$\check {\boldsymbol {u}}(\boldsymbol {x},t)$ of (5.11).
Nevertheless, it is possible to provide the exterior irrotational velocity ![]() $\tilde {\boldsymbol {\mathcal {U}}}_p(r,\theta,\varphi,t)$ for the oscillating component, which is given by
$\tilde {\boldsymbol {\mathcal {U}}}_p(r,\theta,\varphi,t)$ for the oscillating component, which is given by
 $$\begin{gather} \tilde{\boldsymbol{\mathcal{U}}}_p(r,\theta,\varphi,t) = u_1\,\frac{ {\rm j}_{\ell+1}(\jmath_{\ell,p})}{2\ell+1} \left[ \ell(\ell+1) \left( \left( \frac{kr}{\jmath_{\ell,p}} \right)^{ \ell -1} -\left( \frac{kr}{\jmath_{\ell,p}} \right)^{-\ell -2} \right) \boldsymbol{Y}_{\ell}^{m}(\theta,\varphi) \right. \nonumber\\ \left.{}+\left( (\ell+1) \left( \frac{kr}{\jmath_{\ell,p}} \right)^{ \ell -1} + \ell \left( \frac{kr}{\jmath_{\ell,p}} \right)^{-\ell -2} \right) \boldsymbol{\varPsi}_{\ell}^{m}(\theta,\varphi) \right] {\rm e}^{-{\rm i} m \mathfrak{w}t} . \end{gather}$$
$$\begin{gather} \tilde{\boldsymbol{\mathcal{U}}}_p(r,\theta,\varphi,t) = u_1\,\frac{ {\rm j}_{\ell+1}(\jmath_{\ell,p})}{2\ell+1} \left[ \ell(\ell+1) \left( \left( \frac{kr}{\jmath_{\ell,p}} \right)^{ \ell -1} -\left( \frac{kr}{\jmath_{\ell,p}} \right)^{-\ell -2} \right) \boldsymbol{Y}_{\ell}^{m}(\theta,\varphi) \right. \nonumber\\ \left.{}+\left( (\ell+1) \left( \frac{kr}{\jmath_{\ell,p}} \right)^{ \ell -1} + \ell \left( \frac{kr}{\jmath_{\ell,p}} \right)^{-\ell -2} \right) \boldsymbol{\varPsi}_{\ell}^{m}(\theta,\varphi) \right] {\rm e}^{-{\rm i} m \mathfrak{w}t} . \end{gather}$$
Solution ![]() $\tilde {\boldsymbol {\mathcal {U}}}_p(r,\theta,\varphi,t)$ is irrotational (
$\tilde {\boldsymbol {\mathcal {U}}}_p(r,\theta,\varphi,t)$ is irrotational (![]() $\boldsymbol {\nabla }\times \tilde {\boldsymbol {\mathcal {U}}}_p=\boldsymbol {0}$) and satisfies continuity at the spherical boundaries, i.e.
$\boldsymbol {\nabla }\times \tilde {\boldsymbol {\mathcal {U}}}_p=\boldsymbol {0}$) and satisfies continuity at the spherical boundaries, i.e. ![]() $\tilde {\boldsymbol {\mathcal {U}}}(\jmath _{\ell,p}/k,\theta,\varphi,t) =\boldsymbol {\mathcal {U}}(\jmath _{\ell,p}/k,\theta,\varphi,t)$.
$\tilde {\boldsymbol {\mathcal {U}}}(\jmath _{\ell,p}/k,\theta,\varphi,t) =\boldsymbol {\mathcal {U}}(\jmath _{\ell,p}/k,\theta,\varphi,t)$.
In this case, and regarding only the oscillating velocity, the vortex boundary is a second-order singular surface (the velocity field is continuous), and when it propagates, it is an acceleration wave.
When a momentum diffusivity ![]() $\nu \neq 0$ is considered in the Navier–Stokes equation (3.2), the oscillating motion in the interior and exterior domains decays according to (3.3a) but the effect in the vortex boundary may be problematic since the vortex boundary radius may change due to the lateral diffusivity (e.g. Kloosterziel Reference Kloosterziel1990).
$\nu \neq 0$ is considered in the Navier–Stokes equation (3.2), the oscillating motion in the interior and exterior domains decays according to (3.3a) but the effect in the vortex boundary may be problematic since the vortex boundary radius may change due to the lateral diffusivity (e.g. Kloosterziel Reference Kloosterziel1990).
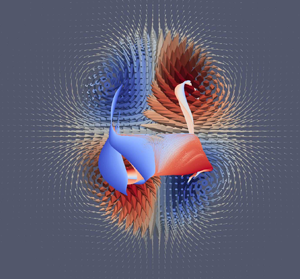
As an example, the velocity field and streamlines of a piecewise vortex are shown in figures 1 and 2. This example is the vortex ![]() $\boldsymbol {u}(\boldsymbol {x},t)$ with parameters
$\boldsymbol {u}(\boldsymbol {x},t)$ with parameters ![]() $\ell =2$,
$\ell =2$, ![]() $m=-2$,
$m=-2$, ![]() $\mathfrak {w}=0$,
$\mathfrak {w}=0$, ![]() $k=1$ and vorticity boundary at
$k=1$ and vorticity boundary at ![]() $k r_b = \jmath _{2,1}$. The streamlines set shown in these figures bifurcates twice. The first bifurcation occurs on
$k r_b = \jmath _{2,1}$. The streamlines set shown in these figures bifurcates twice. The first bifurcation occurs on ![]() $z>0$ on two descending streamline branches, the left and right branches (mostly in blue and red colours, respectively), which descend and cross the plane
$z>0$ on two descending streamline branches, the left and right branches (mostly in blue and red colours, respectively), which descend and cross the plane ![]() $z=0$ close to the regions of minimum axial speed (blue arrows in the north-west and south-east poles). The right branch (mostly in red) bifurcates again on
$z=0$ close to the regions of minimum axial speed (blue arrows in the north-west and south-east poles). The right branch (mostly in red) bifurcates again on ![]() $z<0$ on two ascending branches (see figure 2); the first branch (red) comes back to the initial location at
$z<0$ on two ascending branches (see figure 2); the first branch (red) comes back to the initial location at ![]() $z=0$ (south-west pole), while the second branch (red-blue) crosses the plane at the location of maximum axial speed in the north-east pole. Obviously, as it happens with similar piecewise multipolar vortices in two-dimensional flows for azimuthal wavenumbers larger than
$z=0$ (south-west pole), while the second branch (red-blue) crosses the plane at the location of maximum axial speed in the north-east pole. Obviously, as it happens with similar piecewise multipolar vortices in two-dimensional flows for azimuthal wavenumbers larger than ![]() $1$, this flow structure is not steady and breaks into four spherical dipoles, moving apart.
$1$, this flow structure is not steady and breaks into four spherical dipoles, moving apart.

Figure 1. Top view of the piecewise velocity field ![]() $\boldsymbol {u}(\boldsymbol {x},t_0)$ (arrows) at
$\boldsymbol {u}(\boldsymbol {x},t_0)$ (arrows) at ![]() $z=0$ and
$z=0$ and ![]() $t_0=0$ for parameters
$t_0=0$ for parameters ![]() $\ell =2$,
$\ell =2$, ![]() $m=-2$,
$m=-2$, ![]() $\mathfrak {w}=0$,
$\mathfrak {w}=0$, ![]() $k=1$ and vorticity boundary at
$k=1$ and vorticity boundary at ![]() $k r_b = \jmath _{2,1}$. Colour in the arrows corresponds to the axial
$k r_b = \jmath _{2,1}$. Colour in the arrows corresponds to the axial ![]() $z$-component of the velocity
$z$-component of the velocity ![]() $\boldsymbol {u}\boldsymbol {\cdot }\hat {\boldsymbol {z}}$ (blue and red colours mean negative and positive
$\boldsymbol {u}\boldsymbol {\cdot }\hat {\boldsymbol {z}}$ (blue and red colours mean negative and positive ![]() $z$-components, respectively). The coloured ribbon is a set of streamlines initiated on the plane
$z$-components, respectively). The coloured ribbon is a set of streamlines initiated on the plane ![]() $z=0$ on the region of maximum positive axial velocity (red arrows) in the south-west pole (the initial location of the streamline ribbon is shown more clearly in a side view in figure 2).
$z=0$ on the region of maximum positive axial velocity (red arrows) in the south-west pole (the initial location of the streamline ribbon is shown more clearly in a side view in figure 2).

Figure 2. Side (from the south) view of the streamlines ribbon shown in figure 1. The initial location of the streamlines ribbon is seen on the left-hand side.
11. Concluding remarks
In this paper, we have provided a new family of exact solutions of multipolar vortical flows ![]() $\boldsymbol {u}(\boldsymbol {x},t)$ satisfying the time-dependent vorticity equation. These solutions may be interpreted as time and space oscillations, with spherical geometry, embedded in a cylindrical constant flow with swirl, and are characterized as inertial oscillations in background flow. These time-dependent azimuthal oscillating velocity solutions are a generalization of the steady three-dimensional multipolar vortex solutions given in Viúdez (Reference Viúdez2022), which are recovered in the case of vanishing time dependence (
$\boldsymbol {u}(\boldsymbol {x},t)$ satisfying the time-dependent vorticity equation. These solutions may be interpreted as time and space oscillations, with spherical geometry, embedded in a cylindrical constant flow with swirl, and are characterized as inertial oscillations in background flow. These time-dependent azimuthal oscillating velocity solutions are a generalization of the steady three-dimensional multipolar vortex solutions given in Viúdez (Reference Viúdez2022), which are recovered in the case of vanishing time dependence (![]() $m\mathfrak{w}=0$). The necessary and sufficient condition for the existence of the inertial waves is a double condition: the flows experience inertial waves as long as they have a non-vanishing azimuthal wavenumber
$m\mathfrak{w}=0$). The necessary and sufficient condition for the existence of the inertial waves is a double condition: the flows experience inertial waves as long as they have a non-vanishing azimuthal wavenumber ![]() $(m \ne 0)$ in the presence of a background rotation (angular speed
$(m \ne 0)$ in the presence of a background rotation (angular speed ![]() $\mathfrak {w}\ne 0$). In the case of vanishing azimuthal wavenumber
$\mathfrak {w}\ne 0$). In the case of vanishing azimuthal wavenumber ![]() $m=0$, the background rotation causes no effect in the local flow, which becomes a steady solution
$m=0$, the background rotation causes no effect in the local flow, which becomes a steady solution ![]() $\boldsymbol {\mathcal {U}}(\boldsymbol {x})$ even in the presence of background rotational flow. In this case, however, small perturbations to the local flow would cause inertial oscillations. The vortex solutions also satisfy a superposable property, which is relevant to investigate the stability of simple, not composed, vortices. Furthermore, the more general time-dependent flow solution
$\boldsymbol {\mathcal {U}}(\boldsymbol {x})$ even in the presence of background rotational flow. In this case, however, small perturbations to the local flow would cause inertial oscillations. The vortex solutions also satisfy a superposable property, which is relevant to investigate the stability of simple, not composed, vortices. Furthermore, the more general time-dependent flow solution ![]() $\boldsymbol {u}(\boldsymbol {x},t)\,{\rm exp}(-\nu k^2 t)$ is a solution of the Navier–Stokes equation with constant diffusivity
$\boldsymbol {u}(\boldsymbol {x},t)\,{\rm exp}(-\nu k^2 t)$ is a solution of the Navier–Stokes equation with constant diffusivity ![]() $\nu$. Since the curl of the Lamb vector of the total flow
$\nu$. Since the curl of the Lamb vector of the total flow ![]() $\boldsymbol {\nabla } \times (\boldsymbol {\omega }\times \boldsymbol {u})$ is proportional to the oscillating term
$\boldsymbol {\nabla } \times (\boldsymbol {\omega }\times \boldsymbol {u})$ is proportional to the oscillating term ![]() $\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$, these nonlinear solutions also satisfy several well-known linear wave equations. An important problem to address now concerns the stability of these time-dependent solutions. Though piecewise vortices of individual modes seem to be unstable, it might be possible to find stable solutions in terms of a superposition of time-dependent modes, as has been done already with neutral vortices (vortices with vanishing amount of vertical vorticity) in two-dimensional Euler flows. This research is left for future work.
$\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$, these nonlinear solutions also satisfy several well-known linear wave equations. An important problem to address now concerns the stability of these time-dependent solutions. Though piecewise vortices of individual modes seem to be unstable, it might be possible to find stable solutions in terms of a superposition of time-dependent modes, as has been done already with neutral vortices (vortices with vanishing amount of vertical vorticity) in two-dimensional Euler flows. This research is left for future work.
Acknowledgements
I thank three anonymous reviewers for their comments on the manuscript.
Funding
Partial support for this study was obtained through project RT12018-100844-B-C33 (Spanish Ministry of Science, Innovation and Universities). I acknowledge the ‘Severo Ochoa Centre of Excellence’ accreditation (CEX2019-000928-S).
Declaration of interests
The author reports no conflict of interest.
Appendix A. The curl of the Lamb vector
Noticing that
the curl of the Lamb vector is
Applying the identity
to (A2) yields
Using the cylindrical components and cylindrical coordinates, we express the oscillating function ![]() $\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$ as
$\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$ as
Using the identity
where ![]() $\boldsymbol {A}=A_{\rho }\hat {\boldsymbol {\rho }}+A_{\varphi }\hat {\boldsymbol {\varphi }}+A_{z}\hat {\boldsymbol {z}}$, the term in (A4) simplifies to
$\boldsymbol {A}=A_{\rho }\hat {\boldsymbol {\rho }}+A_{\varphi }\hat {\boldsymbol {\varphi }}+A_{z}\hat {\boldsymbol {z}}$, the term in (A4) simplifies to
Relation (A6) is satisfied also using spherical components in spherical coordinates, and is the expression of the covariant derivative ![]() $\boldsymbol {\nabla }_{\boldsymbol {V}} \boldsymbol {U}$ of a vector field
$\boldsymbol {\nabla }_{\boldsymbol {V}} \boldsymbol {U}$ of a vector field ![]() $\boldsymbol {U}$ with respect to another vector field
$\boldsymbol {U}$ with respect to another vector field ![]() $\boldsymbol {V}$:
$\boldsymbol {V}$:
where ![]() $\varGamma ^k_{ij} = - (\partial \boldsymbol {e}^k/\partial x^i)\boldsymbol {\cdot }\boldsymbol {e}_j$ are the Christoffel symbols, in the particular case where
$\varGamma ^k_{ij} = - (\partial \boldsymbol {e}^k/\partial x^i)\boldsymbol {\cdot }\boldsymbol {e}_j$ are the Christoffel symbols, in the particular case where ![]() $\boldsymbol {U}=\boldsymbol {\mathcal {U}}$ and
$\boldsymbol {U}=\boldsymbol {\mathcal {U}}$ and ![]() $\boldsymbol {V}=\rho \hat {\boldsymbol {\varphi }}$.
$\boldsymbol {V}=\rho \hat {\boldsymbol {\varphi }}$.
Therefore, the curl of the Lamb vector (A4) is a rotation by an azimuthal angle increment ![]() $\Delta \varphi ={\rm \pi} /(2m)$ of
$\Delta \varphi ={\rm \pi} /(2m)$ of ![]() $-\boldsymbol {\mathcal {U}}$ times
$-\boldsymbol {\mathcal {U}}$ times ![]() $m\mathfrak {w}k$, that is,
$m\mathfrak {w}k$, that is,
Furthermore, since
every component ![]() $\mathcal {U}_{i}(\rho,\varphi,z,t)$ of
$\mathcal {U}_{i}(\rho,\varphi,z,t)$ of ![]() $\boldsymbol {\mathcal {U}}_{c}(\rho,\varphi,z,t)$ in (A5) satisfies
$\boldsymbol {\mathcal {U}}_{c}(\rho,\varphi,z,t)$ in (A5) satisfies
and in particular the kinetic energy density ![]() $\mathcal {E}(\boldsymbol {x},t)$ of the oscillating motion,
$\mathcal {E}(\boldsymbol {x},t)$ of the oscillating motion,
satisfies
which implies that in a normal plane ![]() $z=z_0$, the velocity of displacement of the kinetic energy density of the oscillating motion is the azimuthal velocity of the rigid motion
$z=z_0$, the velocity of displacement of the kinetic energy density of the oscillating motion is the azimuthal velocity of the rigid motion ![]() $\mathfrak {w}\rho \hat {\boldsymbol {\varphi }}$. The enstrophy density
$\mathfrak {w}\rho \hat {\boldsymbol {\varphi }}$. The enstrophy density ![]() $\mathcal {S}(\boldsymbol {x},t)$ of the oscillating motion is
$\mathcal {S}(\boldsymbol {x},t)$ of the oscillating motion is
and therefore satisfies the same conservation equation as the kinetic energy density ![]() $\mathcal {E}$ (A13).
$\mathcal {E}$ (A13).
Appendix B. Schrödinger and Klein–Gordon equations
The time-dependent non-relativistic Schrödinger equation for a massive free particle (i.e. without potential energy term) for the wave function ![]() $\varPsi (\boldsymbol {x},t)$ is commonly written as
$\varPsi (\boldsymbol {x},t)$ is commonly written as
where ![]() ${\mathrm{m}}$ is the rest mass of the particle in the quantum mechanics description, and
${\mathrm{m}}$ is the rest mass of the particle in the quantum mechanics description, and ![]() $\hbar$ is the reduced Planck constant. Thus, from (6.2a), assuming the equivalence
$\hbar$ is the reduced Planck constant. Thus, from (6.2a), assuming the equivalence
the oscillating function ![]() $\boldsymbol {\mathcal {U}}$, and all its curls, satisfy (B1).
$\boldsymbol {\mathcal {U}}$, and all its curls, satisfy (B1).
There is a relation between the physical application of the wave function ![]() $\varPsi (\boldsymbol {x},t)$ and the oscillating fields
$\varPsi (\boldsymbol {x},t)$ and the oscillating fields ![]() $\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$ appearing in piecewise vortices (§ 10) having a finite amount of volume. In these cases, and in particular when interactions between finite-size vortices take place (for example, dipole–dipole or dipole–vortex interactions), instead of the position or velocity, etc. (say
$\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$ appearing in piecewise vortices (§ 10) having a finite amount of volume. In these cases, and in particular when interactions between finite-size vortices take place (for example, dipole–dipole or dipole–vortex interactions), instead of the position or velocity, etc. (say ![]() $f(\boldsymbol {x},t)$) of the fluid particles, one is often interested in the spatial mean position, spatial mean velocity, etc. (say
$f(\boldsymbol {x},t)$) of the fluid particles, one is often interested in the spatial mean position, spatial mean velocity, etc. (say ![]() $\bar {f}(t)$) of the travelling or rotating vortices, which are usually defined as
$\bar {f}(t)$) of the travelling or rotating vortices, which are usually defined as
In (B3), the vorticity field ![]() $\boldsymbol {\mathcal {W}}(\boldsymbol {x},t)$ is used as a weight function because the exterior flow is potential in the piecewise vortices, but any of its successive curls would serve as well since
$\boldsymbol {\mathcal {W}}(\boldsymbol {x},t)$ is used as a weight function because the exterior flow is potential in the piecewise vortices, but any of its successive curls would serve as well since ![]() $\boldsymbol {\nabla }\times \boldsymbol {\mathcal {W}}=-k \boldsymbol {\mathcal {W}}$. Now, in the quantum mechanics description of particles, expressions similar to (B3) are used to define the expectation position, momentum, etc. of a quantum particle, but with the wave function
$\boldsymbol {\nabla }\times \boldsymbol {\mathcal {W}}=-k \boldsymbol {\mathcal {W}}$. Now, in the quantum mechanics description of particles, expressions similar to (B3) are used to define the expectation position, momentum, etc. of a quantum particle, but with the wave function ![]() $\varPsi (\boldsymbol {x},t)$ replacing the vorticity field
$\varPsi (\boldsymbol {x},t)$ replacing the vorticity field ![]() $\boldsymbol {\mathcal {W}}(\boldsymbol {x},t)$. However, lacking the fluid mechanics theory leading to the vorticity equation (1.1), the physical meaning of the wave function
$\boldsymbol {\mathcal {W}}(\boldsymbol {x},t)$. However, lacking the fluid mechanics theory leading to the vorticity equation (1.1), the physical meaning of the wave function ![]() $\varPsi (\boldsymbol {x},t)$ remains ambiguous. Indeed, such a meaning is not needed in the application of the mathematical theory as long as wave equations for
$\varPsi (\boldsymbol {x},t)$ remains ambiguous. Indeed, such a meaning is not needed in the application of the mathematical theory as long as wave equations for ![]() $\varPsi (\boldsymbol {x},t)$, such as the Schrödinger or Klein–Gordon wave equations, are postulated for the different classes of quantum particles.
$\varPsi (\boldsymbol {x},t)$, such as the Schrödinger or Klein–Gordon wave equations, are postulated for the different classes of quantum particles.
The oscillating field ![]() $\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$, and all its curls, also satisfy the relativistic Klein–Gordon equation
$\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$, and all its curls, also satisfy the relativistic Klein–Gordon equation
where ![]() ${\mathrm{c}}$ is the speed of light in vacuum. In particular, the oscillating field
${\mathrm{c}}$ is the speed of light in vacuum. In particular, the oscillating field ![]() $\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$ satisfies (B4) as long as the following equivalence relation holds:
$\boldsymbol {\mathcal {U}}(\boldsymbol {x},t)$ satisfies (B4) as long as the following equivalence relation holds:
This is the energy–momentum relation
with the following definitions for energy ![]() ${\mathrm{E}}$ and momentum of magnitude
${\mathrm{E}}$ and momentum of magnitude ![]() ${\mathrm{p}}$:
${\mathrm{p}}$:
in terms of the vortex free parameters ![]() $m$,
$m$, ![]() $\mathfrak {w}$ and
$\mathfrak {w}$ and ![]() $k$. Using the definition of the local frequency
$k$. Using the definition of the local frequency ![]() $\omega _l \equiv m \mathfrak {w}$ in (2.20), the energy (B7a) becomes the Planck–Einstein relation
$\omega _l \equiv m \mathfrak {w}$ in (2.20), the energy (B7a) becomes the Planck–Einstein relation ![]() ${\mathrm{E}}=\hbar \omega _l$, and defining the radial wavelength
${\mathrm{E}}=\hbar \omega _l$, and defining the radial wavelength ![]() $\lambda \equiv 2{\rm \pi} /k$, (B7b) becomes the de Broglie relation
$\lambda \equiv 2{\rm \pi} /k$, (B7b) becomes the de Broglie relation ![]() ${\mathrm{p}} \equiv 2{\rm \pi}\hbar/\lambda$, now defined in terms of the vortex free parameters
${\mathrm{p}} \equiv 2{\rm \pi}\hbar/\lambda$, now defined in terms of the vortex free parameters ![]() $\omega _l$ and
$\omega _l$ and ![]() $\lambda$.
$\lambda$.
The different sets of vortices defined by (7.6) and (B2) may be associated with the properties of scale invariance of the Euler flow equation that, for the particular solutions given here, imply that if ![]() $\boldsymbol {u}_{1}(\boldsymbol {x},t)$ is a flow solution with free parameters
$\boldsymbol {u}_{1}(\boldsymbol {x},t)$ is a flow solution with free parameters ![]() $u_{1,1},k_1,\mathfrak {w}_1$, then
$u_{1,1},k_1,\mathfrak {w}_1$, then ![]() $\boldsymbol {u}_{2}(\boldsymbol {x},t) \equiv s^h \boldsymbol {u}_{1}(s \boldsymbol {x},s^{1-h} t)$, with
$\boldsymbol {u}_{2}(\boldsymbol {x},t) \equiv s^h \boldsymbol {u}_{1}(s \boldsymbol {x},s^{1-h} t)$, with ![]() $s, h \in \mathbb {R}$ and parameters
$s, h \in \mathbb {R}$ and parameters
is a solution as well. In the particular case ![]() $h=-1$, the set comprises vortices satisfying the relations
$h=-1$, the set comprises vortices satisfying the relations
which, with the additional constraint of ![]() $m$-invariance (
$m$-invariance (![]() $m_2=m_1$), implies (B2).
$m_2=m_1$), implies (B2).
Case ![]() $h=0$ corresponds to those vortices having the same oscillating amplitude term
$h=0$ corresponds to those vortices having the same oscillating amplitude term ![]() $u_1$. In this case, we have
$u_1$. In this case, we have ![]() $u_{1,1}=u_{1,2}$,
$u_{1,1}=u_{1,2}$, ![]() $k_2 = s k_1$,
$k_2 = s k_1$, ![]() $\mathfrak {w}_2 = s \mathfrak {w}_1$, and therefore
$\mathfrak {w}_2 = s \mathfrak {w}_1$, and therefore
which, with the additional constraint of ![]() $m$-invariance (
$m$-invariance (![]() $m_2=m_1$), implies (7.6).
$m_2=m_1$), implies (7.6).