Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Shtern, Vladimir N.
2013.
Asymptotic flow in the depth of narrow cavity.
Physics of Fluids,
Vol. 25,
Issue. 8,
Herrada, Miguel
and
Shtern, Vladimir
2014.
Patterns of a creeping water-spout flow.
Journal of Fluid Mechanics,
Vol. 744,
Issue. ,
p.
65.
Shtern, Vladimir
2014.
Moffatt eddies at an interface.
Theoretical and Computational Fluid Dynamics,
Vol. 28,
Issue. 6,
p.
651.
Balci, Adnan
Brøns, Morten
Herrada, Miguel A
and
Shtern, Vladimir N
2015.
Vortex breakdown in a truncated conical bioreactor.
Fluid Dynamics Research,
Vol. 47,
Issue. 6,
p.
065503.
Davoust, Laurent
Achard, Jean-Luc
and
Drazek, Laurent
2015.
Low-to-moderate Reynolds number swirling flow in an annular channel with a rotating end wall.
Physical Review E,
Vol. 91,
Issue. 2,
Balci, Adnan
Brøns, Morten
Herrada, Miguel A.
and
Shtern, Vladimir N.
2016.
Patterns of a slow air–water flow in a semispherical container.
European Journal of Mechanics - B/Fluids,
Vol. 58,
Issue. ,
p.
1.
Naumov, Igor V.
Sharifullin, Bulat R.
Kravtsova, Aleksandra Yu.
and
Shtern, Vladimir N.
2020.
Velocity jumps and the Moffatt eddy in two-fluid swirling flows.
Experimental Thermal and Fluid Science,
Vol. 116,
Issue. ,
p.
110116.
Sharifullin, B R
Naumov, I V
Kravtsova, A Yu
and
Shtern, V N
2020.
Jump of azimuthal velocity in a creeping two-fluid swirling flow.
Journal of Physics: Conference Series,
Vol. 1677,
Issue. 1,
p.
012077.
Kolodezhnov, V.N.
2021.
Plane vortex flow in a cylindrical layer.
Computational Continuum Mechanics,
Vol. 14,
Issue. 2,
p.
159.
Kirkinis, Eleftherios
Mason, Joanne
and
Olvera de la Cruz, Monica
2022.
Odd-viscosity-induced passivation of Moffatt vortices.
Journal of Fluid Mechanics,
Vol. 950,
Issue. ,
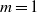 .
.