Article contents
Hydrodynamic diffusion of sedimenting point particles in a vertical shear flow
Published online by Cambridge University Press: 07 August 2013
Abstract
The hydrodynamic diffusion of sedimenting point particles in a vertically sheared periodic system is investigated numerically and theoretically. In both the velocity-gradient direction and the vorticity direction, the rate of hydrodynamic diffusion is reduced as the shear rate is increased. In the velocity-gradient direction, two-particle interactions cause no net displacement, and three-particle interactions are necessary for diffusive behaviour. In contrast to an unsheared system, the resulting diffusion coefficient is only weakly dependent upon the size of the system and 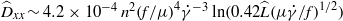 ${\widehat{D}}_{xx} \sim 4. 2\times 1{0}^{- 4} \hspace{0.167em} {n}^{2} {(f/ \mu )}^{4} {\dot {\gamma } }^{- 3} \ln (0. 42\widehat{L}{(\mu \dot {\gamma } / f)}^{1/ 2} )$ , where
${\widehat{D}}_{xx} \sim 4. 2\times 1{0}^{- 4} \hspace{0.167em} {n}^{2} {(f/ \mu )}^{4} {\dot {\gamma } }^{- 3} \ln (0. 42\widehat{L}{(\mu \dot {\gamma } / f)}^{1/ 2} )$ , where  $n$ is the particle number density,
$n$ is the particle number density,  $f$ the force per particle,
$f$ the force per particle, 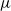 $\mu $ the fluid viscosity,
$\mu $ the fluid viscosity, 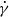 $\dot {\gamma } $ the imposed shear rate, and
$\dot {\gamma } $ the imposed shear rate, and 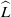 $\widehat{L}$ the system size. In the vorticity direction, although individual two-particle interactions cause no net displacement, a superposition of interactions is sufficient to cause diffusion-like linear growth of the ensemble-averaged square particle displacements. The associated diffusion coefficient is given by
$\widehat{L}$ the system size. In the vorticity direction, although individual two-particle interactions cause no net displacement, a superposition of interactions is sufficient to cause diffusion-like linear growth of the ensemble-averaged square particle displacements. The associated diffusion coefficient is given by 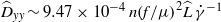 ${\widehat{D}}_{yy} \sim 9. 47\times 1{0}^{- 4} \hspace{0.167em} n{(f/ \mu )}^{2} \widehat{L}\hspace{0.167em} {\dot {\gamma } }^{- 1} $ . At sufficiently long times, the effect of multi-particle interactions cannot be neglected and there is a transition to another regime in which the diffusion coefficient is similar in form, but slightly reduced from this value. The dependence of
${\widehat{D}}_{yy} \sim 9. 47\times 1{0}^{- 4} \hspace{0.167em} n{(f/ \mu )}^{2} \widehat{L}\hspace{0.167em} {\dot {\gamma } }^{- 1} $ . At sufficiently long times, the effect of multi-particle interactions cannot be neglected and there is a transition to another regime in which the diffusion coefficient is similar in form, but slightly reduced from this value. The dependence of  ${\widehat{D}}_{xx} $ and
${\widehat{D}}_{xx} $ and  ${\widehat{D}}_{yy} $ on the number density and dimensionless shear rate is explained using theoretical scaling arguments and analyses.
${\widehat{D}}_{yy} $ on the number density and dimensionless shear rate is explained using theoretical scaling arguments and analyses.
JFM classification
Information
- Type
- Papers
- Information
- Copyright
- ©2013 Cambridge University Press
References
- 1
- Cited by

