Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Li, Larry K. B.
and
Juniper, Matthew P.
2013.
Phase trapping and slipping in a forced hydrodynamically self-excited jet.
Journal of Fluid Mechanics,
Vol. 735,
Issue. ,
Kashinath, Karthik
Waugh, Iain C.
and
Juniper, Matthew P.
2014.
Nonlinear self-excited thermoacoustic oscillations of a ducted premixed flame: bifurcations and routes to chaos.
Journal of Fluid Mechanics,
Vol. 761,
Issue. ,
p.
399.
Balusamy, Saravanan
Li, Larry K.B.
Han, Zhiyi
Juniper, Matthew P.
and
Hochgreb, Simone
2015.
Nonlinear dynamics of a self-excited thermoacoustic system subjected to acoustic forcing.
Proceedings of the Combustion Institute,
Vol. 35,
Issue. 3,
p.
3229.
Camarri, Simone
2015.
Flow control design inspired by linear stability analysis.
Acta Mechanica,
Vol. 226,
Issue. 4,
p.
979.
Ahmed, Anwar
and
Weiner, Arthur J.
2016.
Axisymmetric Jet Subjected to Radial and Azimuthal Forcing.
Journal of Propulsion and Power,
Vol. 32,
Issue. 2,
p.
311.
Zhu, Yuanhang
Gupta, Vikrant
and
Li, Larry K. B.
2017.
Onset of global instability in low-density jets.
Journal of Fluid Mechanics,
Vol. 828,
Issue. ,
Zhou, Hao
Huang, Yan
and
Meng, Sheng
2017.
Response of non-premixed swirl-stabilized flames to acoustic excitation and jet in cross-flow perturbations.
Experimental Thermal and Fluid Science,
Vol. 82,
Issue. ,
p.
124.
Hyodo, Hiroaki
and
Biwa, Tetsushi
2018.
Phase-Locking and Suppression States Observed in Forced Synchronization of Thermoacoustic Oscillator.
Journal of the Physical Society of Japan,
Vol. 87,
Issue. 3,
p.
034402.
Qadri, Ubaid Ali
Chandler, Gary J.
and
Juniper, Matthew P.
2018.
Passive control of global instability in low-density jets.
European Journal of Mechanics - B/Fluids,
Vol. 72,
Issue. ,
p.
311.
Brackston, R.D.
Wynn, A.
and
Morrison, J.F.
2018.
Modelling and feedback control of vortex shedding for drag reduction of a turbulent bluff body wake.
International Journal of Heat and Fluid Flow,
Vol. 71,
Issue. ,
p.
127.
Lückoff, Finn
Sieber, Moritz
Paschereit, Christian Oliver
and
Oberleithner, Kilian
2018.
Characterization of Different Actuator Designs for the Control of the Precessing Vortex Core in a Swirl-Stabilized Combustor.
Journal of Engineering for Gas Turbines and Power,
Vol. 140,
Issue. 4,
He, Wei
Yu, Peng
and
Li, Larry K.B.
2018.
Ground effects on the stability of separated flow around a NACA 4415 airfoil at low Reynolds numbers.
Aerospace Science and Technology,
Vol. 72,
Issue. ,
p.
63.
Yu, Peng
Lu, Ruixin
He, Wei
and
Li, Larry K.B.
2018.
Steady flow around an inclined torus at low Reynolds numbers: Lift and drag coefficients.
Computers & Fluids,
Vol. 171,
Issue. ,
p.
53.
Kashinath, Karthik
Li, Larry K. B.
and
Juniper, Matthew P.
2018.
Forced synchronization of periodic and aperiodic thermoacoustic oscillations: lock-in, bifurcations and open-loop control.
Journal of Fluid Mechanics,
Vol. 838,
Issue. ,
p.
690.
Pawar, Samadhan A.
Sujith, Raman I.
Emerson, Benjamin
and
Lieuwen, Tim
2018.
Characterization of forced response of density stratified reacting wake.
Chaos: An Interdisciplinary Journal of Nonlinear Science,
Vol. 28,
Issue. 2,
Guan, Yu
Liu, Peijin
Jin, Bingning
Gupta, Vikrant
and
Li, Larry K.B.
2018.
Nonlinear time-series analysis of thermoacoustic oscillations in a solid rocket motor.
Experimental Thermal and Fluid Science,
Vol. 98,
Issue. ,
p.
217.
Guan, Yu
Murugesan, Meenatchidevi
and
Li, Larry K. B.
2018.
Strange nonchaotic and chaotic attractors in a self-excited thermoacoustic oscillator subjected to external periodic forcing.
Chaos: An Interdisciplinary Journal of Nonlinear Science,
Vol. 28,
Issue. 9,
He, Wei
Pérez, José Miguel
Yu, Peng
and
Li, Larry K.B.
2019.
Non-modal stability analysis of low-Re separated flow around a NACA 4415 airfoil in ground effect.
Aerospace Science and Technology,
Vol. 92,
Issue. ,
p.
269.
Guan, Yu
He, Wei
Murugesan, Meenatchidevi
Li, Qiang
Liu, Peijin
and
Li, Larry K.B.
2019.
Control of self-excited thermoacoustic oscillations using transient forcing, hysteresis and mode switching.
Combustion and Flame,
Vol. 202,
Issue. ,
p.
262.
Guan, Yu
Gupta, Vikrant
Wan, Minping
and
Li, Larry K. B.
2019.
Forced synchronization of quasiperiodic oscillations in a thermoacoustic system.
Journal of Fluid Mechanics,
Vol. 879,
Issue. ,
p.
390.
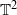 ${ \mathbb{T} }^{2} $ quasiperiodicity via a torus-birth bifurcation; and then (ii) from
${ \mathbb{T} }^{2} $ quasiperiodicity via a torus-birth bifurcation; and then (ii) from 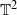 ${ \mathbb{T} }^{2} $ quasiperiodicity to 1:1 lock-in via either a saddle-node bifurcation with frequency pulling, if the forcing and natural frequencies are close together, or a torus-death bifurcation without frequency pulling, but with a gradual suppression of the natural mode, if the two frequencies are far apart. We also find that the jet locks in most readily when forced close to its natural frequency, but that the details contain two asymmetries: the jet (i) locks in more readily and (ii) oscillates more strongly when it is forced below its natural frequency than when it is forced above it. Except for the second asymmetry, all of these transitions, bifurcations and dynamics are accurately reproduced by the forced van der Pol oscillator. This shows that this complex (infinite-dimensional) forced self-excited jet can be modelled reasonably well as a simple (three-dimensional) forced self-excited oscillator. This result adds to the growing evidence that open self-excited flows behave essentially like low-dimensional nonlinear dynamical systems. It also strengthens the universality of such flows, raising the possibility that more of them, including some industrially relevant flames, can be similarly modelled.
${ \mathbb{T} }^{2} $ quasiperiodicity to 1:1 lock-in via either a saddle-node bifurcation with frequency pulling, if the forcing and natural frequencies are close together, or a torus-death bifurcation without frequency pulling, but with a gradual suppression of the natural mode, if the two frequencies are far apart. We also find that the jet locks in most readily when forced close to its natural frequency, but that the details contain two asymmetries: the jet (i) locks in more readily and (ii) oscillates more strongly when it is forced below its natural frequency than when it is forced above it. Except for the second asymmetry, all of these transitions, bifurcations and dynamics are accurately reproduced by the forced van der Pol oscillator. This shows that this complex (infinite-dimensional) forced self-excited jet can be modelled reasonably well as a simple (three-dimensional) forced self-excited oscillator. This result adds to the growing evidence that open self-excited flows behave essentially like low-dimensional nonlinear dynamical systems. It also strengthens the universality of such flows, raising the possibility that more of them, including some industrially relevant flames, can be similarly modelled.