Hostname: page-component-78c5997874-4rdpn Total loading time: 0 Render date: 2024-11-14T22:43:56.278Z Has data issue: false hasContentIssue false
  • English
  • Français

On the acoustic levitation stability behaviour of spherical and ellipsoidal particles

Published online by Cambridge University Press:  31 August 2012

D. Foresti ,
M. Nabavi  and
D. Poulikakos
D. Foresti
Affiliation:
Department of Mechanical and Process Engineering, Institute of Energy Technology, Laboratory of Thermodynamics in Emerging Technologies, ETH Zurich, CH-8092, Zurich, Switzerland
M. Nabavi
Affiliation:
Department of Mechanical and Process Engineering, Institute of Energy Technology, Laboratory of Thermodynamics in Emerging Technologies, ETH Zurich, CH-8092, Zurich, Switzerland
D. Poulikakos*
Affiliation:
Department of Mechanical and Process Engineering, Institute of Energy Technology, Laboratory of Thermodynamics in Emerging Technologies, ETH Zurich, CH-8092, Zurich, Switzerland
*
†Email address for correspondence: dpoulikakos@ethz.ch
Get access Rights & Permissions [Opens in a new window]

Abstract

We present here an in-depth analysis of particle levitation stability and the role of the radial and axial forces exerted on fixed spherical and ellipsoidal particles levitated in an axisymmetric acoustic levitator, over a wide range of particle sizes and surrounding medium viscosities. We show that the stability behaviour of a levitated particle in an axisymmetric levitator is unequivocally connected to the radial forces: the loss of levitation stability is always due to the change of the radial force sign from positive to negative. 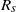It is found that the axial force exerted on a sphere of radius increases with increasing viscosity for 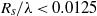( 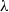is the acoustic wavelength), with the viscous contribution of this force scaling with the inverse of the sphere radius. The axial force decreases with increasing viscosity for spheres with 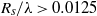. The radial force, on the other hand, decreases monotonically with increasing viscosity. The radial and axial forces exerted on an ellipsoidal particle are larger than those exerted on a volume-equivalent sphere, up to the point where the ellipsoid starts to act as an obstacle to the formation of the standing wave in the levitator chamber.

JFM classification

Drops and Bubbles: Drops Waves/Free-surface Flows: Wave scattering
Type
Papers
Information
Journal of Fluid Mechanics , Volume 709 , 25 October 2012 , pp. 581 - 592
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Andrade, M. A. B., Buiochi, F. & Adamowski, J. C. 2010 Finite element analysis and optimization of a single-axis acoustic levitator. IEEE Trans. Ultrason. Ferroelectr. 57, 469–479.CrossRefGoogle ScholarPubMed
2. Annamalai, P., Trinh, E. & Wang, T. G. 1985 Experimental study of the oscillations of a rotating drop. J. Fluid Mech. 158, 317–327.CrossRefGoogle Scholar
3. Barmatz, M. & Collas, P. 1985 Acoustic radiation potential on a sphere in plane, cylindrical, and spherical standing wave fields. J. Acoust. Soc. Am. 77, 928–945.CrossRefGoogle Scholar
4. Barrios, G. & Rechtman, R. 2008 Dynamics of an acoustically levitated particle using the lattice Boltzmann method. J. Fluid Mech. 596, 191–200.CrossRefGoogle Scholar
5. Bjelobrk, N., Foresti, D., Dorrestijn, M., Nabavi, M. & Poulikakos, D. 2010 Contactless transport of acoustically levitated particles. Appl. Phys. Lett. 97, 161904.CrossRefGoogle Scholar
6. Bruus, H., Dual, J., Hawkes, J., Hill, M., Laurell, T., Nilsson, J., Radel, S., Sadhal, S. & Wiklund, M. 2011 Forthcoming lab on a chip tutorial series on acoustofluidics: Acoustofluidics-exploiting ultrasonic standing wave forces and acoustic streaming in microfluidic systems for cell and particle manipulation lab chip. Lab on a Chip 11, 3579–3580.CrossRefGoogle Scholar
7. Busse, F. H. 1984 Oscillations of a rotating liquid-drop. J. Fluid Mech. 142, 1–8.CrossRefGoogle Scholar
8. Danilov, S. D. & Mironov, M. A. 2000 Mean force on a small sphere in a sound field in a viscous fluid. J. Acoust. Soc. Am. 107, 143–153.CrossRefGoogle Scholar
9. Doinikov, A. A. 1994 Acoustic radiation pressure on a compressible sphere in a viscous fluid. J. Fluid Mech. 267, 1–21.CrossRefGoogle Scholar
10. Doinikov, A. A. 1997 Acoustic radiation force on a spherical particle in a viscous heat-conducting fluid. Part 2. Force on a rigid sphere. J. Acoust. Soc. Am. 101, 722–730.CrossRefGoogle Scholar
11. Foresti, D., Bjelobrk, N., Nabavi, M. & Poulikakos, D. 2011 Investigation of a line-focused acoustic levitation for contactless transport of particles. J. Appl. Phys. 109, 093503.CrossRefGoogle Scholar
12. Foresti, D., Nabavi, M. & Poulikakos, D. 2012 Contactless transport of matter in the first five resonance modes of a line-focused acoustic manipulator. J. Acoust. Soc. Am. 131, 1029–1038.CrossRefGoogle ScholarPubMed
13. Haydock, D. 2005 Lattice boltzmann simulations of the time-averaged forces on a cylinder in a sound field. J. Phys. A: Math Gen. 38, 3265–3277.CrossRefGoogle Scholar
14. King, L. V. 1934 On the acoustic radiation pressure on spheres. Proc. R. Soc. Lond. A. 147, 212–240.Google Scholar
15. Koyama, D. & Nakamura, K. 2010 Noncontact ultrasonic transportation of small objects over long distances in air using a bending vibrator and a reflector. IEEE Trans. Ultrason. Ferroelectr. 57, 1152–1159.CrossRefGoogle Scholar
16. Kozuka, T., Yasui, K., Tuziuti, T., Towata, A. & Iida, Y. 2008 Acoustic standing-wave field for manipulation in air. Japan J. Appl. Phys. 47, 4336–4338.CrossRefGoogle Scholar
17. Lee, C. P., Anilkumar, A. V. & Wang, T. G. 1991 Static shape of an acoustically levitated drop with wave-drop interaction. Phys. Fluids A 3, 2497–2515.CrossRefGoogle Scholar
18. Lee, C. P. & Wang, T. G. 1990 Outer acoustic streaming. J. Acoust. Soc. Am. 88, 2367–2375.CrossRefGoogle Scholar
19. Lierke, E. G. 2002 Deformation and displacement of liquid drops in an optimized acoustic standing wave levitator. Acta Acust. United Ac. 88, 206–217.Google Scholar
20. Lierke, E. G. & Holitzner, L. 2008 Perspectives of an acoustic-electrostatic/electrodynamic hybrid levitator for small fluid and solid samples. Meas. Sci. Technol. 19, 115803.CrossRefGoogle Scholar
21. Rednikov, A. Y., Zhao, H., Sadhal, S. S. & Trinh, E. H. 2006 Steady streaming around a spherical drop displaced from the velocity antinode in an acoustic levitation field. Q. J. Mech. Appl. Maths 59, 377–397.CrossRefGoogle Scholar
22. Rudnick, J. & Barmatz, M. 1990 Oscillational instabilities in single-mode acoustic levitators. J. Acoust. Soc. Am. 87, 81–92.CrossRefGoogle Scholar
23. Trinh, E. H., Thiessen, D. B. & Holt, R. G. 1998 Driven and freely decaying nonlinear shape oscillations of drops and bubbles immersed in a liquid: experimental results. J. Fluid Mech. 364, 253–272.CrossRefGoogle Scholar
24. Vandaele, V. 2011 Contactless handling for micro-assembly: acoustic levitation, PhD thesis.Google Scholar
25. Vandaele, V., Delchambre, A. & Lambert, P. 2011 Acoustic wave levitation: handling of components. J. Appl. Phys. 109, 124901.CrossRefGoogle Scholar
26. Wang, J. T. & Dual, J. 2009 Numerical simulations for the time-averaged acoustic forces acting on rigid cylinders in ideal and viscous fluids. J. Phys. A – Math. Theor. 42.CrossRefGoogle Scholar
27. Xie, W. J. & Wei, B. 2001 Parametric study of single-axis acoustic levitation. Appl. Phys. Lett. 79, 881–883.CrossRefGoogle Scholar
28. Xie, W. J. & Wei, B. 2004 Dynamics of acoustically levitated disk samples. Phys. Rev. E 70, 046611.CrossRefGoogle ScholarPubMed
29. Yarin, A. L., Pfaffenlehner, M. & Tropea, C. 1998 On the acoustic levitation of droplet. J. Fluid Mech. 356, 65–91.CrossRefGoogle Scholar
30. Yarin, A. L., Weiss, D. A., Brenn, G. & Rensink, D. 2002 Acoustically levitated drops: drop oscillation and break-up driven by ultrasound modulation. Intl J. Multiphase Flow 28, 887–910.CrossRefGoogle Scholar
Supplementary material: PDF

Foresti et al. supplementary material

Appendices

Download Foresti et al. supplementary material(PDF)
PDF 2.1 MB