Published online by Cambridge University Press: 14 December 2022
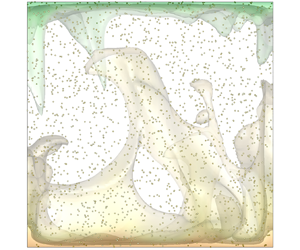
We investigate the energy transport and heat transfer efficiency in turbulent Rayleigh–Bénard (RB) convection laden with radiatively heated inertial particles. Direct numerical simulations combined with the Lagrangian point-particle mode were carried out in the range of density ratio  $854.7\le \rho _p/\rho _0 \le 8547$ and radiation intensity
$854.7\le \rho _p/\rho _0 \le 8547$ and radiation intensity  $1\le \phi /\phi _{solar}\le 100$ for both two-dimensional (2-D) and three-dimensional (3-D) simulations. The Rayleigh number ranges from
$1\le \phi /\phi _{solar}\le 100$ for both two-dimensional (2-D) and three-dimensional (3-D) simulations. The Rayleigh number ranges from  $2\times 10^6$ to
$2\times 10^6$ to  $10^8$ for 2-D cases, and is
$10^8$ for 2-D cases, and is  $10^7$ for 3-D cases for
$10^7$ for 3-D cases for  $Pr=0.71$. It is found that particles with small density ratio that encounter strong radiation significantly alter the flow momentum transport and fluid heat transfer, so the fluid temperature of bulk is remarkably heated. We then derived the theoretical relation of the Nusselt number for interphase heat transfer in the heated particle-laden RB convection, which reveals that the heat transfer difference between the top and bottom plates stems from the interphase heat transfer. We further found that both the interphase heat transfer and the interphase thermal energy transport exhibit universal properties. They are both increased linearly with the reciprocal of the normalized density ratio. Additionally, both the interphase heat transfer and the interphase thermal energy transport increase linearly with the increase of radiation intensity. The growth rates exhibit specific scaling relations versus Rayleigh number and density ratio. Two different regimes distinguished by the critical density ratio, i.e. the exothermic particle regime and the endothermic particle regime, are observed. We further derived the power-law relation of the critical density ratios versus Rayleigh number and radiation intensity, i.e.
$Pr=0.71$. It is found that particles with small density ratio that encounter strong radiation significantly alter the flow momentum transport and fluid heat transfer, so the fluid temperature of bulk is remarkably heated. We then derived the theoretical relation of the Nusselt number for interphase heat transfer in the heated particle-laden RB convection, which reveals that the heat transfer difference between the top and bottom plates stems from the interphase heat transfer. We further found that both the interphase heat transfer and the interphase thermal energy transport exhibit universal properties. They are both increased linearly with the reciprocal of the normalized density ratio. Additionally, both the interphase heat transfer and the interphase thermal energy transport increase linearly with the increase of radiation intensity. The growth rates exhibit specific scaling relations versus Rayleigh number and density ratio. Two different regimes distinguished by the critical density ratio, i.e. the exothermic particle regime and the endothermic particle regime, are observed. We further derived the power-law relation of the critical density ratios versus Rayleigh number and radiation intensity, i.e.  $\rho _p/\rho _c \sim (\phi /\phi _{solar})^{1/2}\,Ra^{1/3}$, which is in remarkable agreement with the 3-D simulations.
$\rho _p/\rho _c \sim (\phi /\phi _{solar})^{1/2}\,Ra^{1/3}$, which is in remarkable agreement with the 3-D simulations.