Article contents
Preferential frequency and size effect of the Brownian force acting on a nanoparticle
Published online by Cambridge University Press: 05 September 2017
Abstract
The Brownian motion of a nanoparticle in fluid depends on the molecular forces acting on it. Because of the small size and the high frequency, it is difficult to make experimental measurements of these forces. In the present work, Brownian forces acting on a nanoparticle are numerically investigated with the molecular dynamics method. Some new phenomena are disclosed. (i) The probability distribution shows that the Brownian forces conform to the Gaussian distribution and self-similarity of the probability distribution is also found for different  $1/Kn$ numbers which are characterized with the particle radius and the mean path
$1/Kn$ numbers which are characterized with the particle radius and the mean path  $\unicode[STIX]{x1D706}$ of the gas molecule
$\unicode[STIX]{x1D706}$ of the gas molecule 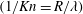 $(1/Kn=R/\unicode[STIX]{x1D706})$ . (ii) The frequency spectrum distribution of the Brownian force is not a white noise spectrum, which is different from the assumption commonly used in Langevin model. The preferential frequency of the Brownian force is found. (iii) The size effect relating to the Brownian forces is not monotonically varying with
$(1/Kn=R/\unicode[STIX]{x1D706})$ . (ii) The frequency spectrum distribution of the Brownian force is not a white noise spectrum, which is different from the assumption commonly used in Langevin model. The preferential frequency of the Brownian force is found. (iii) The size effect relating to the Brownian forces is not monotonically varying with  $1/Kn=R/\unicode[STIX]{x1D706}$ and is also found. It first increases and then decreases after it reaches the maximum value at
$1/Kn=R/\unicode[STIX]{x1D706}$ and is also found. It first increases and then decreases after it reaches the maximum value at 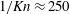 $1/Kn\approx 250$ . The variation process for
$1/Kn\approx 250$ . The variation process for 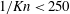 $1/Kn<250$ observed in the present work has not been reported in previous research to date.
$1/Kn<250$ observed in the present work has not been reported in previous research to date.
Information
- Type
- Papers
- Information
- Copyright
- © 2017 Cambridge University Press
References
- 1
- Cited by


